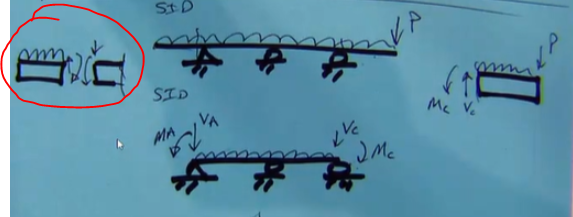
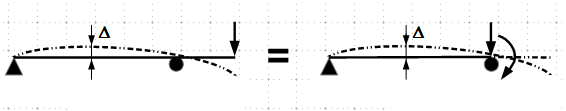I have been practising the superposition method lately and I am confused in terms of drawing the directions when sectioning. Here is the problem:

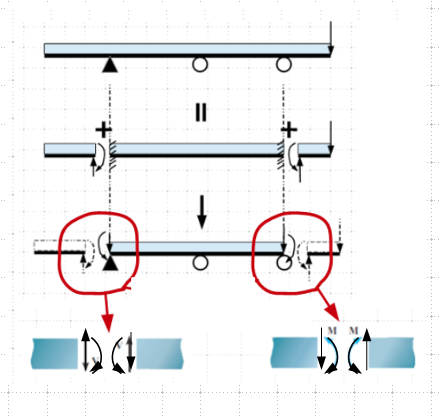
Heres is the process of converting a indeterminate system into a more solvable indeterminate system drawn by my lecturer.
Can someone please explain the direction of shear and moment drawn? For example, Why is it up and clockwise for the sectioning on the left beam? I understand that the opposite side of the beam must have opposite shear/moment.
According to my textbook:
 Shouldn't we assume down and anticlockwise for the left most beam, like this diagram suggests?
Shouldn't we assume down and anticlockwise for the left most beam, like this diagram suggests?