1
$\begingroup$

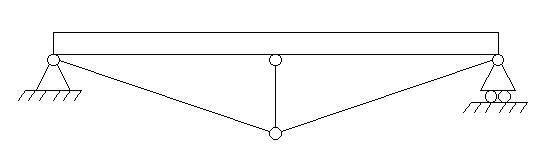
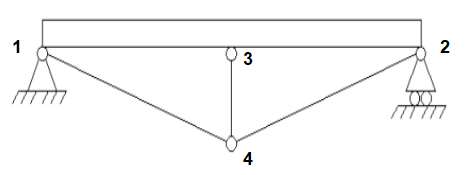
enter image description here

I was working earlier on an example problem of a trussed beam. Above you can see a picture illustrating the situation. On top there is a simply supported beam, with a stiffening truss below. The truss consists of 3 members, all connected through pins to each other, the supports, and the middle member pin connected to the beam. The beam has a uniform load on top.

I'm having a hard time calculating the degree of indeterminacy of this system. I'm told that this system is first degree indeterminate.

If we take the left support as point $A$ and right support as point $B$, we should have supporting reactions $A_x$, $A_y$ and $B_y$, as well as 3 normal loads for the truss members (since they only carry normal loads, no moments or shear). Using statics, we can form 3 equations: $\Sigma F_x$, $\Sigma F_y$ and $\Sigma M_z$. Because we have 6 unknowns, I would say this is 3rd degree indeterminate.

My reasoning is quite likely wrong, I'm having hard time for some reason understanding static determinacy beyond simple examples. Could somebody walk through the process of calculating the degree of indeterminacy for this structure in detail? Thank you very much!

Improve this question
$\endgroup$

3 Answers 3

Reset to default
2
$\begingroup$

enter image description here

Stability & Determinacy of Trusses, in general

enter image description here

In which,

  • number of unknowns = (2 forces/per member)*number of member + external reactions = $2m +r$

  • number of equations = (1 eq/member)*number of member + (2 eq/joint)*numberof joint = $m + 2j$

For this case:

number of unknowns = 2*4 + 3 = 11

number of equations = 4 + 2*4 = 12

The structure is unstable internally, and the reason is the continuity of member 1-2 at joint 3 that causes the joint to rotate, so we realize that member 1-2 is a flexural member than a truss member which can carry axial load only. With this realization, further inspection of member 1-2 as a flexural member (beam) reveals it is statically indeterminate to the first degree, as there are 4 unknowns (2 on jt 1, and 1 each at jts 2 & 3, note that jt 3 is a floating support that is capable of providing a vertical reaction only) with 3 available equations ($\sum M = 0$; $\sum F_X = 0$; $\sum F_Y = 0$). Thus, solution can be found using the "displacement compatibility method" and by releasing and reintroducing a unit force at the internal joint 3.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Why are there two unknown forces per member? Shouldn't a truss member only have one, the axial force? $\endgroup$
    – S. Rotos
    Commented Nov 9, 2021 at 19:03
  • $\begingroup$ Every book writes the stability/indeterminacy equations differently, but the conclusion should be the same. The reference source I used counted one member has two end forces. However, the author also provided another approach (in line with your objection): reactions = # of members + reactions & equations = 2*# of joints. So for this case, reactions = 4+3 = 7; equations = 2*4 = 8 , since equations > reactions ----> the structure is unstable internally. $\endgroup$
    – r13
    Commented Nov 9, 2021 at 19:26
  • $\begingroup$ Okay. But how about the beam? The beam carries shear and moment as well as normal load, doesn't that change things? $\endgroup$
    – S. Rotos
    Commented Nov 9, 2021 at 19:37
  • $\begingroup$ Yes, so the equilibrium equations are "sum horizontal forces (Fx) = 0"; "sum vertical forces (Fy) = 0"; and "sum moments (M) = 0". You compare the number of available equilibrium equations with the number of support reactions to determine the static determinacy. $\endgroup$
    – r13
    Commented Nov 9, 2021 at 19:43
  • $\begingroup$ For example - a fixed end beam has 6 unknowns but 3 equations, so i = 3; a propped beam (one end fixed, one end supported by a roller), it has 4 unknowns but 3 equations, so i = 1 - if the roller is released, or the rotational constrain is removed, the beam becomes statically determined. $\endgroup$
    – r13
    Commented Nov 9, 2021 at 19:53
1
$\begingroup$

Like you said, there are

  • 6 unknowns and
  • 3 equations can be formed for $\sum F_x,\sum F_y$ and $\sum M$ for the whole structure.

There are two additional equations $\sum F_x,\sum F_y$ that can be formed for the node that all 3 trusses converge (right at the bottom of the image).

So there are 6 unknowns and 5 equations, therefore there is one degree indeterminate.

if any of the truss rods are removed then the system could be solved (assuming that the horizontal beam is rigid).

Improve this answer
$\endgroup$
0
$\begingroup$

No of equation:

You have 3 equilibrium equations for the whole structure, plus 2 equations (Fx=0, Fy=0) if you consider equilibrium of the truss joint where the members meet.

i.e. Equations = 5

Number of Unknowns:

You have 3 unknown reactions, plus 3 unknowns, that is, one unknown for each truss member.

i.e. Unknowns = 6

Degree of Indeterminacy:

DI = Unknowns - Equations = 1

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.