0
$\begingroup$

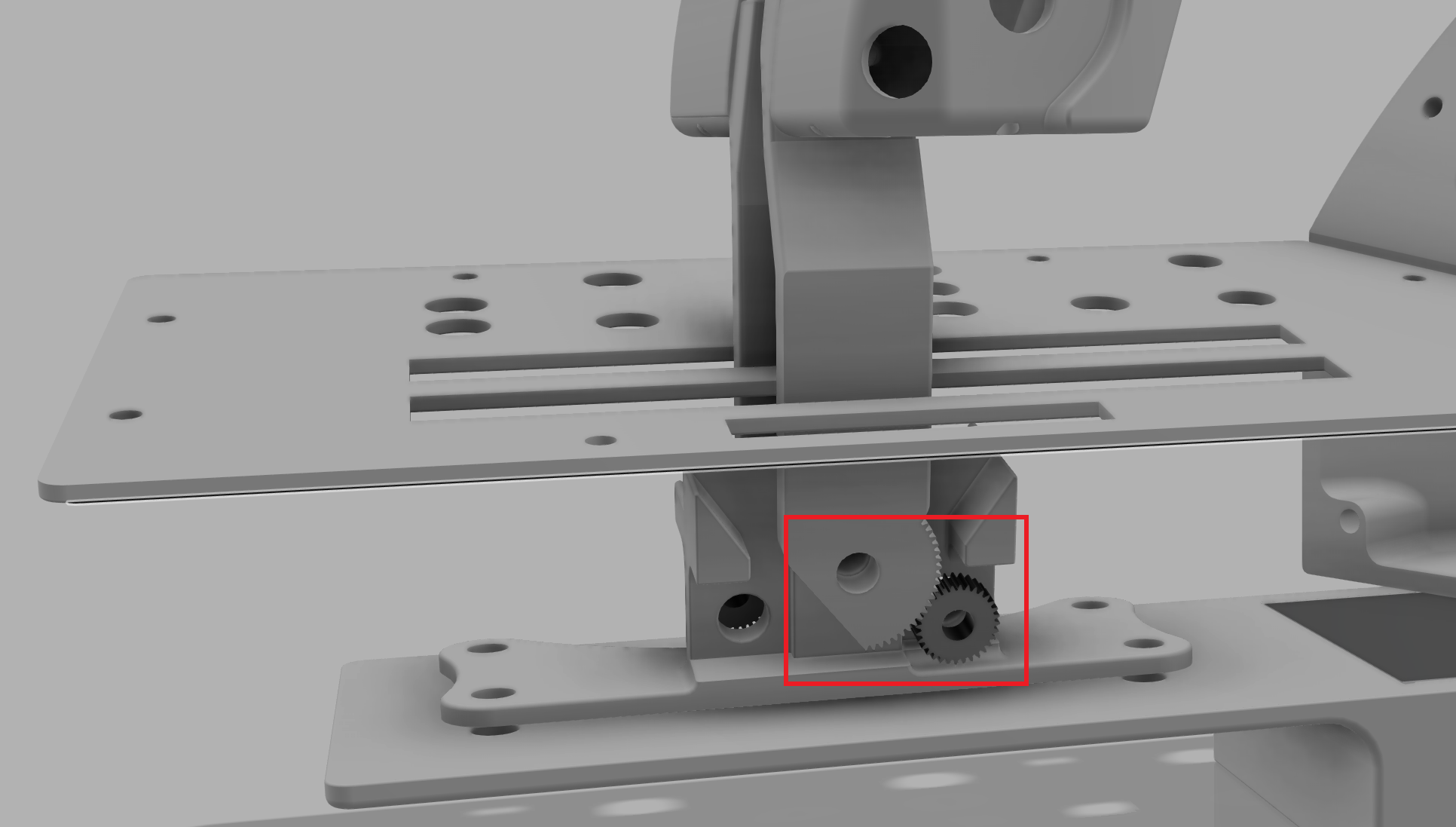
I'm 3D printing an aircraft throttle unit as a POC and am trying to calculate the gearing ratios that are required in order transfer the full range of linear motion into a directionally opposed sensor whilst being constrained by certain design factors.

In the attached image, each throttle unit (L&R) will have a total articulation of 70° (35° each way from centre) and I'm trying to work out how to transfer/translate that linear motion into a rotational force that'll drive input into a potentiometer with a rotational constraint of 295°

I've tried calculating the ratio myself using the following expression 'm=a/r' where 'm' is equal to the articulation of linear motion divided by rotational constraint to gives me 1:4.2 but I have a feeling that's incorrect.

Also, once I have the correct gear ratio I'm unsure of how to work out the number of teeth required on both the spur gear and drive gear (is it as simple as for each tooth on the drive gear, 4 (rounding down) are required on the spur gear?)

Thanks in advance!

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ There is no linear motion here. Both gears rotate about their center. There is no pinion gear here. You want the $\theta = 70°$ of articulation to translate to $n$ rotations of the input gear, so the ratio is $$ \gamma = \frac{n 360}{\theta}$$ $\endgroup$
    – John Alexiou
    Commented Oct 3, 2023 at 6:02

1 Answer 1

Reset to default
0
$\begingroup$

If you want to use gears, then you can end your power handle into an arc of 70 degrees, say 5 degrees more for play.

let's call the radius of this arc R1 and the 2nd one R2.

The other gear shoul have a radoius

$$ R2= 70R1/295$$

The number of the teeth on both gears have to be equal, on the handle they span 70 degrees and on the 2nd gear thy span 295 degrees.

Another possible alternative is to use rack and pinion.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.