0
$\begingroup$

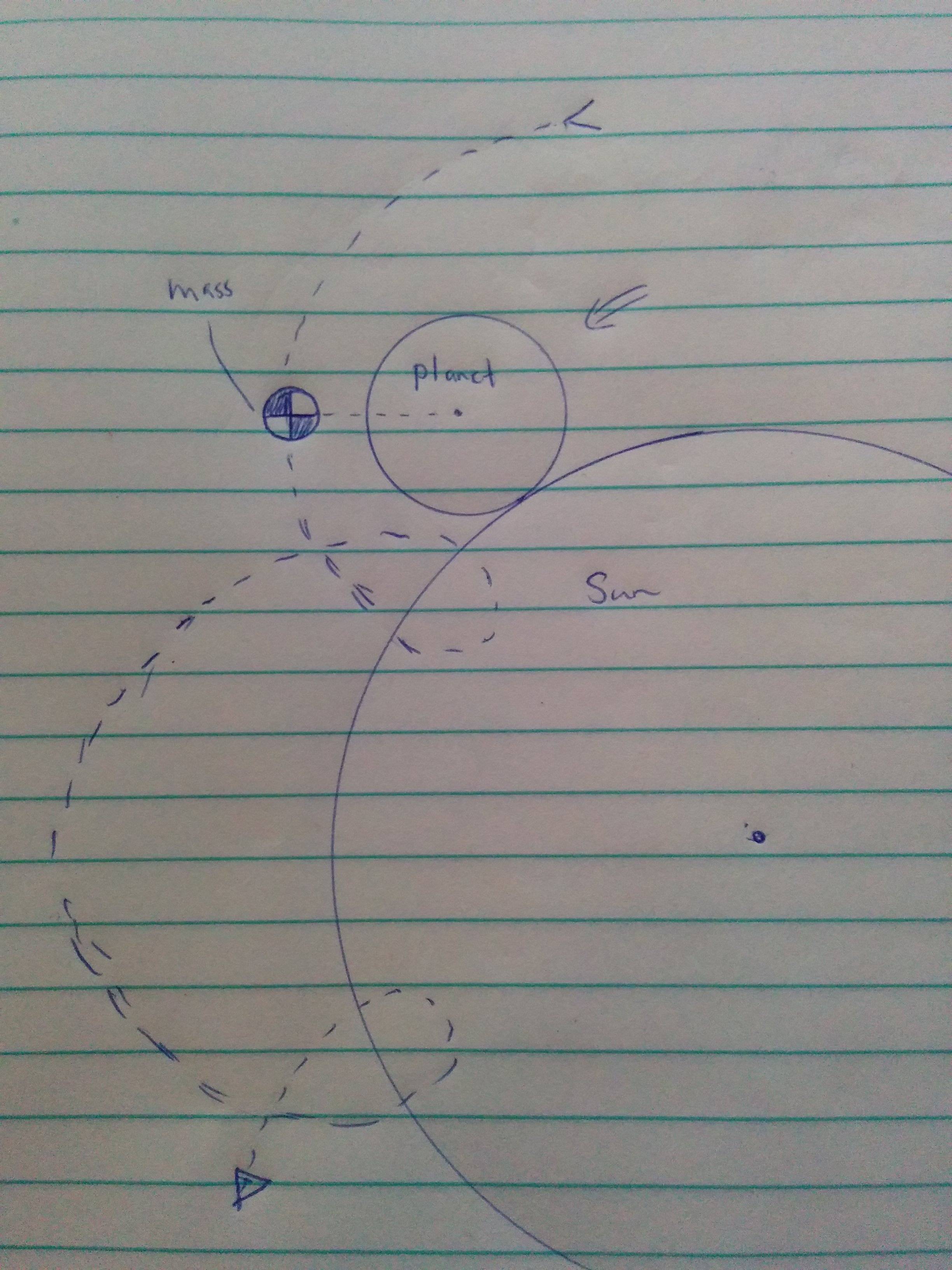
I'm trying to model the planar dynamics of a mass travelling about an epitrochoidal path due to being rigidly attached to a planet gear that rotates about a sun gear. Assume the gears to be circles that rotate about each other with no slip for reasons of simplicity.

Both the sun and the planet can rotate. The input to the system is a torque on the planet carrier, pushing the planet parallel to its path about the sun. The output is the torque of the sun.

As i have very little experience with modelling dynamics, i don't know where to start. I was thinking that separating the problem into a fixed and inertial frame may be best, but i'm not sure.

How should i go about showing the output torque as a function of the input torque and the system constants (e.g. planet mass, sun diametre, etc.)?

I drew a quick diagram of the system as a simple model with circles for reference.

Cheers :)

EDIT: To clarify, i've already solved the kinematics of the problem and have equations governing position, velocity, and acceleration for the mass according to the rotation, rotational velocity, and rotational velocity acceleration of the planet and Sun. What I want to find out is the dynamics. Specifically how torque on the planet carrier will affect torque on the sun.

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ In a planetary gear system you need either a fixed ring or a fixed sun. If both the sun and the planet can rotate freely as stated in your question, and if there are no other components in the system, then the motion you're describing would not be possible. Could you please clarify this point? The dynamics will be different for fixed-ring and fixed-sun configurations. $\endgroup$
    – ConjuringFrictionForces
    Commented Jul 15, 2017 at 14:10
  • $\begingroup$ I'm not sure why the motion would be impossible in a ringless system where both the sun and planet can rotate. A force acting on the middle of the planet (via the planet carrier) would induce rotation of both the sun and planet+mass at a ratio dependent on the rotational inertias in the system, and made variable dependent on the distance of the mass from the centre of the sun (because it changes it rotational inertia about the sun). $\endgroup$
    – Joe
    Commented Jul 16, 2017 at 6:18
  • $\begingroup$ Could I bump this question? I'm sure there's someone who can recommend a method of solving this if its possible. $\endgroup$
    – Joe
    Commented Jul 24, 2017 at 16:47

1 Answer 1

Reset to default
1
$\begingroup$

This may be what you are looking for - graph and source below :

epitrochoidal path

https://www.researchgate.net/figure/229731352_fig7_Fig-710-A-graph-of-the-epitrochoidal-path-that-the-moving-pins-follow-relative-to-the

Edit : the following have some examples of the equations necessary

http://mechdesigner.support/epitrochoid-the-curve.htm

And

http://mechdesigner.support/maths-fb-velocity-eqtns-2d-cam

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thats the motion of the mass yes, but i need to define the dynamic equations as described in the original post $\endgroup$
    – Joe
    Commented Jul 14, 2017 at 7:30
  • $\begingroup$ Added two links that give equations.... $\endgroup$
    – Solar Mike
    Commented Jul 14, 2017 at 7:48
  • $\begingroup$ Thanks for the links. However, I have already solved the kinematics to quite an extent, and its the dynamics that I'm concerned with. $\endgroup$
    – Joe
    Commented Jul 15, 2017 at 3:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.