1
$\begingroup$

Consider a simply supported beam, acted upon by a concentrated load P as shown

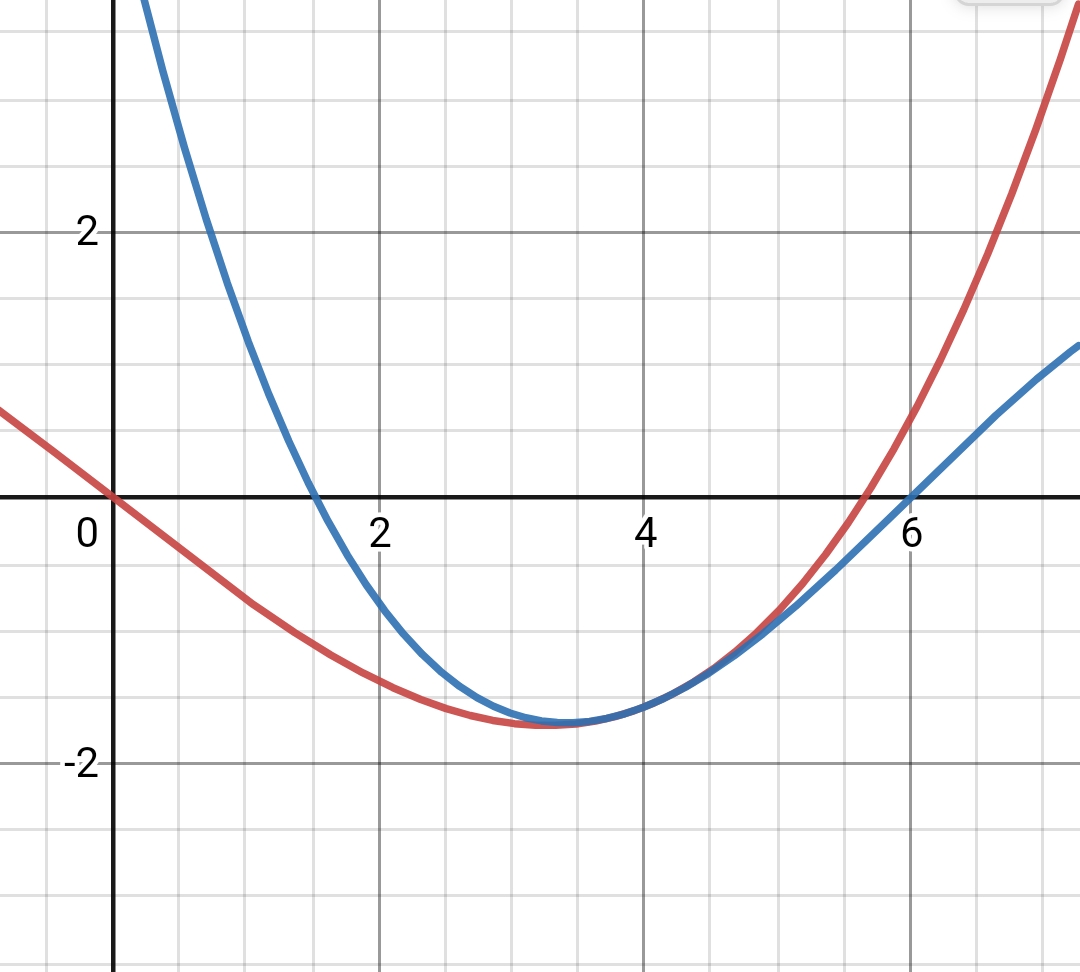
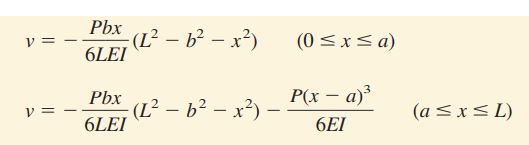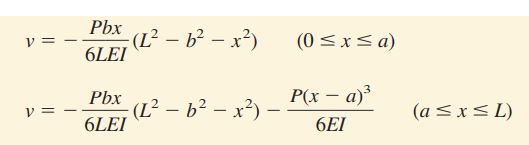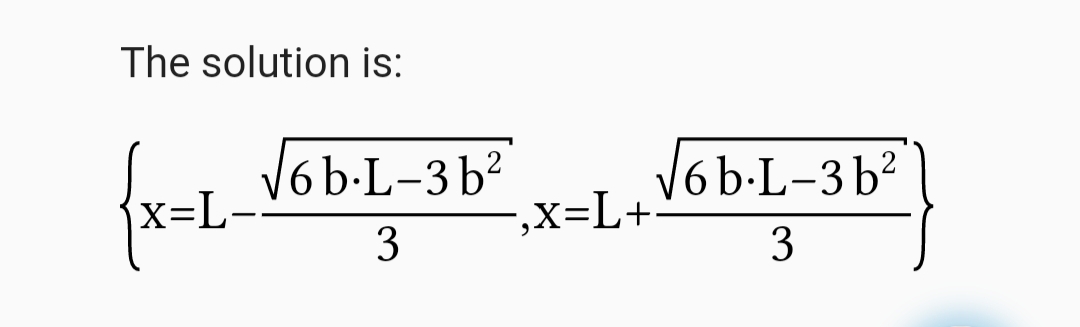enter image description here

By applying the method of successive integration, i.e. by using the differential equation

$$EI \frac{d^2v}{dx^2} = M$$

We can findd out the equation (i.e. $v= f(x)$ ) for the deflection curve:

enter image description here

I was interested in finding out the maximum deflection of the beam, which can be done by setting

$$\frac{dv}{dx}=0$$

However, there are two equations for v, one for interval 0 to a and second for a to L, which equation should I differentiate to obtain the maximum deflection?

The book that I follow, differentiates the first equation for v (0 to a), I don't understand why though.

Improve this question
$\endgroup$
6
  • $\begingroup$ Either should give the same deflection unless the beam is discontinuous at that point. $\endgroup$
    – Solar Mike
    Commented Mar 14, 2022 at 12:17
  • $\begingroup$ Why confused? Both will give the identical result, but the first equation is easier to work with, thus less likely to make mistakes. $\endgroup$
    – r13
    Commented Mar 14, 2022 at 14:38
  • $\begingroup$ It's not making sense to me, how can we determine a deflection which is in the portion 0<x<a, using the equation which is valid for a<x<L ? $\endgroup$
    – Harshit Rajput
    Commented Mar 14, 2022 at 15:35
  • $\begingroup$ To make it clear, I'm not talking about the deflection under the load, but the maximum deflection. $\endgroup$
    – Harshit Rajput
    Commented Mar 14, 2022 at 15:55
  • 1
    $\begingroup$ If you encounter an ambiguity, try to push through and investigate it rather than stopping. What do you get when you solve $\frac{dv}{dx}=0$ for the two intervals? Plot the deflection; where does the maximum deflection appear? What conclusions can you draw? $\endgroup$
    – Chemomechanics
    Commented Mar 14, 2022 at 20:26

1 Answer 1

Reset to default
2
$\begingroup$

As stated in the question, the equation for the deflection curves for the intervals $0<x<a$ and $a<x<L$ are given as

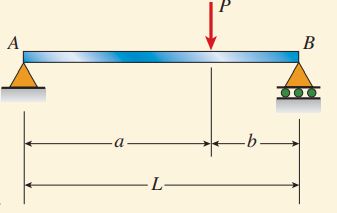
enter image description here

The maximum deflection in the beam can occur either to the left of the load or to the right, and we don't know what is the case here.

Let us start off by assuming the maximum deflection was in the portion $0<x<a$. If that were to be true then at some x lying between 0 to a the slope of the deflection curve for $0<x<a$ would've been zero. That x at which slope would've been zero can be determined by differentiating the equation for deflection curve for $0<x<a$ and then setting it to 0. This would give us,

$$x= \sqrt{\frac{L^2 - b^2}{3}}$$

If this indeed is the value of x, at which the deflection is maximum and if it occurs in $0<x<a$, then $x<a$. If x turns out to be greater than a, that would mean this result is not feasibe. Let us try to investigate whether this x is greater than or less than a.

$$x= \sqrt{\frac{L^2 - b^2}{3}}$$

$$x= \sqrt{\frac{a^2+2ab}{3}}$$

$$\sqrt\frac{a^2+2a.a}{3} > \sqrt\frac{a^2+2ab}{3}$$

$$a > \sqrt\frac{a^2+2ab}{3}$$

$$a > x$$

The value of x, indeed is in the interval 0<x<a. Thus, our assumption was right and the maximum deflection does occur in the region 0<x<a. To find this deflection we can substitute $$x= \sqrt{\frac{L^2 - b^2}{3}}$$

in the equation of the deflection curve for $0<x<a$

We could extend the analysis and disprove that the maximum deflection occurs in the interval $a<x<L$. In the same manner, we can first assume that the max deflection was occurring in the portion $a<x<L$, then we can determine the x at which it would've occurred (if it would've occurred), by differentiating and putting to 0, the equation of the deflection curve for $a<x<L$.

The equation thus obtained will be a qaudratic one, which has solutions

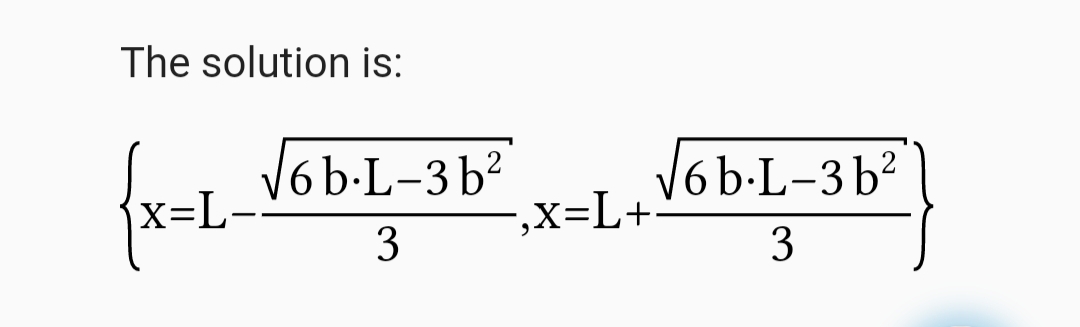
enter image description here

The second value of x is greater than L, hence this is not a feasible solution. Also, by using inequalities we can prove that the first value of x, will be <a. Since this value is <a, we conclude that even the first value of x in the solution above is not feasible since x should occur between $a<x<L$ if the max deflection were to truly occur in the region $a<x<L$.

Here is a plot of the deflection curves for a 6m length of the beam-

enter image description here

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Nice analysis!! $\endgroup$
    – Chemomechanics
    Commented Mar 16, 2022 at 19:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.