1
$\begingroup$

I'm wondering if somebody could explain the 4 position method a bit more understandably. I am trying to follow the explanation in here. What I fail to understand is how does the actual fourbar linkage in this case look like?

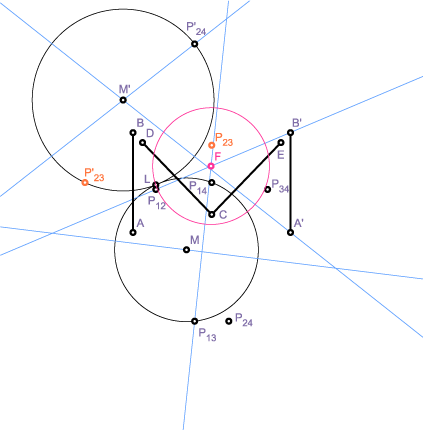
M bar four positions

Image 1: Situation described.

From the image and description I gather that:

  • F is one of the ground joints (pink)
  • The pink Circle is length of that link (link 3)
  • P23 and P'23 are location of next link joint, and thus the length of that link is F to P'23 minus the pink circles radius (link3)

However what I don't understand from the description is where the other ground joint is. Does it happen to be M'? But that seems awfully short when looking at the demonstration piece? What about P13?

Improve this question
$\endgroup$
1
  • $\begingroup$ Perhaps a tag kinematics and bar-linkage could be in order, still haven't got the points to do so. $\endgroup$
    – joojaa
    Commented Apr 17, 2015 at 20:26

1 Answer 1

Reset to default
2
$\begingroup$

Answer

The other ground link is not shown and neither is the floating link. This diagram only shows

  • The 4 desired output link positions
  • The 6 poles generated from those positions
  • The process involved to obtain 1 link

Your first two points are correct, however, P23 and P'23 do not form the next link joint. P23 is the pole of the rotation from DC to CE and P'23 is the reflection of P23 through an invisible line which passes through P13 and P12. P'23 and P'24 are used solely to find M'.

I am not very familiar with Burmester's Theory, but I imagine that the process to find the other links involves picking another set of poles, generating another set of mirrored points, and then using another set of circles like M and M' to find the next ground link.

Supplementary Material

Some other reference reading that may clear things up.

  1. Another earlier article by the same author on four-bar linkages
  2. The Wikipedia page for Burmester's Theory

You may also want to download the GeoGebra file attached to the article and GeoGebra itself. You can play around with it and see how the diagram was generated.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Yes i played with the geogebra file. And yes i am familiar with the 2 and 3 position methods they are just based on simple triangles (they are commonly taught out since it takes 20 minutes for even nontechnical people like lawyers to learn, yes my pedagogics exersise was this). But yes this helps a bit. Altough i kindof figured this one out allready. $\endgroup$
    – joojaa
    Commented Apr 17, 2015 at 22:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.