2
$\begingroup$

A mechanism popped up in my mind when I was studying kinematics, see picture below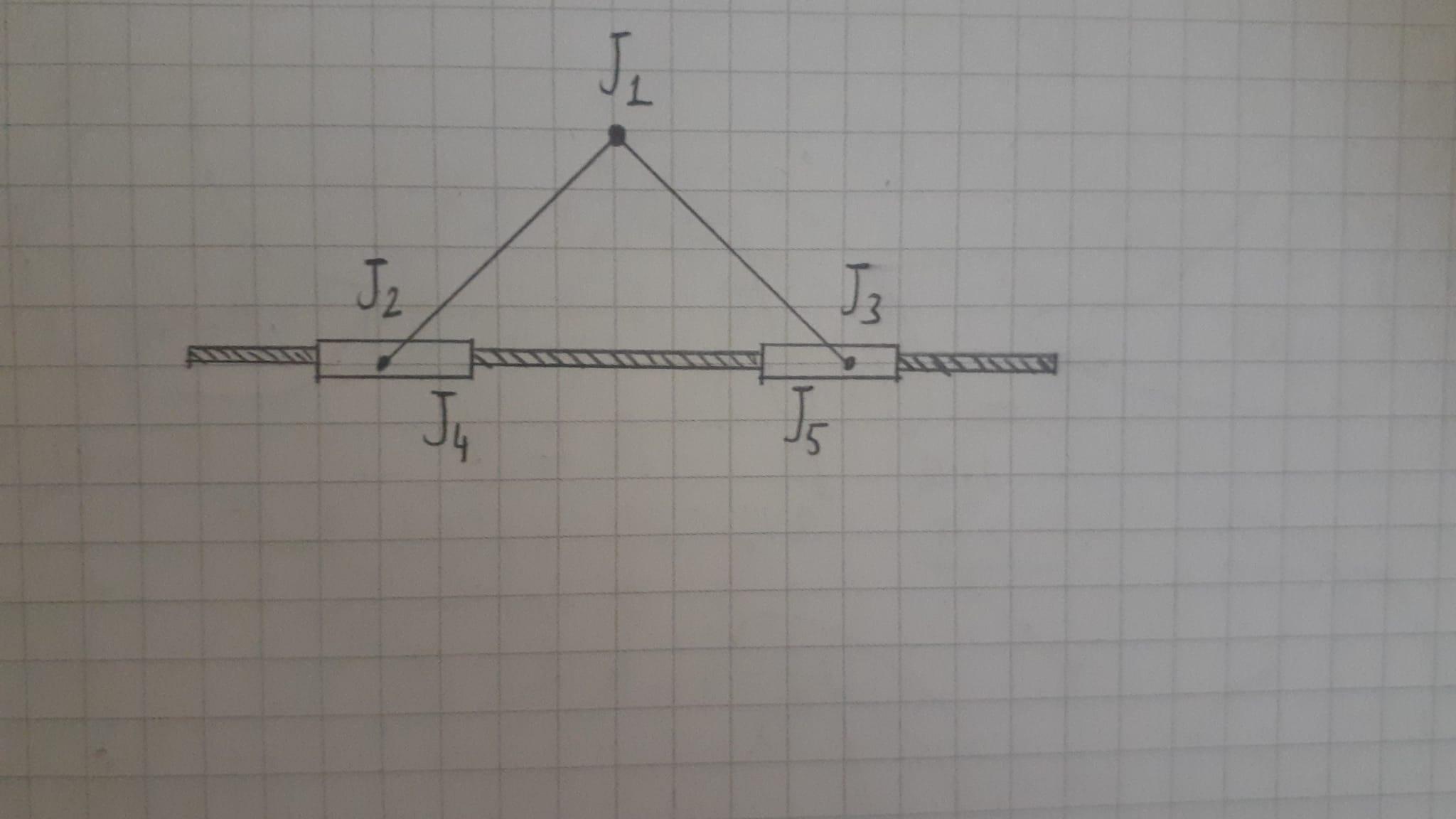enter image description here

$J1,\ J2,\ J3$ are rotational joints and there are two translational joints $J4$ and $J5$ between two sliders and the cross hatched rail. Sliders are free to move horizontally towards or away from one another. Now when I imagine a motion in which a force is applied vertically at $J1$, pushing or pulling on the said joint, thus sliders move away or come closer, I think it is a one degree of freedom mechanism. However the famous formula $3n-2j-3$ where $n$ is the number of links and $j$ is the number of lower pair joints, gives two degrees of freedom. Considering the rail as a ground link, with two sliders and two arms ($J1J2$ and $J1J3$) we have $n=5$ and we have $j=5$

$3*5-2*5-3=2$

So, what is with that?

Improve this question
$\endgroup$
4
  • $\begingroup$ I imagine a motion in which a force is applied vertically at J1, - and what happens when you apply force at J1 horizontally? How does sliders move then? $\endgroup$
    – elementiro
    Commented May 18, 2021 at 23:05
  • $\begingroup$ @elementiro They would move in the direction of that horizontal force, but their motion will still be dependent. Am I wrong? $\endgroup$
    – Ali Kıral
    Commented May 18, 2021 at 23:39
  • $\begingroup$ If you can't represent that movement as the sum of multiplies of movements in already established degrees of freedom then it isn't dependent. For the first DOF you've denoted displacement of J4 is opposite to displacement of J5 but equal in absolute value. Does it stay the same when the sliders move in direction of the force? $\endgroup$
    – elementiro
    Commented May 19, 2021 at 9:18
  • $\begingroup$ @elementiro I was missing the horizontal motion of J1 all along, I understand now $\endgroup$
    – Ali Kıral
    Commented May 19, 2021 at 21:51

1 Answer 1

Reset to default
0
$\begingroup$

This is a 2 degree of freedom mechanism.

For me its easier to think of it by translation of the horizontal sliders. When you know the position of the both the horizontal sliders then you know the position of the mechanism.

Another way to look at it: If you know the position of J1 (which has two degrees of freedom, then you automatically know the position of the horizontal sliders, and the angles.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ I got it. The absolute position of the entire triangle with sliders introduces one of the two degrees of freedom. $\endgroup$
    – Ali Kıral
    Commented May 19, 2021 at 21:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.