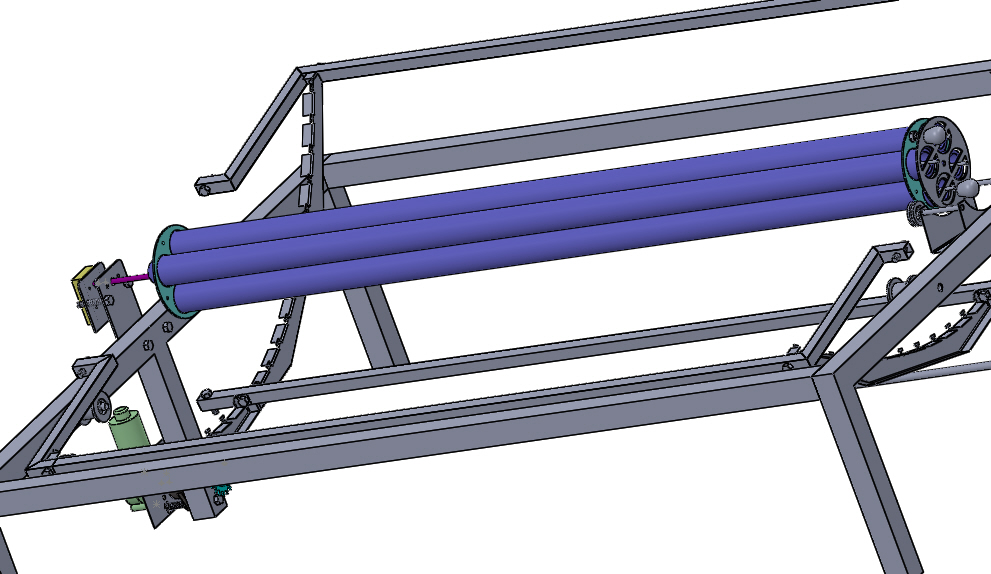
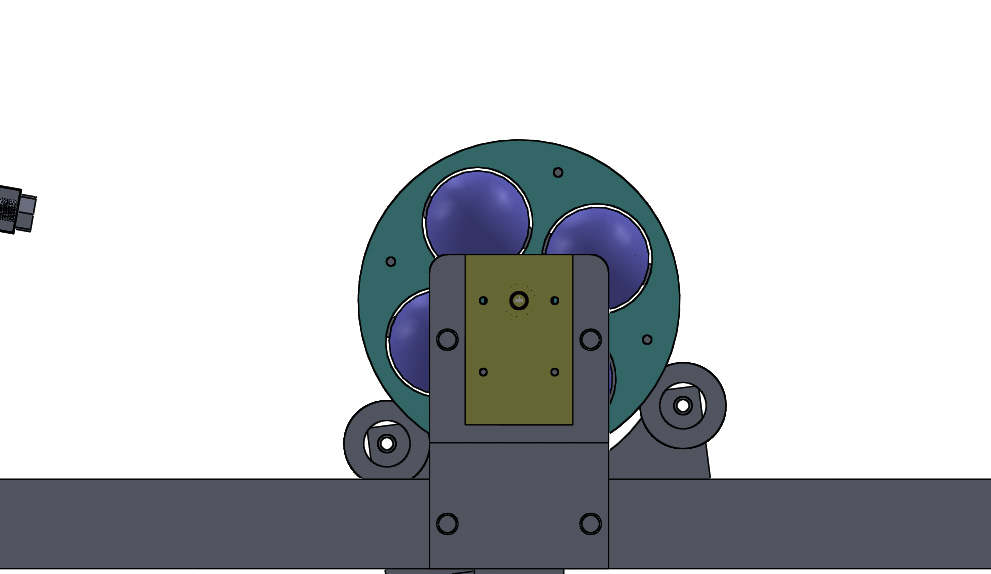
The torque required during the acceleration phase can be calculated using the formula:
$$T=Ia$$
where: $T = \text{Torque (Nm)}\\ I = \text{moment of inertia (kg.m²)}\\ a = \text{angular acceleration (rad/s²)}$
The moment of inertia of a solid disc is $I=\frac{1}{2}MR^2$, where $M=\text{mass (kg)}$, and $R=\text{radius (m)}$.
In this case, the moment of inertia will be lower than that of a solid disk, since the arrangement of the glass tubes concentrate the mass closer to the axis. Therefore, it is a safe estimate to use for calculation, and will ensure that the selected motor has sufficient power. Using the numbers provided in your question, the radius of the disc is found to be $0.09\text{ m}$
$$I=\frac{1}{2}(23)(0.09)^2=0.0932\text{ (kg.m²)}$$
Using the numbers that you provided in your comment, the angular acceleration can be calculated by converting the steady-state speed $100\text{RPM}=10.47\text{rad/s}$, and dividing by the 'spin up time' in seconds
$$a=\frac{10.47}{2.5}=4.19\text{ (rad/s²)}$$
Combining these two calculated values in the original equation gives the peak torque during acceleration:
$$T=(0.0932)(4.19)=0.39\text{ Nm}$$
It's important to note, however, that the torque generated by any motor is not constant in relation to angular velocity.
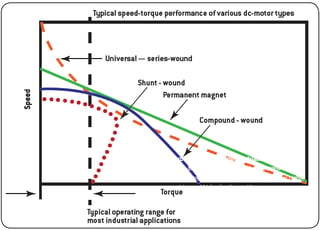
If you specify a motor which is advertised as being capable of sustaining $0.4\text{ Nm}$ at $100\text{ RPM}$, then you should be OK, since the available torque will be higher at lower speeds. This corresponds to a power of $\approx 4.2\text{ W}$, which is certainly achievable with a small DC motor.
If you want to limit the spin-up-speed to take $2.5\text{ s}$ in any case, then this should be done using e.g. PWM control of the motor.