As far as I know, moments/torques occur when forces applied are not in line. However, I often see moments/torques being applied at a single point in free body diagrams.
How can moment/torque be applied at a single point?
Illustration Below:
Caption: The above image shows an ideal moment acting at the center of a beam. An ideal moment is one which is not associated with a force. Link for the page
As you say, torques are caused by forces not in line. In this case you have a "couple," which is caused by TWO equal and opposite forces not in line.
My recommendation is that you draw an EQUIVALENT problem with an upward force and downward force of equal magnitude acting the same distance away from the point of application of the couple. Notice then that it doesn't actually matter which point we take the moment about, you get the same couple about every point. Thus, a couple, or a "pure moment" is a FREE vector. You can place it anywhere on the body and it has the same effect on the deformation or the motion.
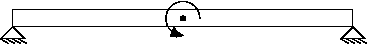
There's a reason why such moments are called "ideal". That's because they're idealized, not real. They are a simplification of reality.
One way you could get an ideal moment in a beam is by welding two rigid vertical bars at the midpoint, one above and one below the bar. And then apply opposing forces at the extremities of the bars. As far as the beam is concerned, it will suffer an ideal bending moment, without any associated forces:
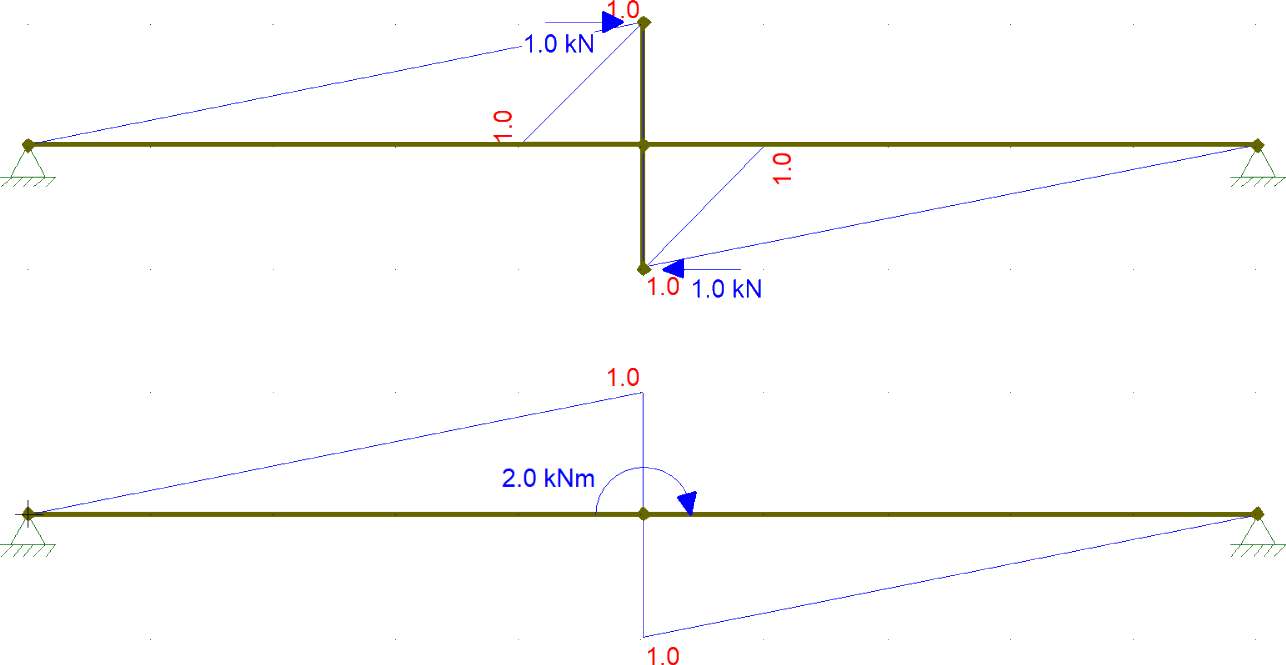
For something a bit more reasonable, imagine the following structure. Beam C is the only one with any loading (ignore self-weight). It is supported by beams B, which are themselves supported by beams A, which are supported by pinned supports at their extremities:
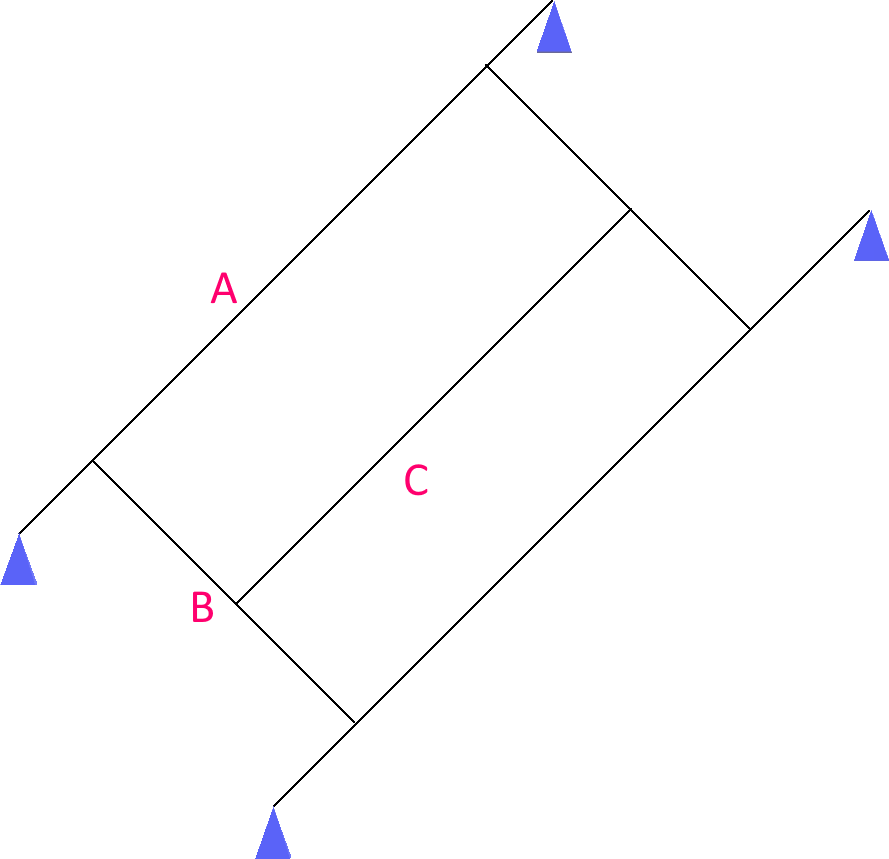
The fixity at the connection of beams B and C means that beam C will suffer a (small but non-zero) bending moment at the connection, which gets transferred to beam B as a concentrated torsional moment (along with the shear support reactions). Likewise, the connection between beams A and B will have the same bending moment in B transferred as torsion to A. However, the torsion in B also gets transferred to A as a concentrated bending moment.
Obviously, these concentrated bending-moment/torsion transformations can be easily described in terms of internal force couples as well.
Isolated moments, as shown here are more of a mathematical construct than a realistic construct. That said, in three dimensions this is completely possible. A mounted pneumatic drill turning a bolt at the midpoint of the beam (with minimal loading into the beam) would cause this concentrated moment. The motor applies a torque to the bolt. This model works well to simplify the complex drill forces, and accurately describes the stresses in the beam when the distance between the flats on a bolt is small compared to the the length of the beam. This substitution from a complex motor to a simple concentrated moment is known as Saint-Venant's Principle
A shorter beam would result in more complex boundary conditions and the need to consider higher order elastic effects. This is the primary difference between a continuum mechanics researcher, who would model these effects with partial differential equations, and an engineer, who would multiply the Saint-Venant stresses with a stress-concentration factor and design accordingly.
An applied pure torque does not have a point associated with it and it is said to shared with the entire body, as opposed to forces that act through a point.
This is entirely equivalent to the statement that rotational motion is shared with the entire body, as opposed to linear velocity which is only defined at a single point.
It is almost impossible to produce a pure torque in real life, with net force zero. You can transmit forces without a torque using a bearing, but you cannot transmit a torque without any forces. At least not mechanically.
Torques represent a force at a distance, and a pure torque is mathematically equivalent to a zero force at infinity.
This is similar to rigid body motion, where linear velocity is a representation of a rotation at a distance, and a pure translation is zero rotation about a infinite point.
