That's because the finished structure with full loading is not the same as the finished structure as a composite of the loading of each construction phase. And the latter is the correct solution.
This can be seen with the trivial structural model below. The first two structures represent the two construction phases, and the last one is the complete structure with all the loading. Here are its axial results.
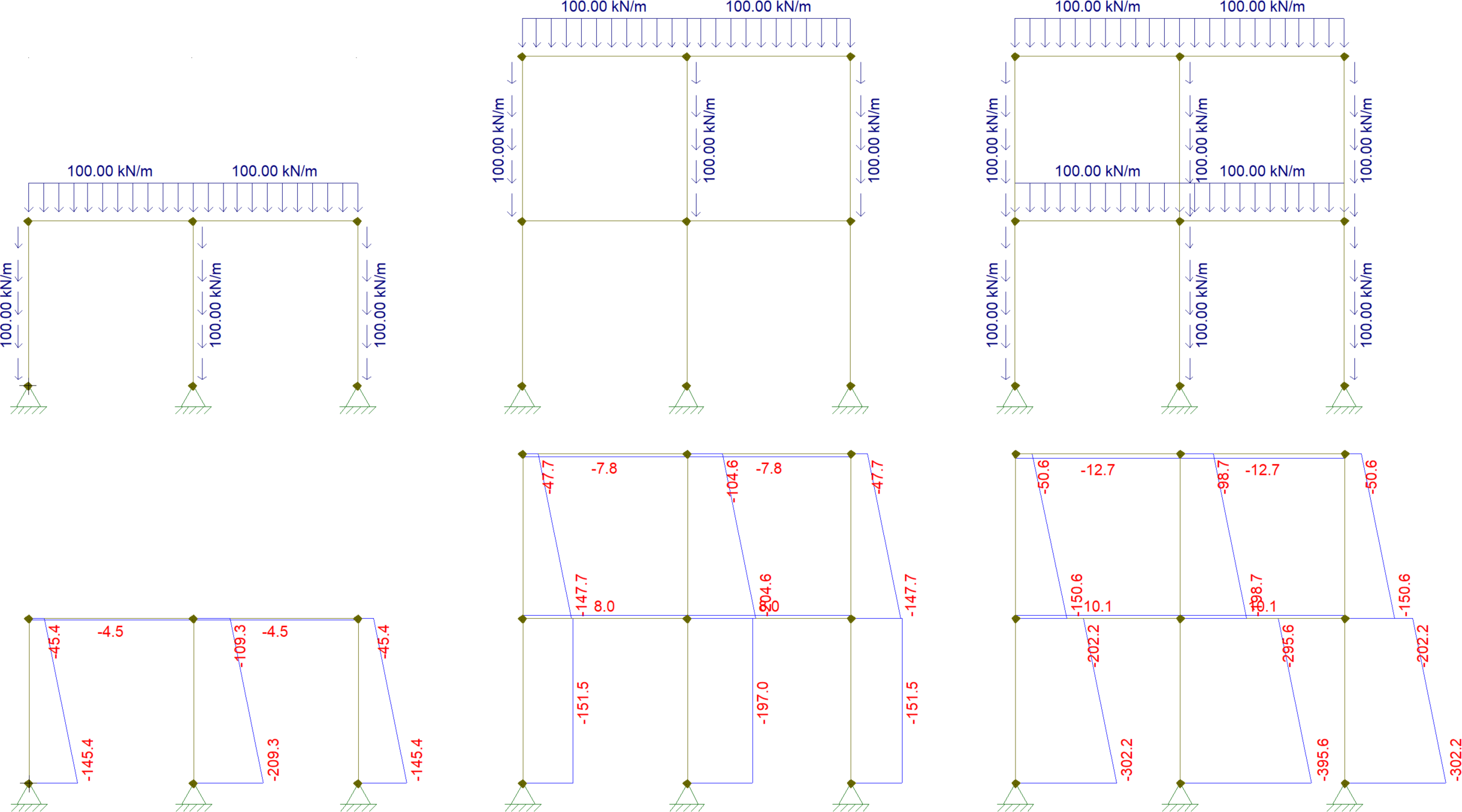
Note that the compression at the very top of the right-most column is different between the second phase (47.7 kN) and the total structure (50.6 kN). Indeed, every single one of the values is different between the total structure and the addition of the first and second phases. For example, the base of the central column has 395.6 kN in the total-structure-total-loading case, while the addition of both phases gives us $209.3+197=406.3\text{ kN}$.
Why is this?
Well, the reason is that the completed structure is applying the loading of the first phase on the whole structure, when in fact those loads are only being supported by the first phase. Compare the results below:
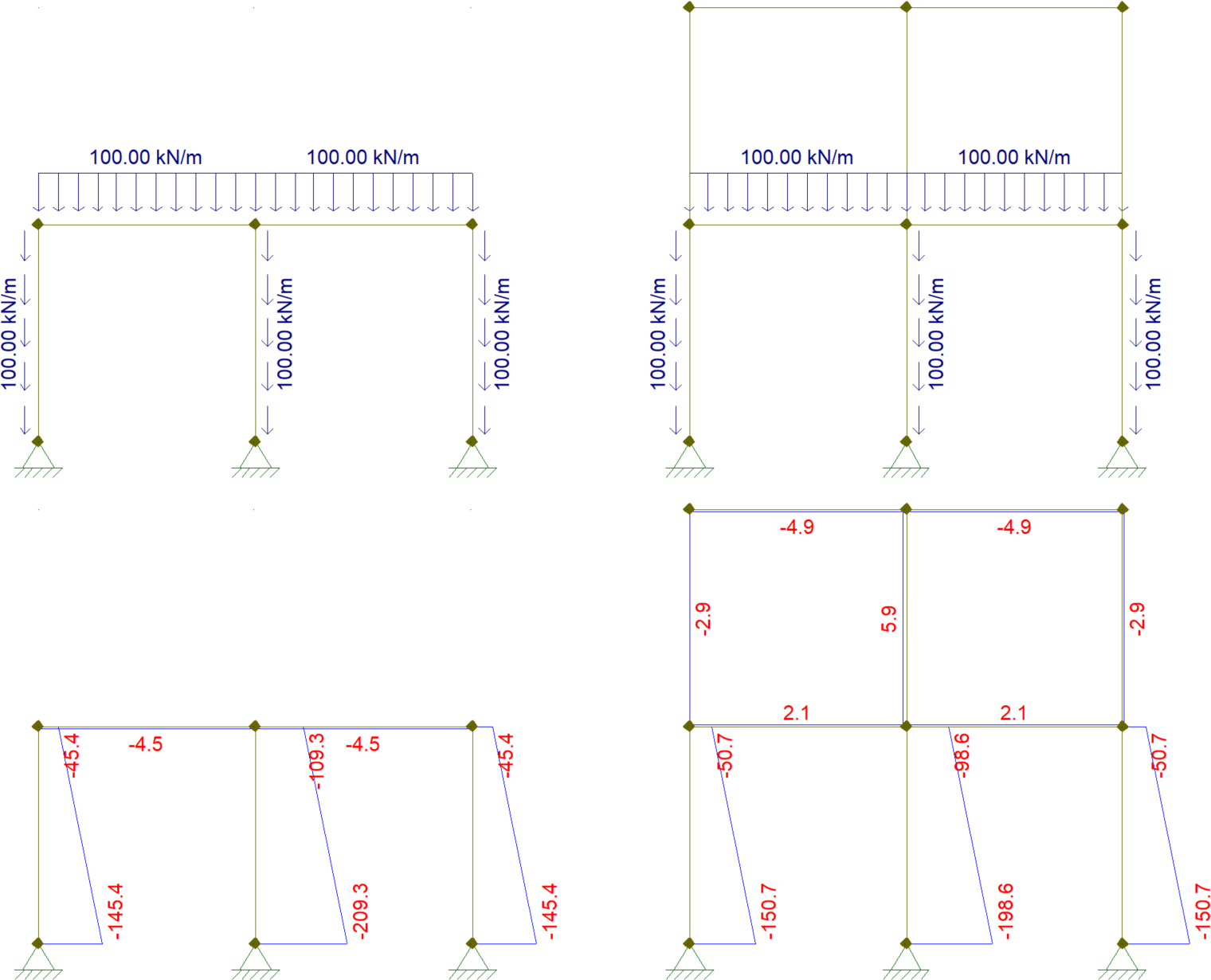
The existence of the second level is causing the loads to be redistributed. The central column is under less loading, while the outer ones are supporting a greater portion of the load. Hell, the second phase is even presenting forces even though it didn't actually exist when those loads were applied.
So, why does the existence of the second phase modify the results from the loading of the first phase? To get an intuitive understanding, let's look at the structure's deflection. But let's do so while telling a story.
The story starts by assuming the second phase is only composed of the external columns, with no central column and no second-phase beams. In this case, the loads from the first phase cause an "inwards" rotation on the top-external nodes of the first phase. Since we're assuming the second-phase columns are also there, those columns also get rotated. However, this is a solid-body motion, with no deformation and no internal forces or moments generated.
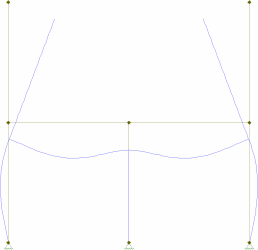
Now, the real second phase obviously has the beams, so let's include them (while still leaving the central column out). The current deflection of the external columns will obviously cause a shortening of the beams, so they'll be under compression (as can be seen in the axial results above). However, every force has an equal and opposite reaction, so the compressed beams will also push the external columns outwards, counteracting the vast majority of the inwards motion we see above. Indeed, the beams in my model are so axially stiff that they barely budge horizontally at all, such that the columns feel as if the node is almost fixed in the horizontal direction. In this case, the top of the second-phase columns ends up rotating outwards, and the beams suffer negative bending moment (as can be seen by their deflection which causes tension on the upper fiber).

Now, the loads have caused a compression and shortening of the first phase's central column. However, the second phase's beams are presenting uplift at midspan, where the second phase's central column should be. Therefore, that column will obviously be under tension as it needs to stretch to resist that motion. That tension will need to be transmitted down to the ground support, so it actually represents an overall reduction in the compression of the first phase's central column (it therefore stands to reason that the external columns end up taking that additional load).
Note that if the axial stiffness of the second-phase beams were very low compared to the columns' bending stiffness (and I mean low, given elements' natural inclination to be much stiffer axially than under bending), they wouldn't offer much resistance to the external columns' desire to move inwards, and would actually present positive bending moment (tension on the inferior fiber):
In this case, it's possible that the structural behavior is different, with the central column absorbing loads from the external ones (the unloaded second phase presents tension in the external columns and compression in the central one). However, for this particular model I had to use unreasonably low axial stiffnesses to achieve that reversed result.
An argument could be made that over time, due to long-term redistribution effects, the loads will in fact approach the "total structure under total loading" results. But that's beyond the scope of this question.
Also, depending on the precise type of structure, the phases might be independent from one another (due to the existence of hinges, for example). In that case, yeah, the results should be the same.
If anything, I'd suggest doing an envelope of results between "sum of each phase" and "total structure" and designing members for the greater of the results.
Also, this analysis is obviously only valid for permanent and construction loads. Once the structure is totally built, all variable loads are supported by the total structure.