0
$\begingroup$

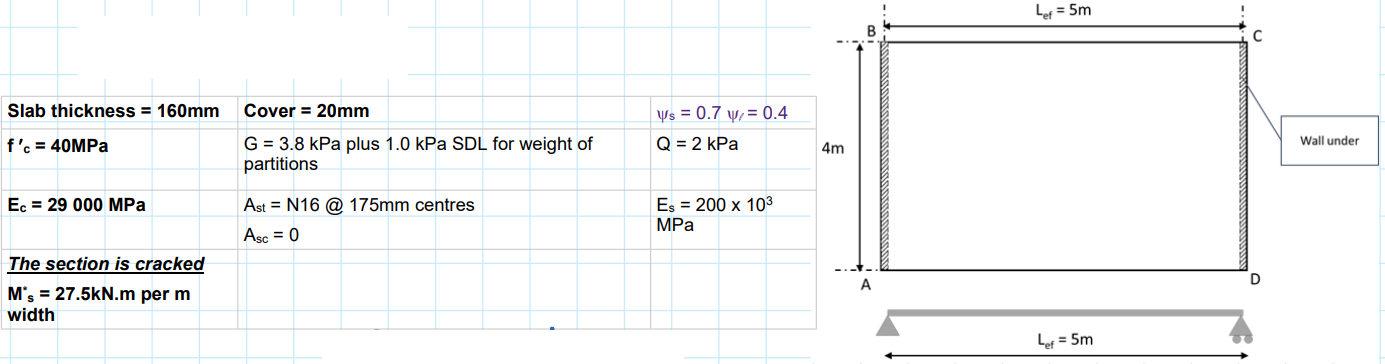
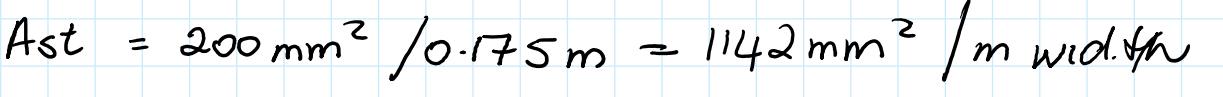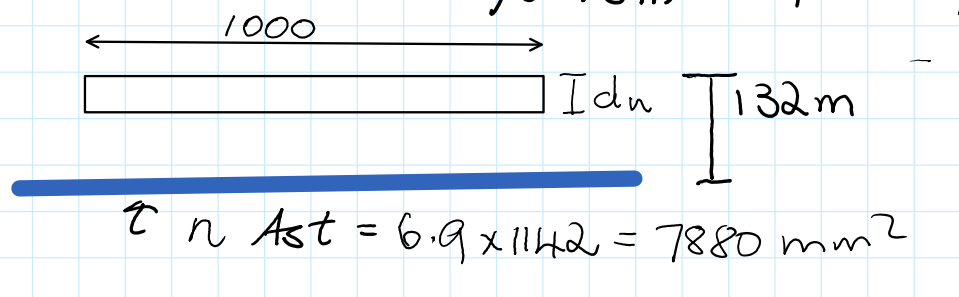I have the following problem, which I already know the general steps into solving.

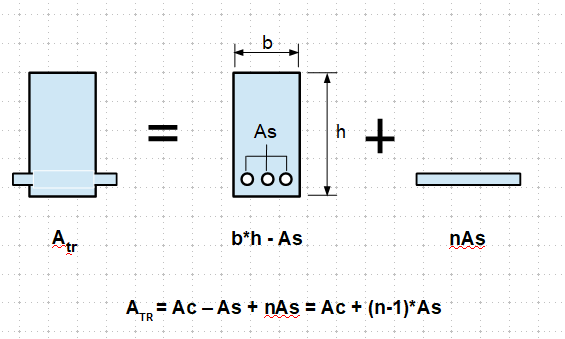
enter image description here

enter image description here

My general approach is: calculate I cracked as we are already told that the section is cracked, and then finally use $My/I$ to calculate the stress.

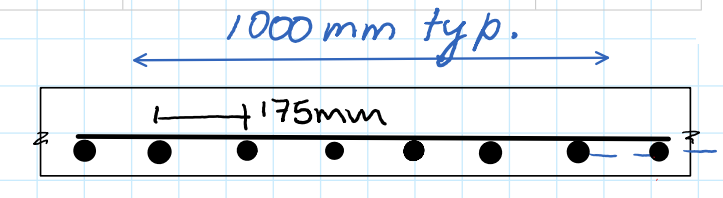
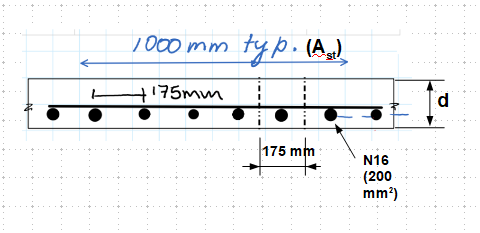
But in order to do that I need to calculate the area of steel, so what does$ Ast = N16 @ 175mm $ centres means? My lecturer drew the following diagram but I still don't get it.

enter image description here

And then he did this to calculate the area:

enter image description here

Why do you divide to find the area of the steel? How do I interpret his diagram and his calculations? Thank you.

UPDATE:

Why do we do $n \times A_{st}$? enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

The notation 'Ast = N16@175mm centres' means 16mm diameter bars spaced at 175mm centres (as per your lecturer's cross sectional diagram). 'N' would be the type of bar which would depend on the country/code/standard implied in the question.

To calculate the area of steel in a 1m wide strip of slab: Area of 1 bar is pi*((16/2)^2) = 201mm2 (they have approximated to 200mm2). The bars are every 175mm, therefore the number of bars in a nominal 1000mm wide strip of slab is 1000/175 = 5.714 (multiplying by 5.714 is the same as dividing by 0.175). The total steel you can allow in your nominal 1m wide strip of slab is therefore 5.714*201 = 1149mm2 (or 1142mm2 as he has approximated).

Note you end up with a non integer (5.714) number of bars, which is fine(indeed correct) in this case as you are considering a 1m wide subsection of a wider slab, 5.714 is just the avg number of bars per metre width. You will end up calculating the bending capacity as moment/metre width of slab.

Hope this helps!

Improve this answer
$\endgroup$
5
  • $\begingroup$ You can't place the fractional bar in concrete, so for 5 spaces, you should end up with 6 physical bars. $\endgroup$
    – r13
    Commented Aug 11, 2022 at 17:55
  • $\begingroup$ You are confusing placing fractions of bars in real life with considering fractions of bars in an arbitrarily selected theoretical width of slab. If you were calculating the capacity of the entire slab as a whole in kNm, yes, you would use the total whole number of bars, but as you are calculating per metre strip, you use the precise area of steel in 1000mm width, namely 1000/175*(bar dia/2)^2. If you were calculating capacity per 175mm wide strip, you would use 1 bar. If you were calculating capacity per 260mm width you would do 260/175 = 1.49x(area of a bar). $\endgroup$
    – PM-14
    Commented Aug 11, 2022 at 18:01
  • $\begingroup$ Thanks so much, for the clear explanation. I have one more question why do we do $n \times A_{st}$ when calculating the final area of steel? Sometimes I see my lecturer use $A_{trans} = (n-1) A_{st}$. $n$ is the modular ratio $\endgroup$
    – CountDOOKU
    Commented Aug 12, 2022 at 5:10
  • $\begingroup$ Hi, glad it helped. You would usually use modular ratio if calculating the 2nd moment of area of a composite section, e.g. if you were trying to carry out an elastic analysis. Because concrete and rft steel have different elastic moduli (~ 30GPa vs ~210GPa), you have to convert the area of one of the materials into an 'equivalent area' of the other material - so 210/30 = 7 (your lecturer has 6.9) and so converting your Ast to an equivalent area of concrete would give 6.9xAst. You can then do your calculation based on the concrete modulus alone. $\endgroup$
    – PM-14
    Commented Aug 12, 2022 at 11:06
  • $\begingroup$ You might find this page useful: roymech.co.uk/Related/Construction/Concrete_beams_theory.html It discusses an elastic (i.e. 'uncracked') and an ultimate (i.e. cracked) analysis of a beam. Bear in mind the exact parameters and approximations(for example of the rectangular stress block) may vary depending on which design code you are using, but most codes accommodate methods similar to these. A rigorous analysis using the actual non-linear concrete stress block and (for any non-rectangular sections) requires an iterative solution that is best implemented in software. $\endgroup$
    – PM-14
    Commented Aug 12, 2022 at 11:06
1
$\begingroup$

Let's calculate the equivalent reinforcing steel area ($Ast)$ in a $1 m$ strip from the given reinforcing configuration ($1-N16@175 mm$ spaced center to center) using the concept of "consistent reinforcing ratio".

The reinforcing ratio of the $1 m$ strip is, $\rho_1 = \dfrac{Ast}{1 m*d}$, and the reinforcing ratio for $1 - N16$ at $175 mm$ spacing is, $\rho_{act} = \dfrac{200 mm^2}{0.175 m*d}$, and, since $\rho_1 = \rho_{act}$,

  • $\dfrac{Ast}{1 m*d} = \dfrac{200 mm^2}{0.175 m*d}$

With "$d$" cancels out, the equivalent reinforcement in $1 m$ strip, therefore, is,

  • $\dfrac{Ast}{m} = \dfrac{200 mm^2}{0.175 m} = 1142 mm^2/m$

enter image description here

ADD: For add'l question in comment

enter image description here

Improve this answer
$\endgroup$
4
  • $\begingroup$ Hi r13 hope you are well, thanks for answering my question. I have one more question why do we do $n \times A_{st}$ when calculating the final area of steel? Sometimes I see my lecturer use $A_{trans} = (n-1) A_{st}$. $n$ is the modular ratio $\endgroup$
    – CountDOOKU
    Commented Aug 12, 2022 at 5:12
  • $\begingroup$ the units for 1m/0.175m also cancel out. dropping the 1m from the calculation is the same thing the teacher did which is one of the things that confused the OP in the first place. You should carry it in your calc to avoid and voila here is your final answer that a lot of students cant make the obvious jump between the second last line and last line of a solution. Simplification is easy when you know what you are doing. Sometimes its not so simple when you are learning. $\endgroup$
    – Forward Ed
    Commented Aug 12, 2022 at 5:41
  • $\begingroup$ @CountDOOKU I suggest making your latest question in another thread with examples that confuse you. In short, the total area of an uncracked RC x-section A = Ac (area of concrete w/o deducting area occupied by steel) + nAs (equvalent Ac of steel) - As (area occupied by steel) = Ac + (n-1)As. Note the last term is sometimes ignored for its influence is usually small and negligible. $\endgroup$
    – r13
    Commented Aug 12, 2022 at 16:00
  • $\begingroup$ Hi r13 thanks for the added comment and diagram. Really appreciate it, I understand now. Many thanks again. $\endgroup$
    – CountDOOKU
    Commented Aug 13, 2022 at 8:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.