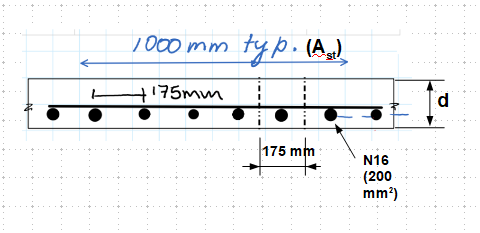
Let's calculate the equivalent reinforcing steel area ($Ast)$ in a $1 m$ strip from the given reinforcing configuration ($1-N16@175 mm$ spaced center to center) using the concept of "consistent reinforcing ratio".
The reinforcing ratio of the $1 m$ strip is, $\rho_1 = \dfrac{Ast}{1 m*d}$, and the reinforcing ratio for $1 - N16$ at $175 mm$ spacing is, $\rho_{act} = \dfrac{200 mm^2}{0.175 m*d}$, and, since $\rho_1 = \rho_{act}$,
- $\dfrac{Ast}{1 m*d} = \dfrac{200 mm^2}{0.175 m*d}$
With "$d$" cancels out, the equivalent reinforcement in $1 m$ strip, therefore, is,
- $\dfrac{Ast}{m} = \dfrac{200 mm^2}{0.175 m} = 1142 mm^2/m$
ADD: For add'l question in comment