1
$\begingroup$

I’m building a friction wheel drive electric Unicycle.

Something like this; enter image description here

My question is how much torque should the friction wheel drive motor have considering the total mass of unicycle including rider is 100kg?

Additional information;

I'd like the unicycle to go at speeds of up to 6 miles per hour, and climb up hill a slope of 45 degrees.

The unicycle would not be self balancing, the rider would have to balance it themselves by using throttle and brake.

The rider would be tilting themselves forward at an angle of 45 degrees.

Improve this question
$\endgroup$
3
  • $\begingroup$ If you were pedalling, how much power would you need for the speed you want? Friction wheel wil need to deliver the same. $\endgroup$
    – Solar Mike
    Commented Apr 23, 2022 at 17:08
  • $\begingroup$ I edited the question and changed the slope angle from 30 degrees to 45 degrees, and also added that the rider would be tilting themselves forward at an angle of 45 degrees too. $\endgroup$
    – Rayyan Javed
    Commented Apr 24, 2022 at 10:23
  • 1
    $\begingroup$ A friend had a friction drive ( gasoline) bike ( Travis) in 1950. We thought the friction drive was poor. $\endgroup$
    – blacksmith37
    Commented Apr 24, 2022 at 15:15

2 Answers 2

Reset to default
1
$\begingroup$

The speed is not really important, how fast it reaches that speed (acceleration) is relevant.

To move 100kg up a ramp of 30 degrees with a speed of 6miles

$V=6*\frac{1600m}{1mile}=9600mh=2.6m/s$

$$2.66*sin(30^ \circ)=1.33m/s$$

The vertical component of force, Fv

$$F=mgsin(30^ \circ)=100*9.8*(1/2)=490N$$

$$P=F*V=490N*1.33m/s=651.7*1.1(friction and loss)=716Watts$$

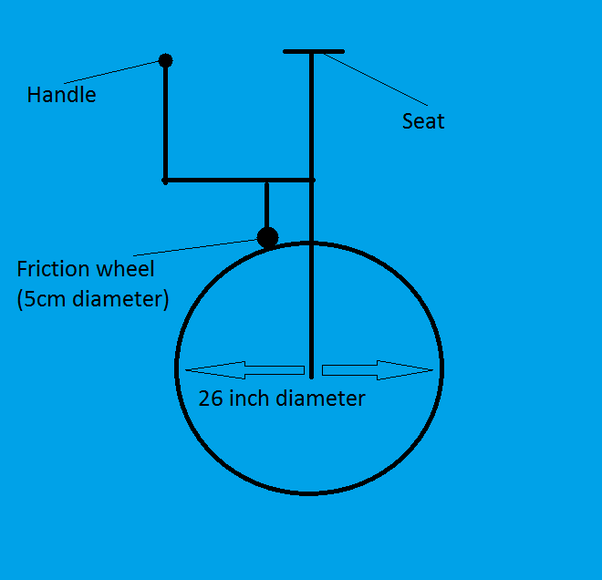
Let's assume 10%friction and loss, the torque of the monocycle wheel (big wheel) is

$$T=490*1.1*26*0.025=175Nm$$

The torque of the friction wheel is proportional to the radius of the friction wheel divided by the radius monocycle wheel multiplied by the torque of the big wheel.

$$T_{frictionwheel}=175.175\frac{R_{frictionwheel}}{R_{monocicle}}$$

Edit

the rider has to tilt his body same as the angle of slope to make equilbrium. but this doesn't have any effect on the torque.

however if the rider want to accelerate with an acceleration of $a , $ he needs to bend an addition angle of $ arctan(a/g) $ with g, being gravity acceleration and the friction wheel torque has to be increase by the same ratio.

also as to the slope of 45 degrees just plug in $mgsin(45)$ in the above calculations.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I edited the question and changed the slope angle from 30 degrees to 45 degrees, and also added that the rider would be tilting themselves forward at an angle of 45 degrees too. $\endgroup$
    – Rayyan Javed
    Commented Apr 24, 2022 at 10:19
  • 1
    $\begingroup$ @RayyanJaved so change the angles and redo the calculations. What else do you expect? $\endgroup$
    – Solar Mike
    Commented Apr 24, 2022 at 10:25
  • $\begingroup$ Will the rider tilting forward add any more torque than calculated? $\endgroup$
    – Rayyan Javed
    Commented Apr 24, 2022 at 11:40
  • $\begingroup$ When running at constant velocity the rider would have to tilt to that angle which puts the centre of gravity of rider and cycle over the point of contact of the wheel with the slope. This may not be 45°. $\endgroup$
    – Transistor
    Commented Apr 24, 2022 at 22:17
  • $\begingroup$ @Transistr, i mean the same thing. at constant speed the CG of the bike and rider must pass through the contract point. we need to have the height and posision of the saddle to be more exact. $\endgroup$
    – kamran
    Commented Apr 25, 2022 at 0:09
0
$\begingroup$

Swap your labels for handle and seat and add a hinge on the seat structure where it attaches to the frame of your contraption. That will be suffuce.

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Explaining the reasons for the suggested changes would improve this answer. $\endgroup$
    – Fred
    Commented Jul 1 at 6:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.