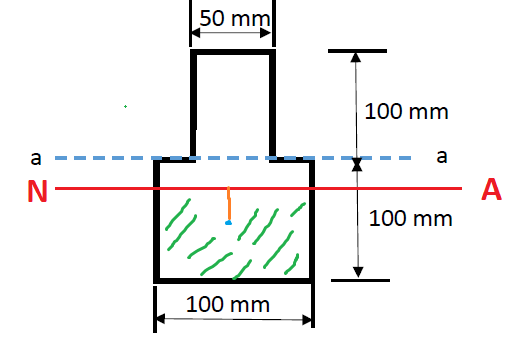
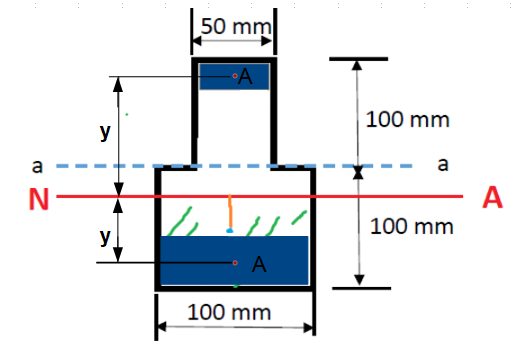
$\tau \:=\:\frac{VQ}{It\:}$ My confusion lies within $Q$ term. $Q = yA$ where $A$ is the cross-sectional area of the segment that is connected to the beam at the juncture where the shear flow is calculated, and $y$ is the distance from the neutral axis to the centroid of $A$.
So $y$ (orange) can be negative if the area is below the neutral axis right? What does that mean?
$\tau = \dfrac{V}{Ib}\int ydA = \dfrac{VQ}{Ib}$
The static/first moment of area, $Q = \int y dA$, is a property of a shape, which is always positive.
However, depending on the direction of shear force, $V$, the resulting shear stress can be negative, for which the negative sign indicates the location of a section cut along the span of the beam, and on which face of the section cut the shear stress is acting on/calculated. The sketch below shows where negative shear force occurs, and what negative shear stress means.
Just the direction you are measuring ie above the line is positive, below is negative.
If you put line A at the bottom of the item then all values would be positive.
The area moment,q, quation is
$$q=\int{\bar{y}}{da} $$
And is always positive because the distance, $\bar{y} \ $ is always positive.
If "y" was a signed value all beams sections would end up having zero shears because the lower half of the section with supposedly a negative shear would cancel the half with positive shear.
