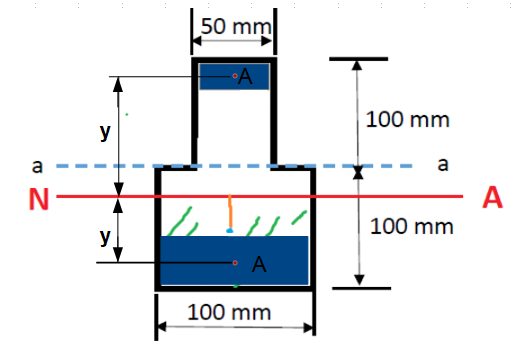
$\tau = \dfrac{V}{Ib}\int ydA = \dfrac{VQ}{Ib}$
The static/first moment of area, $Q = \int ydA$$Q = \int y dA$, is a property of a shape, and itwhich is always positive.
However, depending on the direction of shear force, $V$, the resulting shear stress can be negative, for which the negative sign indicates the location of a section cut along the span of the beam, and on which face of the section cut the shear stress is acting on/calculated. The sketch below shows where negative shear force occurs, and what negative shear stress means.