1
$\begingroup$

The maximum shear stress which we get from the equation: $\frac{VQ}{It}$ (for instance, the maximum shear stress at the neutral axis for a rectangular beam cross section, fixed at one end and tranverse loaded on the other can be thought of as: $\frac{3V}{2A}$), while the maximum shear stress which we get from the Mohr's Circle (i.e. $\frac{principal_{max} - principal_{min}}{2}$), so what is the difference between these two values? These both are maximum values of the shear as learned at the university level, so it is slightly confusing. Is former absolute while the latter is maximum?

Are these two shear stresses supposed to occur at the same location or different?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

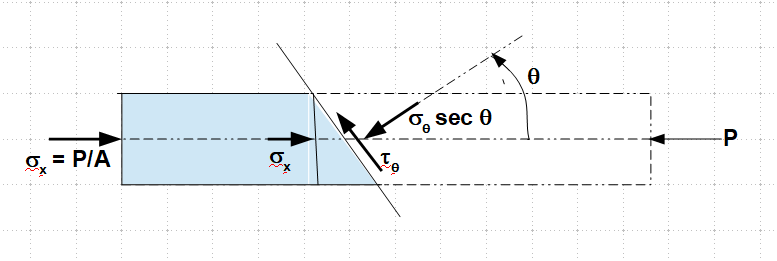
The Mohr circle is a tool that helps visualize the stress state in a location in the structure. The way I interpret it is that each point in the Mohr circle represents the stresses at a rotated coordinate system. (For the 2D case) in two of the orientations (the principal directions), there is no shear stress, while in the 45 degrees to those planes the maximum shear stress is observed (with non zero normal stresses in the general case).

The maximum shear stress in the bending of a rectangular beam is the maximum shear stress along the cross-section in a specific coordinate system which one axis is along the beam, another parallel to the transverse forces. For each point along the crosssection of the beam its possible to draw a Mohr's circle.

In the pure bending case of a symmetric beam (maybe in the asymetric also) the maximum shear stress of the neutral axis coincides with the maximum shear stress from the Mohr's circle. The reason is that there are no normal stresses.

At any other point above and below the neutral axis, the maximum shear stress obtained by the Mohr's circle is different to the shear stress calculated by the $\frac{VQ}{It}$ equation. (I would hazard a guess that the maximum shear stress as obtained by the Mohr's circle is maximum at the furthest points from the neutral axis and their magnitude is greater than $\frac{3V}{2A}$, because yielding and failure usually initiates at the edges of the cross-section.)

Improve this answer
$\endgroup$
6
  • $\begingroup$ So, while trying to calculate the maximum von misses stress at a cross section of beam subjected to bending for beam sizing, I will take into account the maximum bending stress. Now, to incorporate the maximum shear stress, should I take the one at the neutral axis or the max one along the cross section which results from the Mohr's circle? $\endgroup$
    – Rameez Ul Haq
    Commented Oct 21, 2021 at 18:54
  • $\begingroup$ I expect that the max von mises stress will occur at the edges. The reason is that the yielding and failure starts away from the edges (away from the neutral axis). $\endgroup$
    – NMech
    Commented Oct 21, 2021 at 20:17
  • $\begingroup$ @NMech, I'm trying to understand how transverse work. Is there transverse shear during pure bending? From the formula VQ/IT equation, there shouldn't since V = 0, backed up by looking at the derivation of the formula where transverse seems to originate from a difference in normal stresses causes by a difference in bending moment; m + dM. In the case of pure bending, there is no dM - so it would make sense that transverse would be zero at that point. It's hard to picture 'physically'. What do you think? $\endgroup$
    – Erik
    Commented Oct 22, 2021 at 0:21
  • $\begingroup$ Even at the middle section of the four point test (which is in pure bending) there is shear. It's not the same shear as the one induced by the transverse forces, but its due to the different magnitudes of stresses (and forces) on each layer of the beam. It is more akin to viscous shear, rather that the transverse shear of a pair of kitchen shears. $\endgroup$
    – NMech
    Commented Oct 22, 2021 at 7:19
  • $\begingroup$ @NMech, regarding the the second last paragraph you wrote in your answer, so it means that the non-zero principal stresses still exists at the neutral axis? Namely, double of the shear stress that we are seeing at the neutral axis? Is it correct? $\endgroup$
    – Rameez Ul Haq
    Commented Oct 22, 2021 at 19:34
1
$\begingroup$

$f = \dfrac {VQ}{Ib}$ is the "shear flow" in the flexural beam element caused by the applied load, and its intensity varies along the beam as the internal shear force $V$ varies.

enter image description here

  • The shear stress is always starting from zero at the free surface because shear occurs at the interface of sliding elements as shown below:

enter image description here

Mohr's Circle is used to find the stresses on an inclined plane of an axially loaded member as depicted below:

enter image description here

So, "No", the two types of shear stress (due to flexural and due to axial load) are completely different matters.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I just want to use the maximum possible value of the von-mises stress (which depends on the max value of bending stress and shear stress along a cross section) in order to size the beam. Now, what should be done? $\endgroup$
    – Rameez Ul Haq
    Commented Oct 21, 2021 at 19:13
  • $\begingroup$ Then the shear flow is the clear cut choice using the maximum internal shear force. $\endgroup$
    – r13
    Commented Oct 21, 2021 at 19:21
  • $\begingroup$ Please read the last paragraph that @NMech wrote in his answer. I guess he doesn't agree with what you say. $\endgroup$
    – Rameez Ul Haq
    Commented Oct 21, 2021 at 19:24
  • $\begingroup$ Why would the shear stress be zero at the free surface but not the normal stress? $\endgroup$
    – Rameez Ul Haq
    Commented Oct 21, 2021 at 19:41
  • 1
    $\begingroup$ I've deleted my previous comments as they are no longer valid after the revision of the answer. $\endgroup$
    – r13
    Commented Oct 22, 2021 at 22:57

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.