I want to find the exact net force exerted by the spring between pt. P and C, when it is compressed till point P with some mass, m attached to it and released (assuming spring goes to pt. C after its release) . The spring force till its mean position is given by formula "Kx" but there are spring inertial forces too (or due to its kinetic energy). Is there any method to find these inertial forces? ( assuming we only know k, m and x in this situation) 
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
Usually, in these types of problems, it is assumed the spring is massless. If we want to assign mass to the spring its analysis can become complicated because if we consider infinitesimally small sections of spring for vibration they are each having to deal with different K and different masses and the interaction between these infinitesimal parts before them and after them.
In simple cases where M>>m, it can be shown using energy methods it's reasonably ok to assume an effective mass of 1/3 of spring-mass and solve as follows. Wikipedia effective mass
M- mass of soli
m -mass of spring
$\alpha$ = acceleration
$\bar{x}= equilibriom\ on\ x\ where\ acceleratin=0$
$$ \left( \frac{m}{3}+M\right) \alpha=k\bar{x}$$
The equilibrium point $\bar{x}$ can be found,
$$\bar{x}=x-x_{equal} \ x_{equal}= \frac{1}{k}(1/2mg+Mg)$$
the period is
$$\tau=2\pi\ \left(\frac{M+m/3}{k} \right)^{1/2}$$
From here you can calculate your data.
$\begingroup$
$\endgroup$
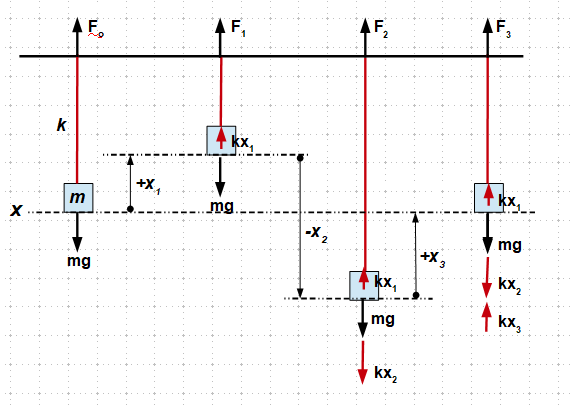
By definition, $F = mg = k\Delta x$, in which $mg$ is the gravity force, $k\Delta x$ is the force in the spring due to the change in physical position and represents the inertia force. The diagram above describes the force system at each stage.
Through equilibrium requirement, we can write the equation for each stage and identify the corresponding spring force.
$F_i = mg - k\Delta x$
$F_o = mg - kx_o = mg$, "$x_o = 0$" at initial stage.
$F_1 = mg - (kx_1)$
$F_2 = mg - kx_1 + kx_2 = mg - k(x_1 - x_2)$
$F_3 = mg - kx_1 + kx_2 - kx_3 = mg - k(x_1 - x_2 + x_3)$
In the equations above, $k\sum x_i$ is the net spring force, or inertia force, at the end of the respective stage. As expected, at the end of stage 3, $F_3 = mg = F_o$, which indicates zero spring force and the spring has returned to the original (balanced) position.
