0
$\begingroup$

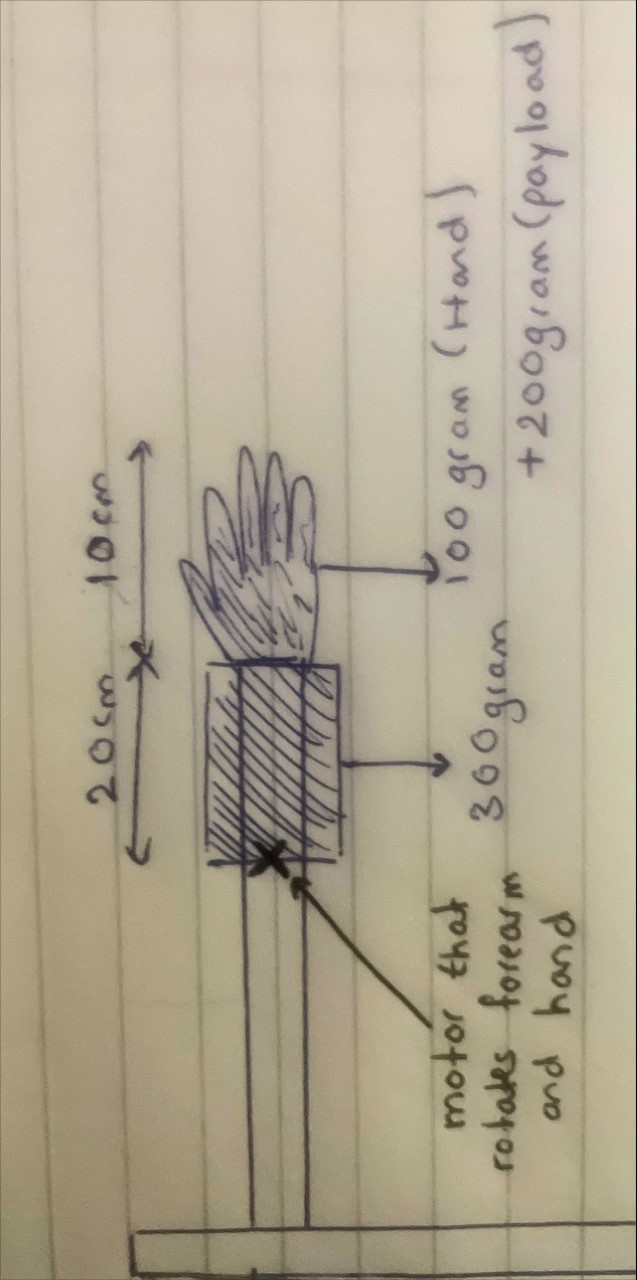
Diagram

Torque due to force of gravity:
-Payload: 0.2kg x 0.3m x 9.81 = 0.5886
-Hand: 0.1kg x 0.25m x 9.81 = 0.24525
-Forearm: 0.3kg x 0.1m x 9.81 = 0.2943

Torque due to force of gravity = 1.13Nm

What about torque due to angular acceleration?
I know it is Torque = Moment of inertia x angular acceleration, However, the motor will be rotating the hand and the forearm around the x-axis.

So, how would I calculate the torque for this motion?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

I assume you are trying to measure the torque needed to turn the arm horizontally to right or left.

If that's the case we calculate the angular moment, I as:

$$ I= \Sigma m_i r^2_i \ = \\ 0.2kg*0.3^2m+0.1kg*0.25^2m+0.3kg*0.1^2m \\=0.18+0.00625+0.003=0.027Nm^2$$

Not that we don't have g factor, and torque is:

$$T=\alpha*I$$

Improve this answer
$\endgroup$
15
  • $\begingroup$ thank you for your reply!! So do I ignore the torque due to the force of gravity? So I'm talking about this movement: makeagif.com/gif/pronation-and-supination-of-the-forearm-pKemMR $\endgroup$
    – user36991
    Commented Feb 17, 2022 at 18:30
  • $\begingroup$ no, if you needto lift you need the torque in X direction, if you need to turn you use the torque in z or y directio. if you dneed both you are dealing with 90 degre right hand moment and gyro precession. and it bocomes substantially more invonlved. $\endgroup$
    – kamran
    Commented Feb 17, 2022 at 18:40
  • $\begingroup$ Ah ok thank you!! So the above calculations from your answer will be all I need. So I will only need the torque due to angular acceleration if I'm understanding correctly? $\endgroup$
    – user36991
    Commented Feb 17, 2022 at 18:47
  • $\begingroup$ yes, as long as you measure the arm length from its hing and keep the motions separate you're fine. $\endgroup$
    – kamran
    Commented Feb 17, 2022 at 20:05
  • $\begingroup$ So, T=a*I, where I has been calculated to be 0.027Nm^2 from your calculations. To find a, I will need to specify how fast I want the arm to turn to a specific angle? Is that correct? $\endgroup$
    – user36991
    Commented Feb 17, 2022 at 20:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.