Can I dismiss wind loads for this solar panel configuration?

Question

I asked this question before in the Physics Stack Exchange, but it was closed because it was considered being about engineering. That is why I am asking it here.

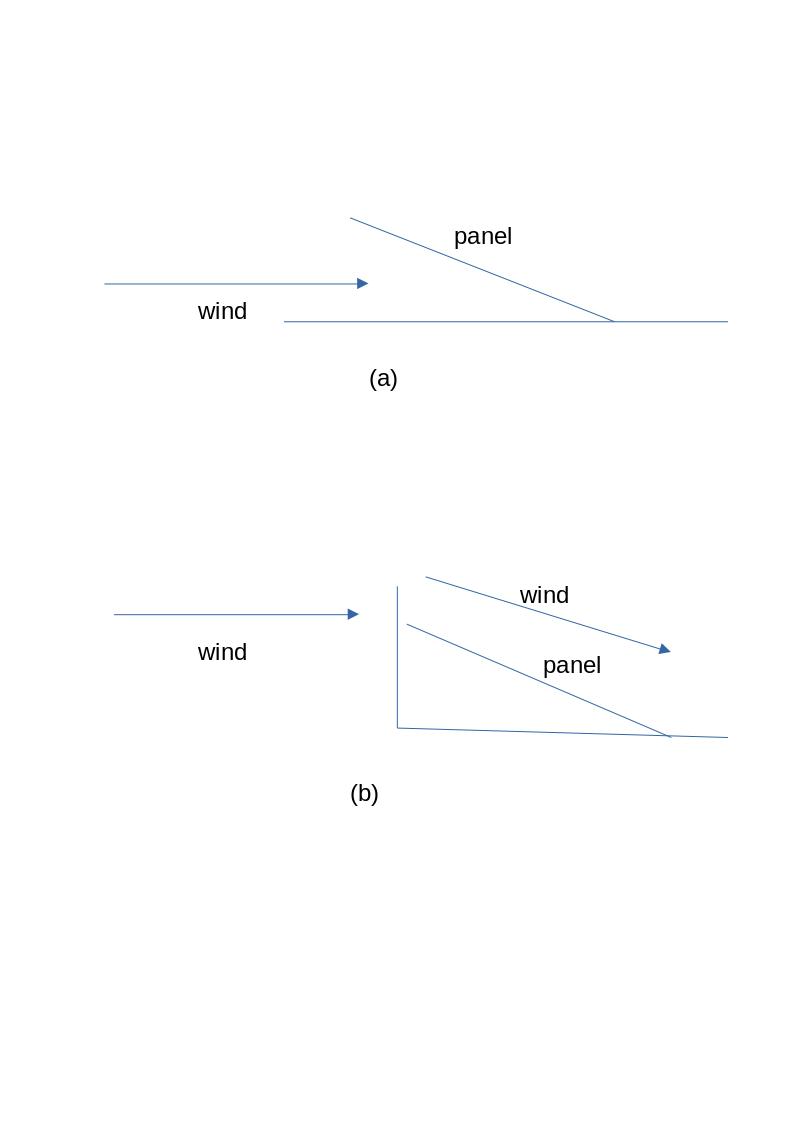
I am planning to install some solar panels on a flat floor. They must be inclined to optimizing the solar incidence and facilitates the cleaning of dust by the rain. In the fig (a) the wind pressure requires a strong attachment to the ground. My case is like fig (b): there is a wall that avoids that pressure.

But some people raises the Bernoulli argument: the wind above the panels lowers the pressure above, and there is an upward pressure anyway. For a wind velocity of $30 m/s$, and density of air of $1 kg/m^2$, $\frac{1}{2}\rho v^2 = \Delta p = 450 N/m^2$, what is four times the weight of the same area of the panel.

But I think that the Bernoulli principle is based only on energy conservation. Here, the energy of the air above the panel has nothing to do with the air below it, and there is no reason to expect lower pressure as a kind of compensation for higher kinetic energy.

Should I be confident that the wind is not relevant in this case, and the relevant forces on the panels are only their own weights and the Normal?

Answer 1

Normally one would use ASCE 7-10 Wind Load Calculations for leeward roofs.

$$p=qGCp−qi(GCpi) $$

Depending on the location, height, prevalent winds, zone, and some other factors you end up with a range of roughly 10 to 40psf wind pressure (uplift) on leeward side of sloped roofs, which is similar to this case.

Here is the link to Skyciv solved example.

Answer 2

In Europe, Eurocode EN1991-1-4:2004 describes wind loads. The nominal pressure (characeristic peak velocity pressure) for a wind with speed $v$ is calculated as

$$q = \frac{1}{2} \rho v^2$$

where:

• $\rho$ is the air density.
• $v$ is the velocity when taking into account orography and roughness.

Normal forces: pushing down (-) and pulling up (+)

Section 7 is devoted to pressure and force coefficients. It states net pressure coefficients should be determined for free standing wall and fences and monopitch canopies and monopitch roofs.

for 30 degrees inclination, you will get some downward pressure and some upward pressure (there are oscillations due to vorteces). The downward pressure coefficient at 1 m above ground is between -1.5 and -2.5 (depending on zone and wind direction), and the upward pressure is between 0.5 when the wind is blowing in the direction of configuration (b) in your post

Friction and tangential forces

Additionally, it defines friction coefficients for forces parallel to the surface.

Without any data, I can't really provide much further guidance because most of the data in the standard its in tabulated form.