0
$\begingroup$

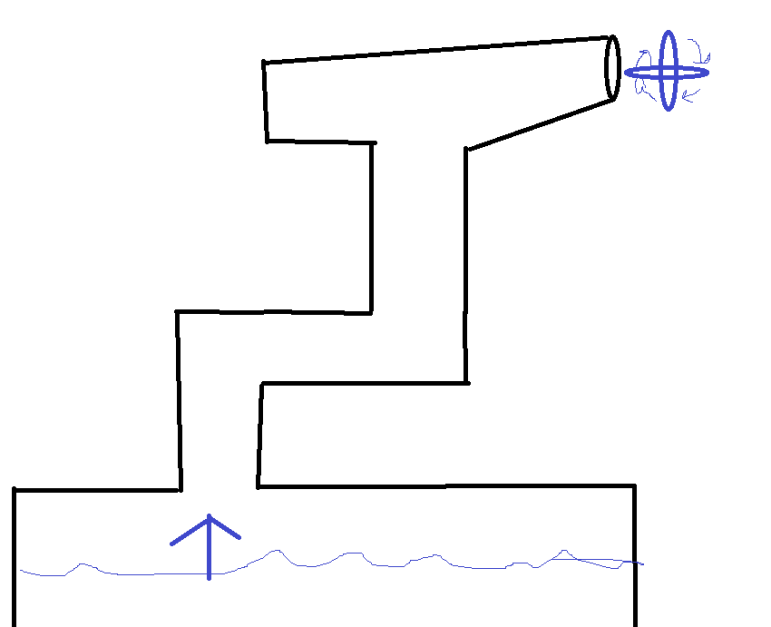
To better explain my question, I've included a schematic below. Let's assume that the water level rises. As the water rises in the wide chamber, it displaces the volume of air and essentially pushes the air through the duct and out through the exit to turn a wind turbine. According to the modified Bernoulli's Principle accounting for frictional losses, the more bends and curves present in the duct, the greater loss in pressure the air would exhibit. However, would that have an impact on the amount of energy that the turbine would be able to generate. Based on the wind power equation:

$$P=\frac{(pAV^{3})}{2}$$

the power output is only a function of velocity and area, and velocity according to the continuity equation only changes based on changes in cross-sectional area.

Continuity Equation: $A1V1=A2V2$

There aren't any pressure terms associated with power. So does that mean that the frictional losses from bends and the pressure loss determined by the Bernoulli's Principle don't have any bearing on the final power output? If that's the case, in theory, I can have hundreds of bends, duct reductions and expansions, but what would only matter would be my starting cross-sectional area (A1), starting velocity (v1), and final exit cross-sectional area. However, this doesn't seem true because the turbine can only convert energy that the air flow provides, and there is bound to be frictional losses to heat for every bend and curve that the air faces.

Water Rising at bottom, Air Spinning a Turbine on Top

Improve this question
$\endgroup$
3
  • $\begingroup$ Yes. Air Is compressible so when its flow is impeded, it gets compressed. This will have several effects, the biggest of which is that decompresses later. Because it decompresses at a later time the average velocity ends up less because it is the same mass moved out over a greater period of time. $\endgroup$
    – Abel
    Commented Sep 23, 2022 at 0:41
  • $\begingroup$ @Abel So does that mean the continuity equation doesn’t work for compressible fluids? In other words, you can think of some amount of air being stored in the duct as the air compresses. Is there an equation to determine the velocity after those bends similar to how we can determine pressure change using Bernoulli’s principle for incompressible fluids? $\endgroup$
    – ARJ
    Commented Sep 23, 2022 at 1:15
  • $\begingroup$ All bends and changes of section cause losses. $\endgroup$
    – Solar Mike
    Commented Sep 23, 2022 at 5:18

2 Answers 2

Reset to default
1
$\begingroup$

Bends and other changes of direction in airways cause shock losses. The more numerous the number of bends and the tighter the change of direction, the greater the shock loss, with each change.

When airflow is required to change direction, eddy currents will be initiated. These eddy currents consume the mechanical energy of the air and thus increase the resistance of the airway. This increase in resistance is defined as shock loss.

Improve this answer
$\endgroup$
8
  • $\begingroup$ It's quite intuitive to see that there would be kinetic and pressure losses because of bends in the airduct. Most of the equations I've come across tell me how to calculate pressure/head loss, but I don't see any equations on how to calculate energy loss. After all, the equation for a turbine is entirely dependent on velocity, and according to the continuity equation, friction is not accounted for. There must be something I'm missing in this that would allow me to account for the mechanical losses when calculating final power output. $\endgroup$
    – ARJ
    Commented Sep 23, 2022 at 2:04
  • $\begingroup$ ~ARJ - head loss is energy loss, as the pressure reduces, the energy available to do workdrops. $\endgroup$
    – Tiger Guy
    Commented Sep 23, 2022 at 16:15
  • $\begingroup$ @TigerGuy But how do I account for that in the wind turbine equation if that only has a variable velocity term? $\endgroup$
    – ARJ
    Commented Sep 24, 2022 at 0:33
  • $\begingroup$ @arj, the work you have to do is detemine the flow in the pipe. The resulting velocity is the output form that, which becomes an input to the turbine. The turbine isn't worried about the duct/pipe run. $\endgroup$
    – Tiger Guy
    Commented Sep 24, 2022 at 3:03
  • $\begingroup$ @TigerGuy So essentially, you can't just use the continuity equation (A1v1 = A2v2) for this? $\endgroup$
    – ARJ
    Commented Sep 24, 2022 at 4:37
0
$\begingroup$

The rule of thumb is that a 90 degree bend in an air duct will cause a pressure drop equivalent to that of a straight pipe whose length is about 10 to 15 times the duct diameter at the bend. So you do not want any unnecessary bends (or length!) in that air duct.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.