How to Find the Magnitude of Force and Direction

Question

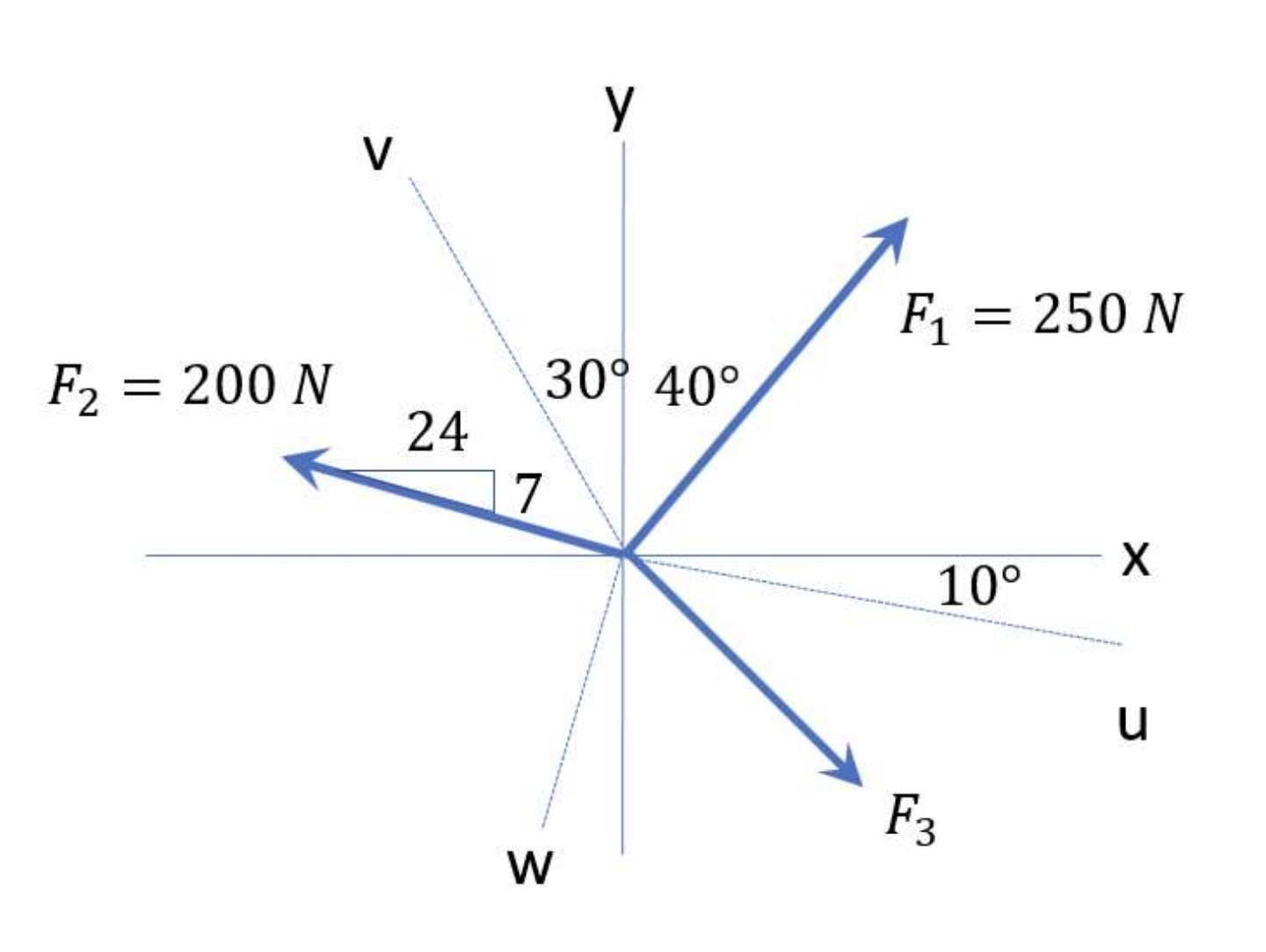
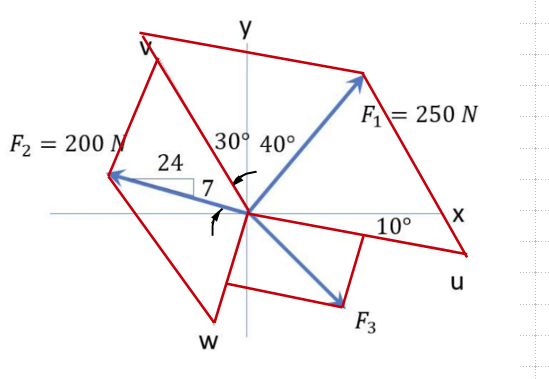
Give me some tips on how I can find the magnitude of $F_3$. The hint maybe is the angle of inclination of w axis such that the force components are equal along that axis, my instructor once told me. However, I can't still figure it out on how to solve for that angle.

The problem stated that $F_1$ is resolved in u and v axis, $F_2$ is resolved in v and w axis, while the $F_3$ is resolved in w and u axis. It is also stated in the problem that the components of $F_1$ and $F_2$ are equal along the v axis, the components of $F_2$ and $F_3$ are equal along the w axis, and the components of $F_1$ and $F_3$ are equal along the u axis.

Original problem

Answer 1

Assuming equilibrium, $\vec{F_1} + \vec{F_2} + \vec{F_3} = 0$ . We have: $$\vec{F_1} = \vec{{F_1}_u} + \vec{{F_1}_v}$$ $$\vec{F_2} = \vec{{F_2}_v} + \vec{{F_2}_w}$$ $$\vec{F_3} = \vec{{F_3}_w} + \vec{{F_3}_u}$$ And the problem states that: $$\color{red}{\vec{{F_1}_v}} = \color{red}{\vec{{F_2}_v}}$$ $$\color{blue}{\vec{{F_2}_w}} = \color{blue}{\vec{{F_3}_w}}$$ $$\color{green}{\vec{{F_3}_u}} = \color{green}{\vec{{F_1}_u}}$$ So we can rewrite the equilibrium condition as: $$\color{green}{\vec{{F_1}_u}} + \color{red}{\vec{{F_1}_v}} + \color{red}{\vec{{F_2}_v}} + \color{blue}{\vec{{F_2}_w}} + \color{blue}{\vec{{F_3}_w}} + \color{green}{\vec{{F_3}_u}} = 0$$ Or: $$\color{green}{\vec{{F_1}_u}} + \color{red}{\vec{{F_1}_v}} + \color{blue}{\vec{{F_2}_w}} = 0$$ Which leads to: $$\vec{{F_2}_w} = - \vec{F_1}$$ The rest of the solution is left as an exercise for the interested reader.

Answer 2

The forces are lying on planes defined by the space grid system, u, v & w. You need to work out the given angle between F2 and V-axis first, then from the given condition F1v = F2v, the angle between F1 and V-axis can be found. After that, everything is cleared up then.