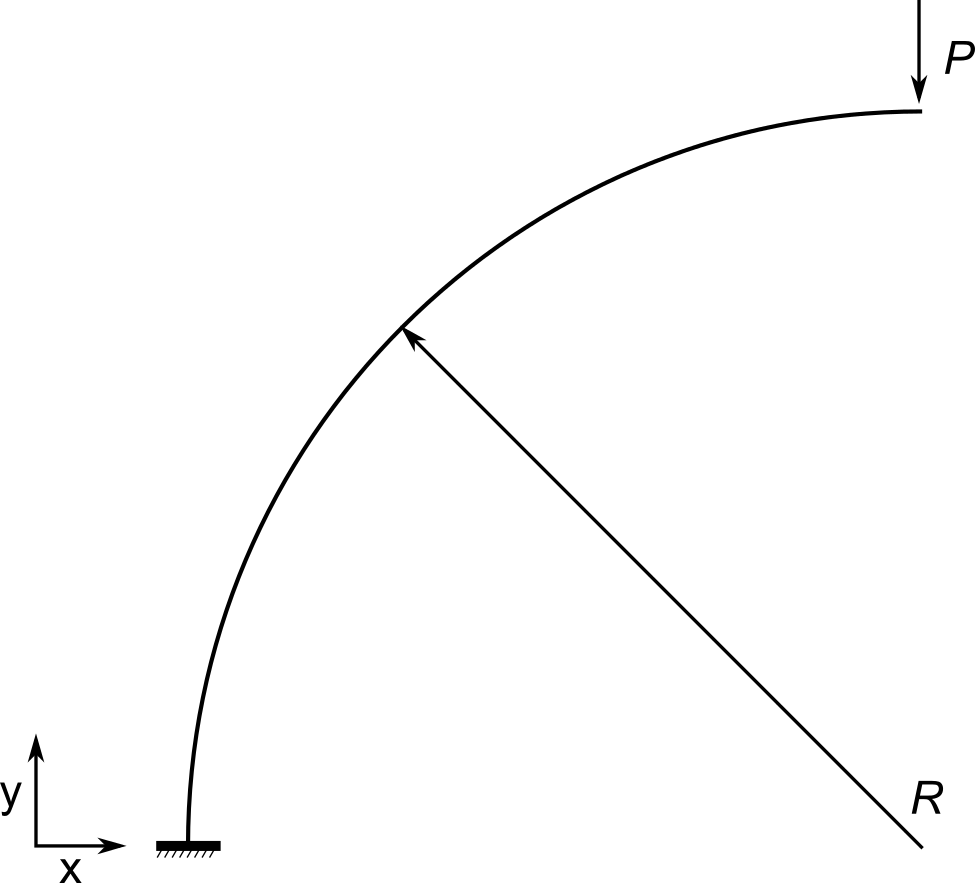
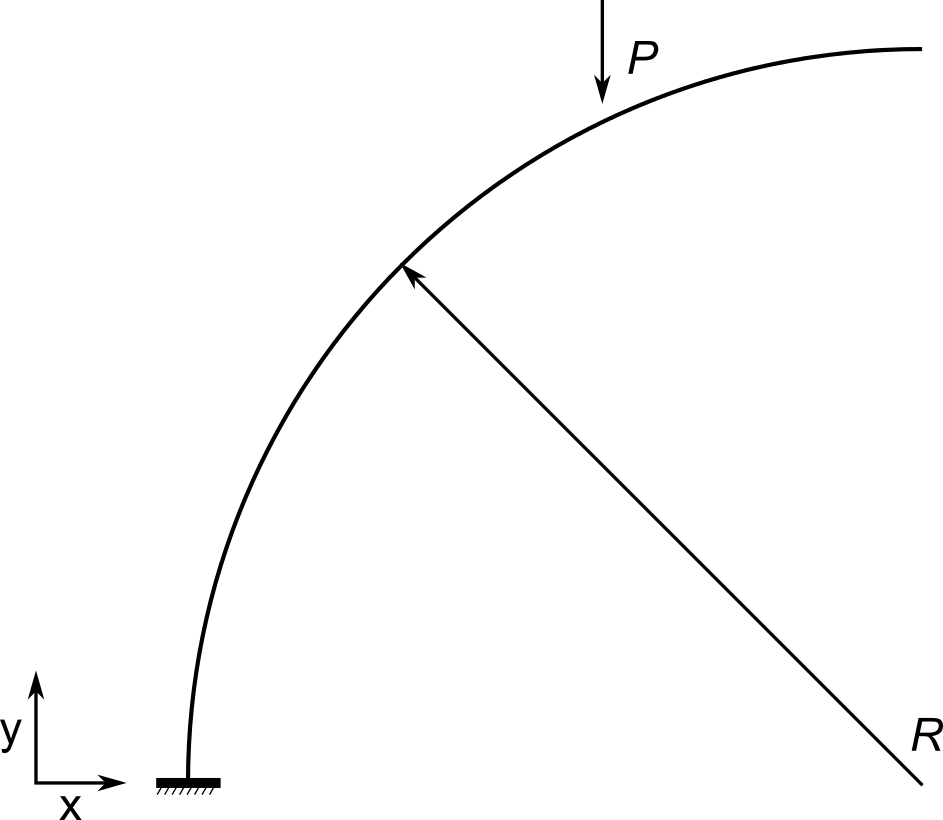
Suppose we have a curved beam of a rectangular cross-section $A$, Young's modulus $E$, and a constant radius of curvature $R$. We now expose the beam to a vertical point load of magnitude $P$ at its free end:
What I now want to know is where the beam will snap (I am assuming perfectly brittle material behaviour, and that the beam material is far stronger in compression than in tension). For this simple case, this is relatively straight forward (the beam will snap very close to the point of force application), but I want to know what happens when $P$ is shifted along the negative x-axis closer to the clamped end of the beam. For clarity:
Etc. pp.
Intuitively, as $P$ is shifted along the x-axis, the dominant type of stress will shift from bending to compression; if $P$ is applied very close to the clamped point, it's essentially a buckling problem, while it's similar to the bending of a straight cantilever beam when $P$ is applied at the free end. Now what happens in between, and how does this transition take place?
My gut feeling is that as $P$ is shifted (for clarity, I don't mean to imply any dynamic loading here), the point at which the beam will snap will increasingly move away from the point of force application, so if we were to start loading the beam at its far end until it snaps, then move the point of force application to the new far end and so on, we would end with snapped fragments of the beam which increase in length. However, I have thus far failed to come up with a sensible model that would allow me to predict whether this is what is actually happening, and would be grateful for any hint.