0
$\begingroup$

I was recently reading some of Leonardo da Vinci's notes on architecture. One passage on arches states:

An Experiment to show that a weight placed on an arch does not discharge itself entirely on its columns; on the contrary the greater the weight placed on the arches, the less the arch transmits the weight to the columns. The experiment is the following. Let a man be placed on a steel yard in the middle of the shaft of a well, then let him spread out his hands and feet between the walls of the well, and you will see him weigh much less on the steel yard; give him a weight on the shoulders, you will see by experiment, that the greater the weight you give him the greater effort he will make in spreading his arms and legs, and in pressing against the wall and the less weight will be thrown on the steel yard.

There are two parts to my question:

  1. What are the physical principals behind Leonardo's statements on the distribution of an arch's load to its columns?
  2. How does his experiment demonstrate these principals?

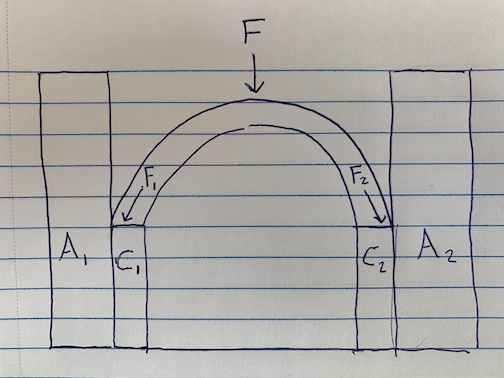
On 1, I interpret his scenario as in the below diagram. There is some arch which is supported at is ends by two columns $C_1$ and $C_2$. There are additionally two abutments $A_1$ and $A_2$ to the sides of the arch to counter the arch's horizontal thrust. A downward force $F$ is applied to the apex, which is directed through internal compression forces to the arch's ends, where we see $F_1$ being applied to $A_1$ and $C_1$ (and analogously for the other side). My understanding is that no matter how large $F$ is, the vertical components of $F_1$ and $F_2$ must both be $F/2$ in order to satisfy the static equilibrium constraints.

Then, how do I reconcile this analysis with Leonardo's claim "the greater the weight placed on the arches, the less the arch transmits the weight to the columns"? Is Leonardo assuming a different setup than I have? Or is one of us wrong?

Arch Diagram

Improve this question
$\endgroup$
5
  • $\begingroup$ I think that if everything was frictionless you might be right but would it not be the case that the larger F might cause F1 and F2 to 'bite' into A1 and A2 to the extent that some of the vertical load is transferred into them? And did he mean 'the less proportion the arch transmits to the columns'? $\endgroup$
    – Transistor
    Commented Apr 12, 2020 at 20:15
  • $\begingroup$ Yeah, great point! Leonardo's claims almost always come from real-world experiments (in which there will of course be friction). I see how adding friction could transfer some of the vertical load to A1 and A2, and originally I was trying to use this to understand how the absolute vertical forces on C1 and C2 could decrease as F increases. But, now that you mention it, I agree that he must have been talking about the proportional forces relative to F. How would we quantify how much the proportional force changes with increasing F? Would we need analyze the torque induced at the ends? $\endgroup$
    – radkins
    Commented Apr 12, 2020 at 21:24
  • $\begingroup$ The one part that makes me think he still might be talking about the absolute vertical force, is in his proposed experiment, where he mentions a scale reading out a smaller number when adding weight to the system. But maybe it's just the phrasing. $\endgroup$
    – radkins
    Commented Apr 12, 2020 at 21:39
  • $\begingroup$ I haven't a clue, really. I'm an electrical engineer! $\endgroup$
    – Transistor
    Commented Apr 12, 2020 at 22:02
  • $\begingroup$ Perhaps look at how arches looked like from his time...notice that they don’t look like how you drew it, and the components...on top of that don’t forget normal and frictional forces. $\endgroup$
    – DrMrstheMonarch
    Commented Apr 12, 2020 at 23:01

1 Answer 1

Reset to default
0
$\begingroup$

I think he may have been thinking two things,

  • Friction.

  • Large deformations

If we follow him in his path of organizing the gradual transfer of vertical reactions to tangential friction forces from the wall taking most the vertical load, he must have been thinking of a gradual change in the mechanism of the arch from a regular arch to an arch with a plastic hinge formed on the apex.

That would make it possible for the vertical forces to be supported entirely by a triangular force mechanism with the external force on the top and a cracked or flexed apex and to friction forces on the two lateral bottom sides as the tangential components of the friction force.

Let's assume at the ultimate case when the apex is fully cracked or turned into a plastic hinge, a triangular truss substituting the arch with the two base angles at 45 degrees and the load P and the friction factor of 0.8 just to illustrate his idea.

The horizontal reaction at the walls

$F_h= P/2 \quad \text{and the vertical component of friction, Fr} \quad F_r= 0.8P$

So for an angle of 45 degrees, the wall takes 80% of the load.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you! It's the deformations that I left out while I've been running my calculations. Without deformations, it seemed that the frictional force parallel to the walls was always in the same proportion independent of the load. But it makes sense that with the tendency of the arch to develop a plastic hinge at the apex that the proportion could change with the applied pressure. $\endgroup$
    – radkins
    Commented Apr 13, 2020 at 18:27
  • $\begingroup$ Now my question is: what's the math that describes the deformation as as a function of the vertical load? It seems that the deformations would limit the force distribution toward that of a triangular truss from what you say? Anyway, maybe it's worthy of a separate question :) $\endgroup$
    – radkins
    Commented Apr 13, 2020 at 18:27
  • $\begingroup$ Deformation calculations in an arch no matter how complex the shape is is possible by either FEM software or analytical methods, even by using engineering handbook formulas. But masonry is very brittle, like in concrete default assumption is a cracked section. So most of the great masons of the old times relied on experience and well kept secrets of the trade. I beleive day used a mix of aggregate with lime and clay as mortar. One could start by assuming all the mortar joints cracked. $\endgroup$
    – kamran
    Commented Apr 13, 2020 at 18:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.