Probability of structural failure

Question

In structural engineering we have to accept the fact that you can never be exactly sure how much load a structural member can resist, that the load carrying capability of an element is not one exact value but distributed with some probability distribution function (quality of laid concrete, for example, is quite dependent on many conditions on the worksite, such as the workers skill, etc.). The same goes for structural loads. Therefore we cannot say with absolute certainty whether some structural is stable, we can only calculate certain probabilities.

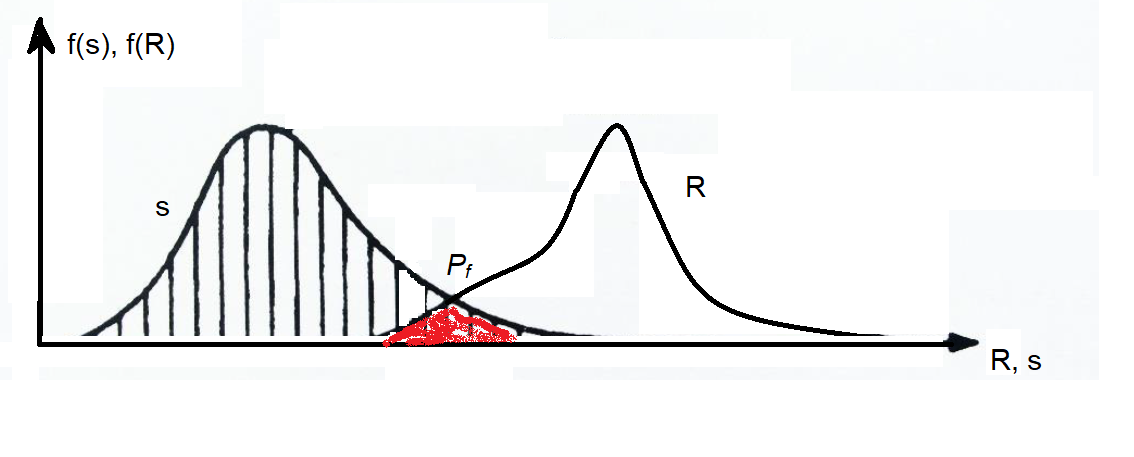
In the image below, the left curve represents the probability density of the possible loads, and the one of the right represents the probability density of the load carrying capacity of some structural element. The y-axis is the probability density, and the x-axis is the structural load. The red area where the curves overlap is a range of loads where structural failure is possible; before that range there is "zero" possibility of capacity being that low, therefore the capacity must be higher than any possible load (failure is defined as a scenario where the load is greater than the capacity). Beyond this range the load has "zero" possibility of being higher than the capacity therefore no chance of failure.

The actual probability of failure can be calculated as follows, according to Wikipedia:

$${\displaystyle P_{f}=\int _{0}^{\infty }F_{R}(s)f_{s}(s)\,ds\qquad \mathrm {} }$$

where $F_R(s)$ is the probability the cumulative distribution function of resistance/capacity (R) and $f_s(s)$ is the probability density of load (S).

I have hard time understanding why the above formula gives the probability of failure. This is supposed to give the probability of the load being higher than the capacity/resistance, but I just can't wrap my head around how that works. Could somebody explain this to me in more detail? I am familiar with probability distribution functions and cumulative distribution functions, but I don't understand what's happening here. Why do you take the cumulative function of the load resistance but the probability density function of the load?

Answer 1

Like mentioned by @mart above, I believe there is something wrong with the curve of $F_R(s)$. The text states cumulative function so it should have been something like.

The way I would explain the Probability of failure is the following. There is failure if the load is greater (S) than the structural resistance capacity (R). So you want to iterate over all possible loads (thus the probability density of a load s).

Assuming you get a structural load s of S e.g. 1 [kN]. If the structure has R greater than S there is no failure. So you are interested for all the values, below S. That is the probability of $$P(R\le S) = \int_{0}^Sf_R(s)ds = CDF_R(s)=F_R(s)$$.

Since the two probabilities (A: load at S), (B: resistance R is less or equal to load S) are statistically independent, their probability of occuring simultaneously is given by:

$$P(A\cap B) =P(A)\cdot P(B)= f_s(s)\cdot F_R(s)$$

Then all you need to do is integrate over the entire range of $s$. That gets you:

$$P_{f}=\int _{0}^{\infty }F_{R}(s)f_{s}(s)\,ds$$

TL;DR I think the Wikipedia entry is incorrect and confuses.

Answer 2

Suppose the resistance capacity has a probability density function between 1kN and 2kN and is 0 everywhere else.

What happens if you apply a load of 1,000kN? Answer: the structure is guaranteed to fail.

So you need to use the cumulative density function for the resistance. The cumulative density is 1.0 for any load greater than 2kN.

IF you use the probability density for the resistance instead of the cumulative density, you are saying that the structure might fail for loads between 1kN and 2kN, but it will not fail for any load greater than 2kN, which is obviously wrong.