-1
$\begingroup$

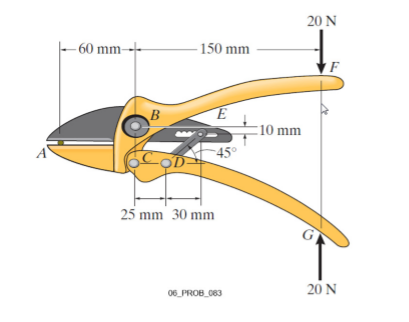
I am trying to find the forces in hinches $B$, $C$ and $D$. The exact solution is not important for me. I introduced the following internal forces to solve the problem: $A_y$ in $A$, $B_x$ and $B_y$ in B, $C_x$ and $C_y$ in $C$, a force $F_{DE}$ in the direction $\vec{DE}$. But I think I am missing something. I have currently 6 unknowns. But I need equilibrium for the subsets ABE (grey), ABF (yellow) and CDG (yellow). Are there any other internal forces / torques I should introduce?

Pruning shears enter image description here

First subsystem:

$C_x + F_{DE} \cos(45) = 0$

$C_y + F_{DE} \sin(45) + 20= 0$

$F_{DE} \sin(45) \times 25 + 20 \times 150= 0$

Second subsystem:

$B_x - C_x = 0$

$B_y - C_y - A_y - 20= 0$

$-F \times 150 + A_y \times 60 - C_x \times 30 = 0$

Third subsystem:

$-B_x - F_{DE} \cos(45) = 0$

$A_y -B_y - F_{DE} \sin(45)= 0$

$-A_y \times 60+ F_{DE} \sin(45) \times 55 = 0$

I have 9 equations but only 6 unknowns. Are there any linear interdependencies between the equations?

Improve this question
$\endgroup$
6
  • $\begingroup$ Are tou applying 20N or 40N? Try separating this compound lever system into 3 separate systems which might simplify things. $\endgroup$
    – Solar Mike
    Commented Jan 15, 2020 at 6:58
  • $\begingroup$ Two times 20N are applied. $\endgroup$
    – Wim Nevelsteen
    Commented Jan 15, 2020 at 11:40
  • $\begingroup$ So, how has the separation gone? $\endgroup$
    – Solar Mike
    Commented Jan 15, 2020 at 11:43
  • $\begingroup$ On the second picture. I split it up in 3 subsystems. For every system on that picture I have 3 equations. That means 9 equations. But I have only 6 unknowns. $\endgroup$
    – Wim Nevelsteen
    Commented Jan 15, 2020 at 11:49
  • $\begingroup$ Later today I can add the equations. $\endgroup$
    – Wim Nevelsteen
    Commented Jan 15, 2020 at 11:50

1 Answer 1

Reset to default
2
$\begingroup$

Writing down a system of equations for all the unknowns is generally not the best strategy. Instead, look for strategic opportunities to apply one equation of equilibrium, to one component, where it will involve one unknown.

A good starting point is often to sum moments about some location that is in line with one or more of your unknowns. That way, those unknowns are not part of the equation.

For example, if you look at part CDE, and you take the moment about C, you only have to deal with force DE as an unknown. With one equation, you solve for one unknown, force DE.

Then, stay with part CDE. Sum horizontal forces, and that will give you the horizontal component of the force at C. Sum vertical forces and that will give you the vertical component of the force at C.

Now you know all the forces that touch part CDE, so move on to different part.

Again, use the strategy of summing moments at a location that is in line with one or more unknowns.

Look at part ABF, and sum moments about B. You know the forces at point C, so the only unknown will be the force at point A.

Keep going, and you will get all the unknowns.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you. It was in my mind that I had tot write down all 3 equations for all 3 subsystems. I see that they are redundant. Thanks. $\endgroup$
    – Wim Nevelsteen
    Commented Jan 16, 2020 at 2:11
  • $\begingroup$ @WimNevelsteen: If this has answered your question, please click the checkmark to the top-left of the answer to mark it as accepted. $\endgroup$
    – Wasabi
    Commented Jan 18, 2020 at 1:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.