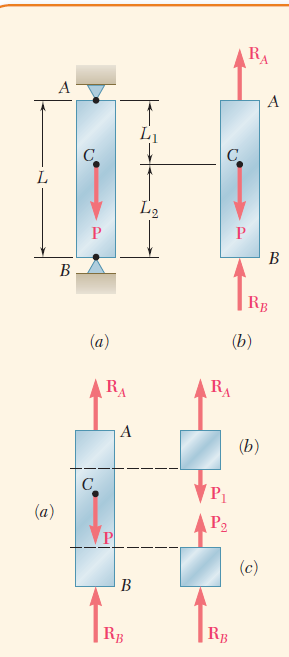
I thought the reaction force should go downward at B but textbook says upward. If it goes up, doesn't that mean its a tensile force? I don't think there's any tension in the system. Also, when you cut the bar into pieces, for part c (that's the lower part), shouldn't it be internal force and load P as well, cause it's going downward?
A metal bar will deflect under its weight by
$$\Delta L= \frac{\int_{0}^{L}A\rho. d L}{AE}$$
A area of bar
L length off bar
E modulus of elasticity
$\rho$ specific density
Therefore if the distance between the top and bottom supports is not $L- \Delta L \ $ say its distance is $L$The top support will have an upward reaction.
Not exactly sure what the question is here, but I'll take a shot at clarifying.
We have a pin at points A and B. The pin allows for rotation, but will resist translation. So, if the force P "tries" to move the block downward, then the pins at A and B will resist this force by opposing it. In order to oppose the downward force, $R_A$ and $R_B$ must point upwards.
In this case, $R_A$ is a tensile force, while $R_B$ is a compressive force. $R_A$ will try to "stretch" the top portion of the block, because force $P$ is trying to move the block away from pin A. $R_B$ will "squish" the bottom portion of the block, because force $P$ is trying to move the block towards pin B. However, because the block is pinned at both ends, it won't actually have a displacement; that is, its length will be the same as if there were no force:
$$\delta_A - \delta_B = 0 \implies \delta_A = \delta_B$$
About your last point--I think the diagram shows something different from what you might be thinking. The bottom half of the images displays portions of the block not including the portion affected by force $P$. So, $P_1$ and $P_2$ are necessarily internal forces. Their magnitude is changed by the presence of force $P$, but that doesn't mean you have to label it $P_1 + P$, as I think you might be implying.
