Stress in a L-shaped Beam due to Thermal Expansion

Question

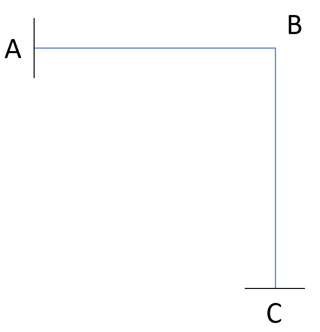
A L-shaped frame $ABC$ (right angle at $B$) consisting of beams $AB$ and $BC$ are fixed at ends $A$ and $C$ to rigid walls. Find the tensile stress at $A$ when the temperature of the frame is raised by $\Delta T$. Properties: length of $AB = BC = L$, flexural rigidity $EI$, coef. of thermal expansion $\alpha$ and circular cross-section with diameter $d$. Also, assume that the right angle at $B$ is maintained during deflection.

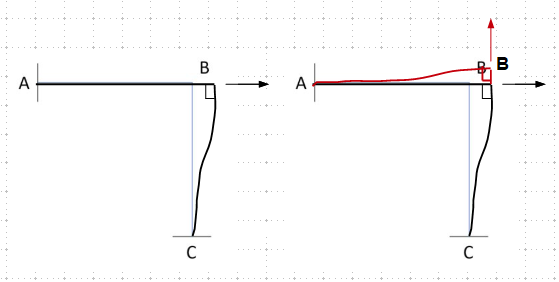
Initial thoughts: due to $\Delta T$, beam $AB$ will cause beam $BC$ to deflect at $B$ rightwards ($\rightarrow$), and $BC$ will cause $AB$ to deflect at $B$ upwards ($\uparrow$).

1. Since the right-angle remains after the temperature change, the L-shape of the bar is also maintained.
2. The upward deflection does not influence the tensile stress at $A$.
3. The thermal expansion of $AB$ ($\delta_T = \alpha \Delta T L$) is counteracted by a reaction force from $BC$, which has the same magnitude of its 'virtual' deflection ($\delta$).

Which leads to

$$\alpha \Delta T L - \delta = 0$$

This is equal to zero because the shape of the beams are maintained.

From the integral method for deflection (or energy method),

$$\delta = \frac{F}{A} \frac{L^3 A}{3} = \sigma \frac{L^3 A}{3}$$

Substituting $\delta$ and solving for $\sigma$

$$\sigma = \frac{3\alpha \Delta T}{AL^2}$$

I am worried that my assumptions are wrong and I cannot think of solving it other way. Could someone check these assumptions? Is there a way that I could do it differently to check the answer?

Answer 1

The frame will be deformed as shown below - a distorted L if so claimed.

Answer 2

you are right your method is not correct because the stiffness of the two members are not rqual.

the stifness of

$K_{Ab}= EA/L $

but stiffnes of the cantilever BC is.

$K_{BC}= 3EI/L^3$

The thermal extention of ab will be countered by a force $F = K_{BC} \delta AB$

Final delta, note we use F/2 because as AB recoils the F decreases to zero.

$\delta_{final}= F/2* K_{AB}$

Edit

After some comments The stiffness of the two members is not identical! because AB is loaded axially and extends longitudinally but BC is a cantilever beam both loaded transversely and deflecting by rotation not by elongation. Also even though there is a rule for springs in series, $\frac{1}{K_{final}}= \frac{1}{K_1}+\frac{1}{K_2}$ I don't know if it applies here because usually, the load is the same in a series spring system, here it is not.