How can I justify a consistent sign convention for statically indeterminate problems?

Question

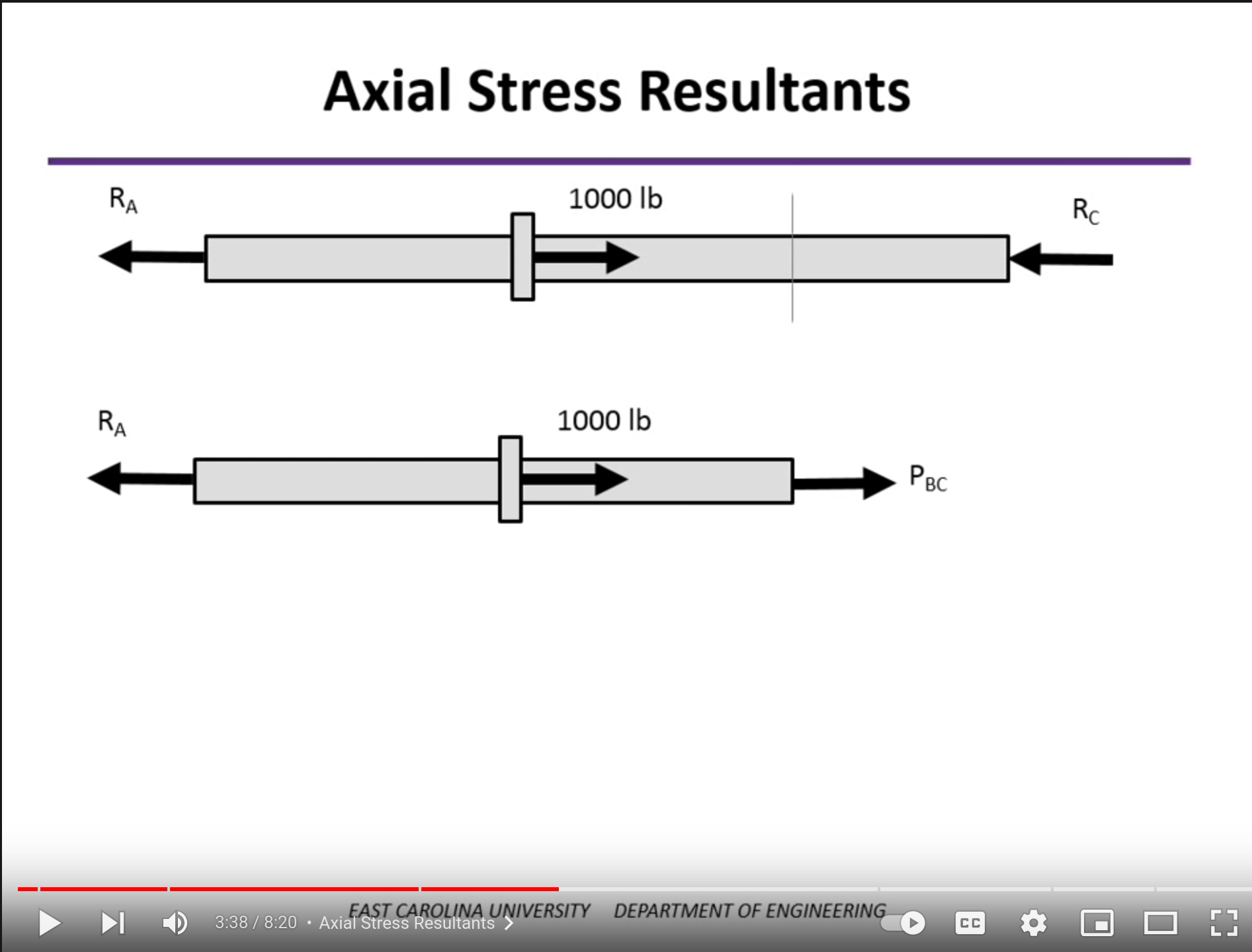
I am watching the below video on statically indeterminate axial loading. When he makes a cut on the left half, I understand why he draws the internal force acting to the right, because if he drew it acting to the left then the cut would be accelerating to the left. But when he makes the cut in the right hand side, things are not so simple. He states, "I always draw the internal force as if it were in tension and let the sign take care of itself" even though it is clearly in compression. Here is a screenshot of that part. He is obtaining an expression for PBC.

Working the rest of the problem he finds reaction A as 600 lb. The problem comes if we had drawn the internal force in the opposite direction. If we had done that and solved as he did we would have found

$$R_A\cdot (12''-18'') + 18000\cdot lb\cdot in = 0 \rightarrow R_A = \frac{-18000}{-6} = 3000 lb$$

So, it is more than just letting the signs take care of themselves. There is a real difference!

In sum:

How do I know what direction to put the internal force for a generic problem?

https://www.youtube.com/watch?v=d3NElXJGwdc

Answer 1

(Without knowing the details of your calculations - so I am making pretty heavy inferences), the usual mistake is that the compatibility equation would be different.

In the video the assumption is that the internal forces (both $P_{AB}$, and $P_{BC}$) have a direction that causes extension. That is why the compatibility equation is:

$$\delta_{AB} + \delta_{BC} =0 $$

I.e. the added extension that both forces cause needs to be zero. This by extension mean that if one of the internal forces is in tension the other will be in compression.

---

if you draw only the internal reaction in the section BC as compressive $P_{BC}$, then you need to take account for that into the compatibility equation. I.e.

$$\delta_{AB} \color{red}{\mathbf{-}}\delta_{BC} =0 $$

Then the $P_{BC}$ inverted formula (i.e. $P_{BC}= -P_A + 1000$) will yield the same result.

---

Note this is about the stressed word only in the previous section:. If both $P_{AB}$ and $P_{BC}$ were drawn as compressive, then you'd need to use the $-\delta_{AB} - \delta_{BC} =0$ (which is identical to $\delta_{AB} + \delta_{BC} =0$)

---

Please note that this is only my assumption to what went wrong from extrapolating from your question and from my past experiences explaining to other people. In order to help you more, you'd need to provide more explicit details of your calculation.