I'm stuck trying to find the resultant of the force system. Here it is said to find the angle θ the resultant manes with the horizontal. But θ here is narked relative to F₂. How do I find the resultant and θ
3 Answers
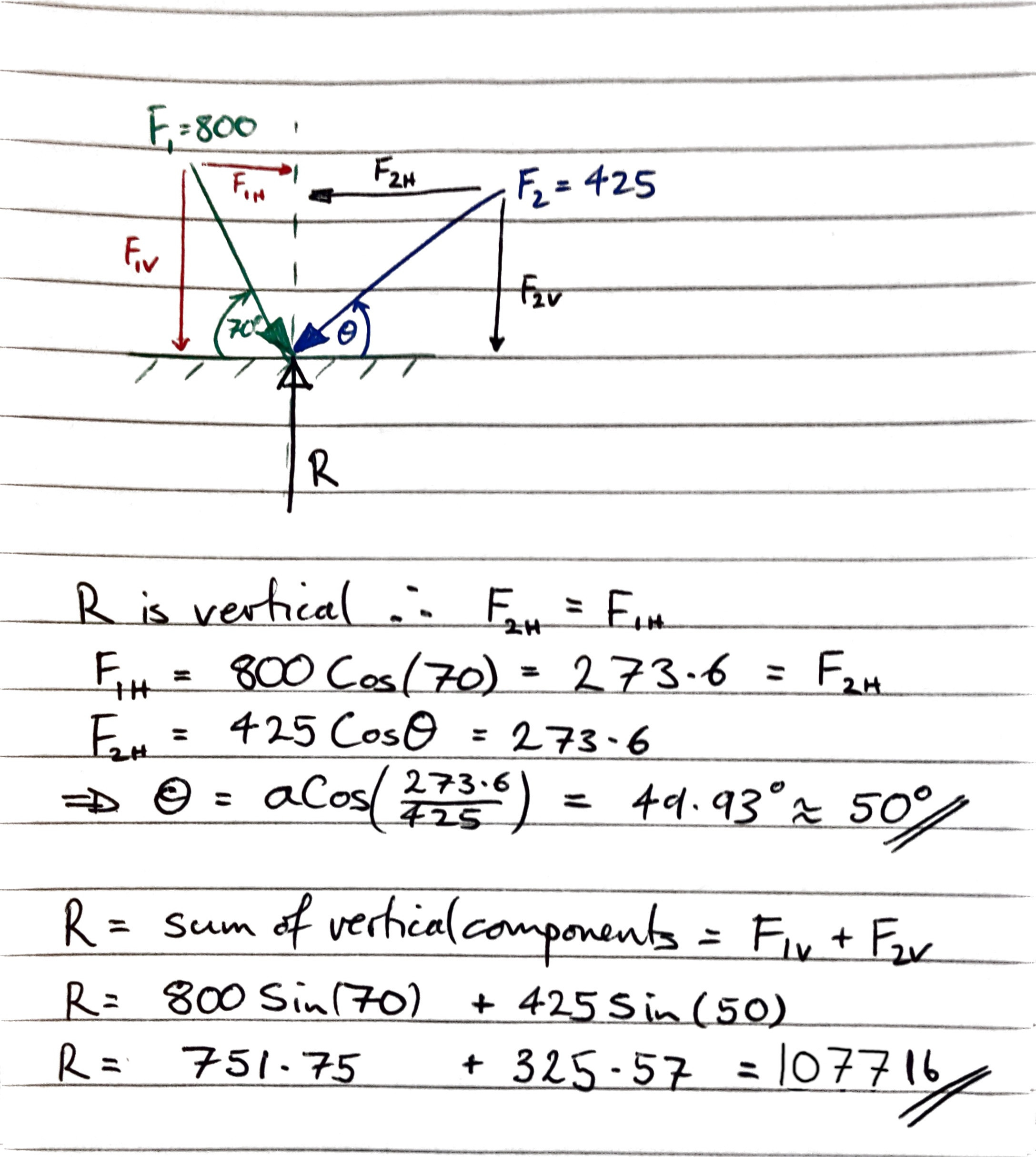
In order for the Resultant force to be vertical you only need the sum of the horizontal components of $F_1$ and $F_2$ to be zero
$$F_{1x} - F_{2x} =0 $$ $$F_{1}\cos(70) - F_{2}\cos\theta =0 $$
$$\cos\theta=\frac{F_{1}}{F_{2}}\cos(70) - F_{2} $$
$$\theta=\arccos\left(\frac{F_{1}}{F_{2}}\cos(70) - F_{2}\right) $$
if you substitute you should get $$\theta=50[deg]$$
And the resultant R should be :
$$F_{1y} + F_{2y} =R $$ $$F_{1}\sin(70)+ F_{2}\sin(50) =R $$ $$R \approx 1077 [lb]$$
-
$\begingroup$ What theory guides add the forces & equating them to zero, since it's not stated that the forces are in equilibrium $\endgroup$ Commented Mar 3, 2021 at 12:04
-
2$\begingroup$ It is stated that the resultant of the two forces is vertical, which indicates that the horizontal portion of the forces is in equilibrium. $\endgroup$ Commented Mar 3, 2021 at 12:05
-
$\begingroup$ The question is about the resultant of the forces. If you are thinking about the reaction from the bracket, then this will be determined by the load. $\endgroup$– NMechCommented Mar 3, 2021 at 12:08
I don't have time to type this up nicely - thought it would be quicker to write out by hand but in hindsight my handwriting is terrible. Let me know if you can't read anything!
-
$\begingroup$ There seem to be an error in the resultant R $\endgroup$ Commented Mar 3, 2021 at 12:12
-
$\begingroup$ You're totally right - I must have mashed the buttons on my calculator weirdly. Hopefully you can work out the correct value from the addition before it! $\endgroup$ Commented Mar 3, 2021 at 12:19
-
$\begingroup$ I have done a quick photoshop update to correct the final value or R! $\endgroup$ Commented Mar 3, 2021 at 12:22
A problem with meaningful solution must have a key with enough conditions/constraints, that make it soluble. As a problem solver, we need to find/identify the key and the associated conditions/constraints. Sometimes work a problem in the backward manner can be helpful. The steps are as follows:
- Expand the unknown (R = ?) to a more detailed expression. That is, in an orthogonal grid system,
R = (Fx^2 + Fy^2)^1/2; in which, Fx = F1cos70 + F2cos(\theta), and Fy = F1sin70 + F2sin(\theta)
- Identify/find the key. In this problem, the angle (\theta) is the key, and the statement that says "Determine the angle (\theta) which makes the resultant (R) of the two forces vertical, is the furnished condition/restraint. Laterally the statement tells us to find the angle (\theta) that will result in: sum Fx (horizontal resultant force) = 0, so the relationship can be expressed as,
Fx = F1cos70 + F2cos(\theta) = 0
Now you have the key to solve the problem. Hope this helps.