How to replace these two forces with one force?

Question

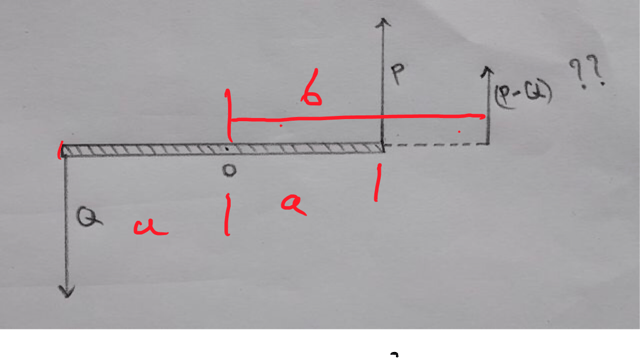
Here, $P>Q$. $O$ is the center of mass of the rigid and uniform bar/stick.

As $P>Q$, the resultant is situated to the right of $\vec{P}$ and is parallel to $\vec{P}$. The magnitude of the resultant is $P-Q$.

To convince you that the figure is correct, I'll do some math to prove it.

Let us obtain the sum of torques about the center of mass,

$$(P-Q)b=Pa+Qa$$

$$b=\frac{P+Q}{P-Q}a$$

$$b=fa\ \left[\text{Let $f=\frac{P+Q}{P-Q}$}\right]$$

As $P>Q$, $f>1$, and $b>a$. So, the correct figure will be,

I hope you're satisfied that the figure is correct.

My comments:

Is it possible to replace $\vec{P}$ and $\vec{Q}$ with a single force? I mean practically, not theoretically. From the figure, we can see that the resultant force is outside the bar. In other words, $\vec{P}$ and $\vec{Q}$ can be replaced by a force of magnitude $P-Q$, which will act outside the bar. This may be possible theoretically; however, this is not possible practically as the resultant force will be acting on literally nothing as it is outside the bar. Therefore, I conclude that it is impossible to replace $\vec{P}$ and $\vec{Q}$ with a single force practically. Theoretically, it is possible, but practically, no.

My question:

1. Can $\vec{P}$ and $\vec{Q}$ be replaced by a single force? Is my conclusion correct?

---

These may help you to answer this question:

1. Comment by @Ivan
2. Answer by @Farcher

---

This question was posted with the help of @Eli.

Answer 1

As you have calculated the torque on the bar is

$$\tau= (P+q)A$$

and a net force

$$F=P-Q$$

This will cause the bar to turn with an angular acceleration,

$$\alpha=\frac{\tau}{I}$$

and also accelerate with,

$$a=\frac{P-Q}{m}$$

Any substitute pair of forces acting within the length of the bar can be scaled by the factor of $A/D$ to impart the same torque. But the new net force will not be the same.

$P_N-Q_N\neq P-Q$.

• A= half-length of bar
• m= mass
• a= linear acceleration
• D = distance of new pair of force Pn, Qn, from the center of the bar
• $\alpha$= angular acceleration
• I= bar's moment of inertia
• $\tau$= torque

So depending on what you demand the answer varies, if you require just the same torque, yes. If you require the same torque and linear acceleration no!