Method to Solve the Problem
This problem requires only 2 things:
- one must remember that a force applied to a rigid body may be considered to act on the body anywhere along its line of action, and
- the application of the parallelogram of forces (PoF) method.
To solve the problem, simply find the resultant force of two of the forces by applying the parallelogram of forces method. Proceed by finding the point of intersection of this resultant force's line of action and the line of action of one of the remaining original forces. Again apply the parallelogram of forces method. You will now have the resultant force from 3 of the original forces as well as its location. Proceed in this manner until you are left with a single resultant force and no original forces.
Solving the Problem
Let's go ahead and perform this analysis on the problem given.
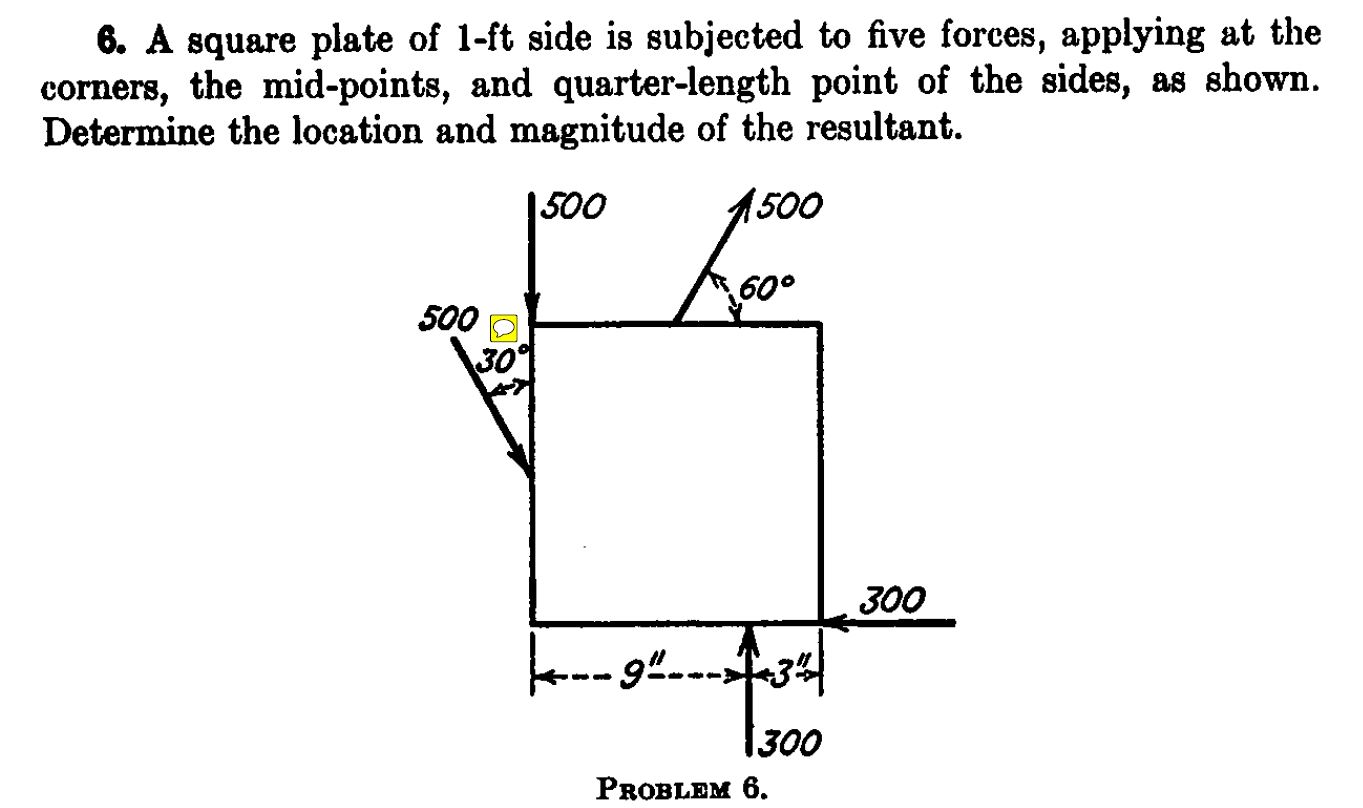
Start by considering the 500 unit force applied to the upper left corner of the plate and the 500 unit force acting at the midpoint of the left edge of the plate. By inspection we see that the intersection point of their lines of action is at the midpoint of the left edge of the plate. Using the Law of Cosines (LoC) we can find the length (and angle) of the resultant of these two forces,
$$
c^2 = a^2 + b^2 -2ab \cdot cos (\gamma)
$$
$$
c^2 = 500^2 + 500^2 - 2 \cdot 500\cdot500\cdot cos(150^\circ)
$$
$$
c = 966
$$
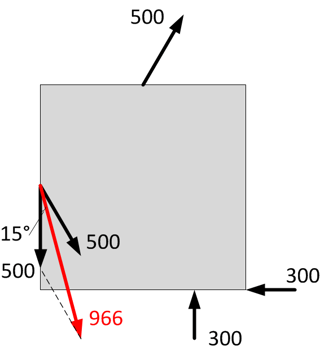
We proceed in the same manner. I did this manually (mathematically via LoC) but I'm too lazy to type it all out. I did, however, check my work by doing it graphically in AutoCAD. Here are some pictures of each step.
Find intersection of lines of action for resultant and top 500 unit load:
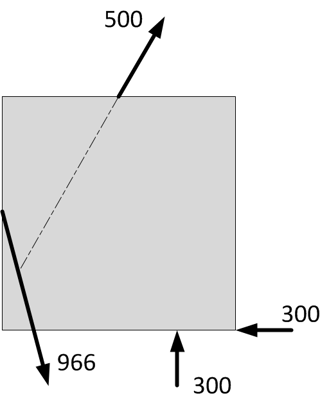
Apply PoF method:
Find intersection of lines of action for resultant and bottom 300 unit load:
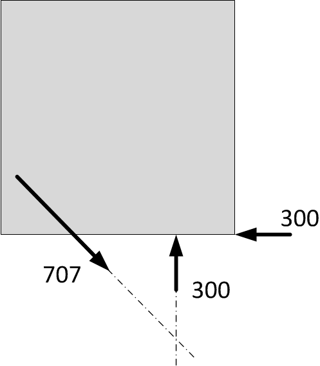
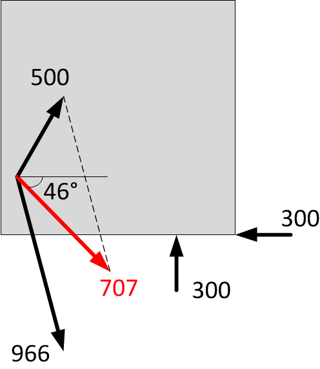
Apply PoF method:
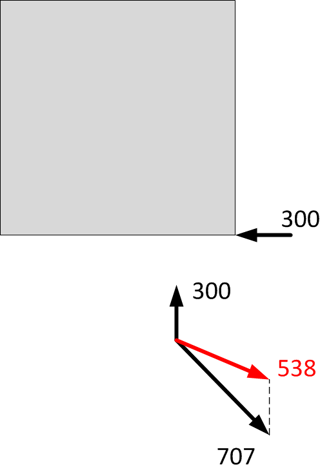
Find intersection of lines of action for resultant and right 300 unit load:

Apply PoF method:
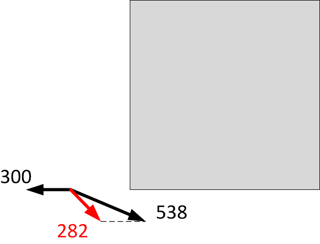
Final Result:
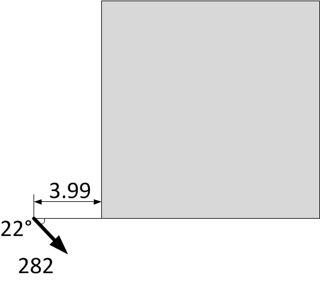
So there you are, how to find the magnitude, location, and angle of the resultant resulting from multiple force vectors applied to a rigid body.
Graphic Statics
The above are a few of the basic principles of a pretty cool but obscure method of static analysis called Graphic Statics.
If you'd like to know more, you should check out the book Shaping Structures | Statics, by Zalewski & Allen. As, alpehzero alluded to, graphic statics used to be an extraordinarily handy tool to solve otherwise tricky analysis problems prior to the advent and subsequent proliferation of computers. Note that research into graphic statics methods, particularly with respect to optimizing truss deisgn, is still being done today