Solving truss using method of joints

Question

So, this is the truss I have to solve, more precisely I have to find forces in all of the members of the truss. That is why I used method of joints. I started from the joint A and realised without calculating that members attached to it (Fab and Fal) are the zero force members.

PICTURE ON THE LEFT: After that I continued the process with joint B and calculated forces in members Fbl and Fbc. If I have made a mistake here, I will appreciate you also point that out.

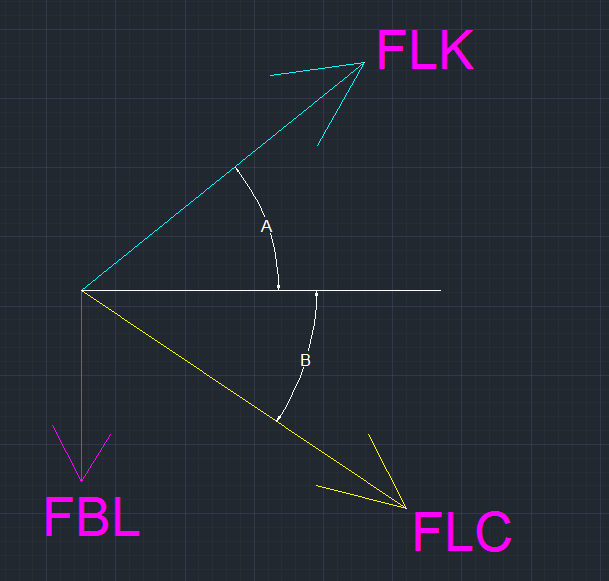
PICTURE ON THE RIGHT: The only joint with 2 unknown members that has left after calculating members of joint B is joint L. But here is the problem. Since I can only use 2 equations - sum of the forces in the X and Y direction (in method of joints), I don't know how to find Flk and Flc, since those are not neither vertical nor horizontal forces. I know I should split those into their components (vertical and horizontal), but since they are both unknown members (so are the components), I don't see way to calculate any of them.

Answer 1

I cannot view your image clearly, but your problem is solvable:

Let me try to explain.

Since FBL is known (FBL = 45 tension), the remaining members are FLK and FLC. You need to find the inclination of FLK. Solve for angles A and B

Summing up forces along Y gives equation:

FLK (sin A) - FLC (sin B) - FBL = 0

Summing up forces along X gives equation:

FLK (cos A) + FLC (cos B) = 0

Then solve the two equations using either: Method of elimination, Method of substitution or by calculator. Please refer to your algebra notes for this :)

Let me give you the first step of Method of Substitution:

FLK = - FLC (cos B) / (cos A)

substitute in the first equation to solve FLC.

Hope this helps.