0
$\begingroup$

I am working on analyzing a few problems, but have not really come across this type of stress concentration problem. Does anyone know where I can study the analysis of this situation?

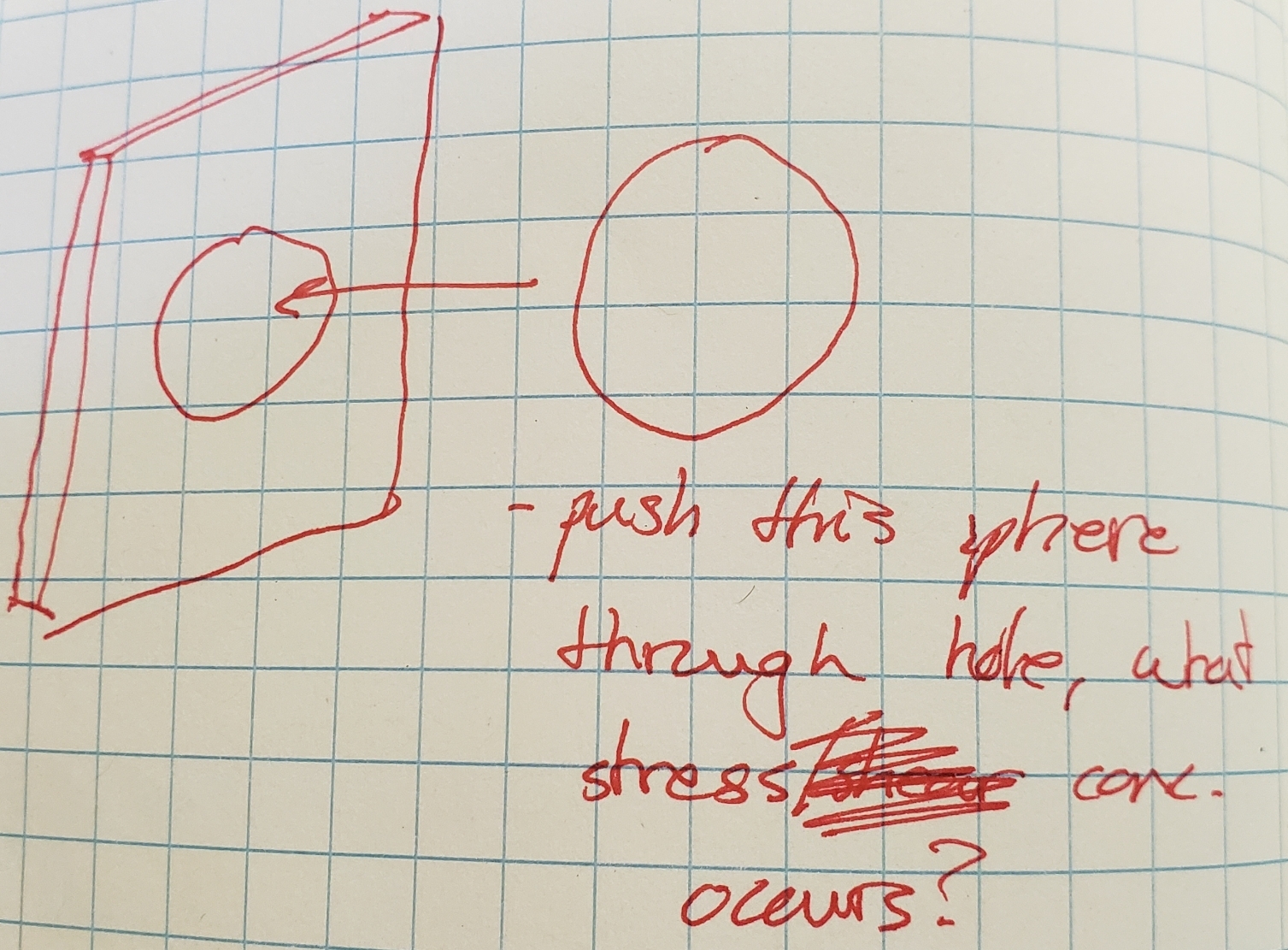
Pressing ball through hole with smaller diameter with some force F

Pressing ball through hole with smaller diameter with some force F

Another problem involves modelling a structure like so, and attempting to find the shear experienced at the connection E:

Bodies 1, 2 and 3 connected (maybe bolts) at lines B and E, if force F_ab acts on body 3, what shear force experienced at connection E? Is it simply summation of forces? Would the geometry changes not matter?

Bodies 1, 2 and 3 connected (maybe bolts) at lines B and E

Any guidance would be much appreciated!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

assume in the first part of your question we are measuring the stresses at the moment the ball is about to pass through the hole. Say R1 is the radius of the hole at rest and R2 is when it is expanded to allow for the passage, and we assume no warping of the surface.

$V = F/2\pi R_2$

As for he second part the shear stress equation for composite beams is: $$ \tau = VQ/It$$ V is the shear from the shear diagram, Q is the first moment of area of the part of the beam above or below the centroid.

All you need is the dimensions and if the platform is made of a different material its E and G, shear modulus of the platform. You need to multiply the platform section area by $E_{platform}/E_{base\ material}$ to calculate the centroid.

Edit

For the first part you need some basic data like the dimension of the hole and radios of the ball, to verify if the stresses are plastic or elastic. You can model the plate around the hole as many say 60 radial cantilever beams connected to each other by concentric rings.

if the plate is thin enough it will allow bending around the edge of the hole or it will make the whole expand while creating a depressing. Another case that can be modeled in FEM is the bell itself will shrink into a football shape to pass through.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks, For the first part, I guess I am more interested in the stress concentration experienced prior to the radius reaching R_2 (I'd like to know what forces the hole can handle before failure for a given material). For the second part, I was under the impression that the equation given is for transverse shear? (i.e. the shear at line x of i.gyazo.com/0fa02ad3765777aadb6a09461294c795.png) But in my case, I guess it would be a flipped version of that with the force acting in the x direction instead, which would then not allow me to find the shear at line E as I intend? $\endgroup$
    – dan
    Commented Dec 3, 2018 at 9:39
  • $\begingroup$ sorry, after reviewing shear force a bit more I see how the equation will work in the current configuration; so I believe my approach will now be to move section by section A -> B, B -> C, calculating the shear stress for each of the different cross sectional areas. $\endgroup$
    – dan
    Commented Dec 3, 2018 at 9:58
  • $\begingroup$ response to edit: would the contact reaction force around the hole then be a force per unit circumference? and this is the force that I would use on the cantilever beam? (and I guess that during the deformation, R would change from R1 to R2, maybe I use just R1 for a conservative approach) $\endgroup$
    – dan
    Commented Dec 4, 2018 at 15:05
  • $\begingroup$ @dan For the first question, there is no stress concentration but flexural stresses depending on the support condition of the edges. For the second case, again, how the built-up section is supported. Is it part of a beam or a cantilever? Are you looking for the shear force on the connection or shear flow? You need to clarify your question to get the correct answers. $\endgroup$
    – r13
    Commented Apr 21, 2021 at 22:48

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.