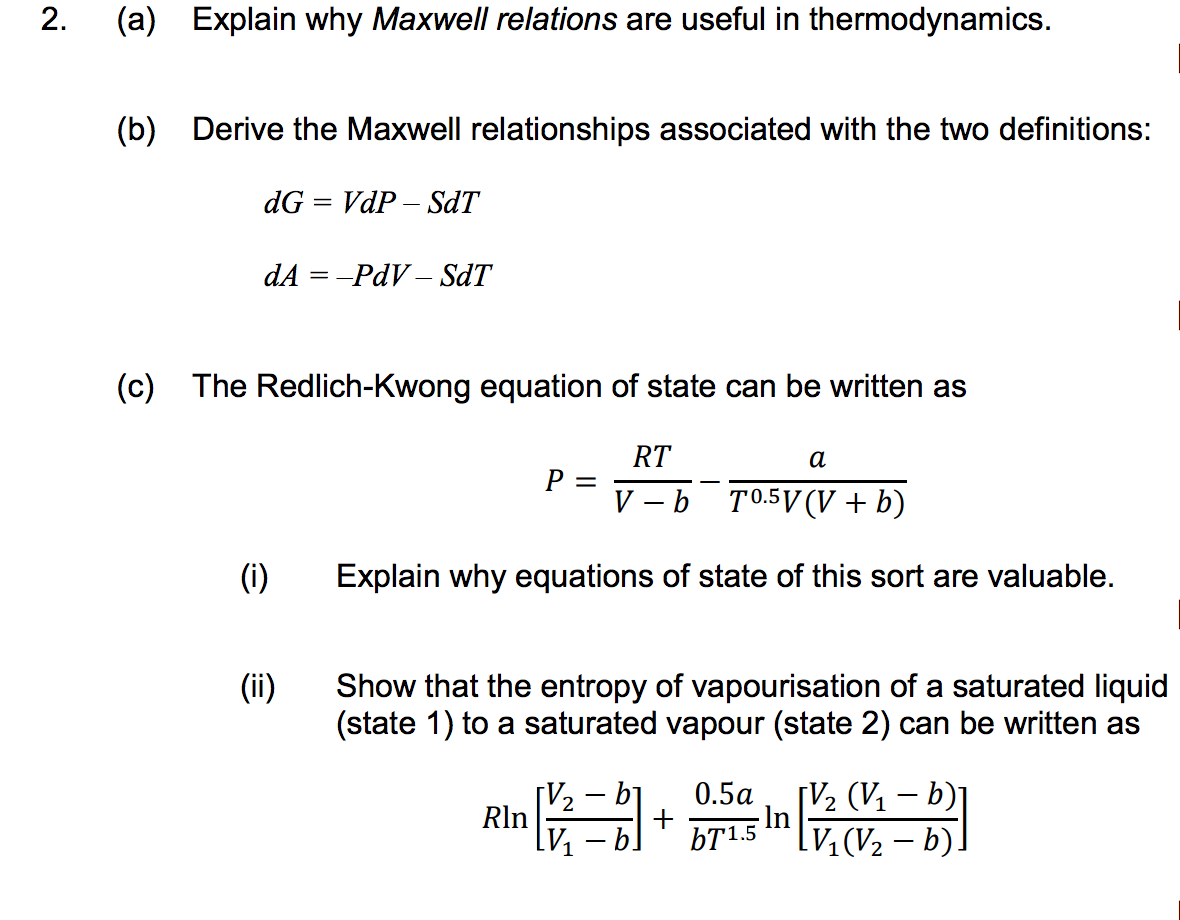
The part I'm stuck on is the last part. Basically, the question is to obtain the following equation for the entropy of vaporisation using the Redlich-Kwong equation: $$ \Delta S = R\Bigg[ \ln \frac{V_2 -b}{V_1 - b} \Bigg] + \frac{0.5a}{bT^{1.5}}\ln \Bigg[ \frac{V_2(V_1-b)}{V_1(V_2-b)} \Bigg] $$
Solution Attempt:
I think I should start by using the known fact that at equilibrium, the free energy of both phases must be the same. Using Gibbs free energy: $$ dG = -SdT + VdP \implies -S_1dT + V_1dP = -S_2dT + V_2dP $$ Therefore, I can write an equation for change in entropy due to vaporisation: $$ S_1 - S_2 = (V_1 - V_2)\frac{dP}{dT} $$ However when I simply differentiate the given EoS and multiply by $V_1 - V_2$ I don't get the same result. Clearly, in the answer they have integrated wrt v at some point but I just don't get why or how. Any help will be appreciated, thank you.