1
$\begingroup$

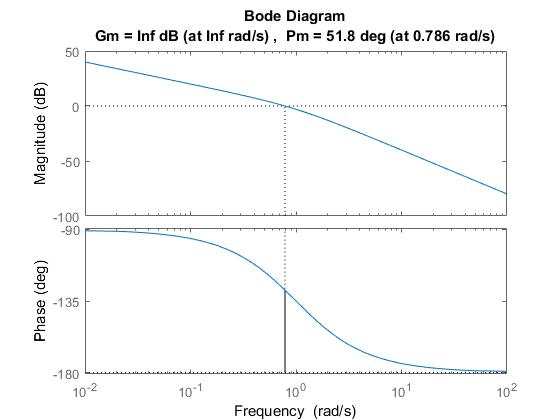
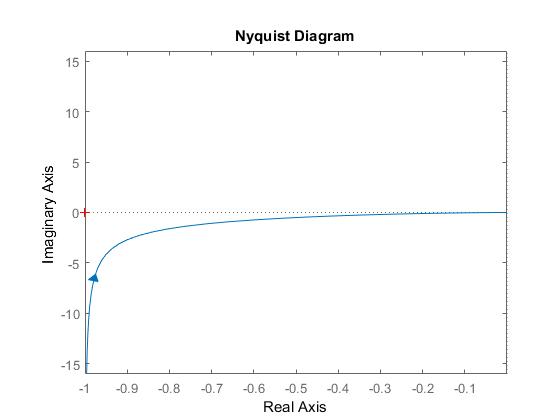
Consider $$\hat{G}(s) = \frac{1}{s^2+s}$$ than the Nyquist plot is nyquist plot

and the Bode plot is Bode Plot

In both plots it seems that the closed-loop system is stable even when the eigenvalues are {0, -1}. For a higher order closed-loop system one can see that a system like

$$\hat{G}(s) = \frac{1}{s^3+s^2+s}$$

is at its stability limit since ne Nquist plots hits the real axis at -1 and in the Bode plot there is no phase reserve when magnitude hits 0. Why dose the Nyquist and the Bode plot fail for a closed-loop system of 2nd order?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

I think you are mixing up the closed- and open-loop systems.

The closed-loop system is $$\frac{\frac{1}{s^2+s}}{1+\frac{1}{s^2+s}}=\frac{1}{s^2+s+1}$$

This has poles at $-0.5\pm 0.866025 i$ which is stable.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Am i only allowed to view the plots for closed-loops? $\endgroup$
    – solid
    Commented Nov 29, 2016 at 18:15
  • 1
    $\begingroup$ The Nyquist stability criterion and things like gain and phase margin that are seen on the Bode plot are for the closed-loop system. The utility of these plots is that you can get insights into the closed-loop behavior using the open-loop transfer function. $\endgroup$
    – Suba Thomas
    Commented Nov 29, 2016 at 18:17
  • $\begingroup$ I don't get your point, so let the open-loop system be $\frac{1}{s^2+s-1}$ so the closed on is $\frac{1}{s^2+s}$ now the eigenvalues of the closed one are still {0, -1} but my bode plot still shows a phase reserve of 51.8 deg when the magnitude hits 0 $\endgroup$
    – solid
    Commented Nov 29, 2016 at 18:58
  • $\begingroup$ For $\frac{1}{s^2+s-1}$, when the magnitude is 0 db, the phase is -180 degrees. The phase margin is 0. $\endgroup$
    – Suba Thomas
    Commented Nov 29, 2016 at 19:29
  • $\begingroup$ but not in the matlab plot above $\endgroup$
    – solid
    Commented Nov 29, 2016 at 19:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.