Steady state error of negative feedback system - zeroes of feedback function

Question

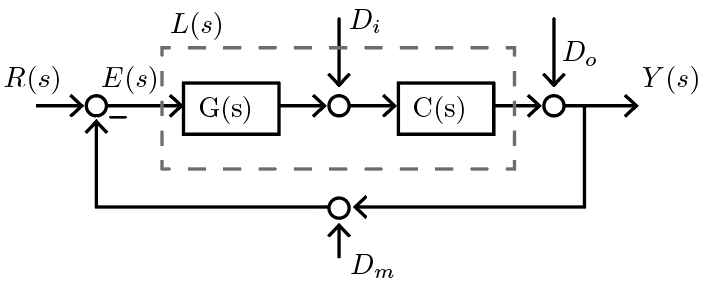
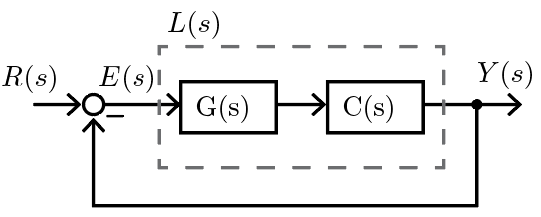
I am currently studying control systems from the KUO - GOLNARAGHI book. Regarding the steady state error, the book makes the following distinction:

1. Feedback function H(s) has no zero at s = 0
2. Feedback function has zero of order N at s = 0. In this case, the closed loop transfer function M(s) has extra poles at s = 0.

Based on that, we get two different methods of calculating the error. My questions are:

a. What is the significance of whether H(s) has zeroes at s = 0 and

b. Why does this affect the poles of M

Answer 1

despite that you are not providing any reference of the transfer function, I would try to give you some remarks. Lets assume you are looking a system of this kind:

In order to not make it too complicated lets neglect the disturbances to have a simplified model, where the open loop is described as $L(s)=G(s)C(s)$.

1. The steady state error ($e_\infty$ )is calculated using $e_\infty=\lim _{s\rightarrow0}s\,E(s)$, where $E(s)=R(s)-Y(s). $From the block diagram $Y(s)=\frac{R(s)L(s)}{1+L(s)}$. Replacing this in the limit, we obtain $$e_{\infty}=\lim _{s\rightarrow0}s\,E(s)\\ =\lim_{s\rightarrow0}s\,(1-\frac{L(s)}{1+L(s)})R(s)\\ =\lim_{s\rightarrow0}s\,\frac{1}{1+L(s)}R(s)$$

2. Zero and Pole definition: A zero is a value that causes the numerator to be zero in the a transfer function, meanwhile, a value that causes the denominator to be zero is a pole.

3. if the transfer function do not have zeroes then it could be represented as $L(s)= K\,\frac{1}{N(s)}$, where $K$ is a gain and $N(s)$ is the denominator. Now all you have to do is replace this in the steady state error $e_\infty$ and analyze

4. Conclusion regarding your first question: Of course the limit analyzed in point 1 can give back an unstable value for some circumstances (i.e. $\infty$), thats why is important to recognize the zeroes on the transfer function. Having more zeroes at s=0 will affect the dynamics and the stability of the system.

5. Conclusion regarding your second question: if your system has a pole in the zero value and also a zero in the numerator, this will be reduced in $e_\infty$