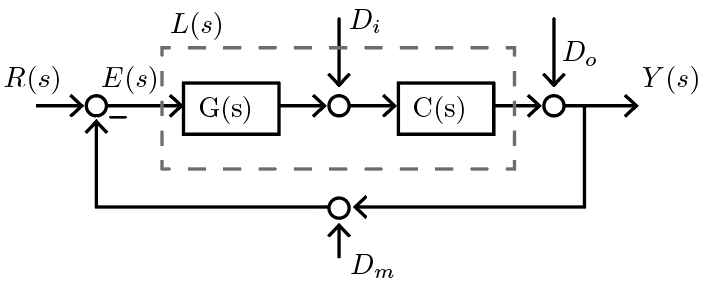
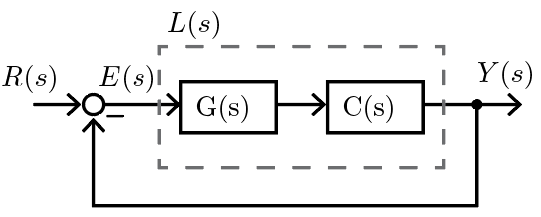
despite that you are not providing any reference of the transfer function, I would try to give you some remarks. Lets assume you are looking a system of this kind:
In order to not make it too complicated lets neglect the disturbances to have a simplified model, where the open loop is described as $L(s)=G(s)C(s)$.
The steady state error ($e_\infty$ )is calculated using $e_\infty=\lim _{s\rightarrow0}s\,E(s)$, where $E(s)=R(s)-Y(s). $From the block diagram $Y(s)=\frac{R(s)L(s)}{1+L(s)}$. Replacing this in the limit, we obtain $$e_{\infty}=\lim _{s\rightarrow0}s\,E(s)\\ =\lim_{s\rightarrow0}s\,(1-\frac{L(s)}{1+L(s)})\\ =\lim_{s\rightarrow0}s\,\frac{1}{1+L(s)}$$$$e_{\infty}=\lim _{s\rightarrow0}s\,E(s)\\ =\lim_{s\rightarrow0}s\,(1-\frac{L(s)}{1+L(s)})R(s)\\ =\lim_{s\rightarrow0}s\,\frac{1}{1+L(s)}R(s)$$
Zero and Pole definition: A zero is a value that causes the numerator to be zero in the a transfer function, meanwhile, a value that causes the denominator to be zero is a pole.
if the transfer function do not have zeroes then it could be represented as $L(s)= K\,\frac{1}{N(s)}$, where $K$ is a gain and $N(s)$ is the denominator. Now all you have to do is replace this in the steady state error $e_\infty$ and analyze
Conclusion regarding your first question: Of course the limit analyzed in point 1 can give back an unstable value for some circumstances (i.e. $\infty$), thats why is important to recognize the zeroes on the transfer function. Having more zeroes at s=0 will affect the dynamics and the stability of the system.
Conclusion regarding your second question: if your system has a pole in the zero value and also a zero in the numerator, this will be reduced in $e_\infty$