Steady-state tracking error has to equal zero, what values do I need?

Question

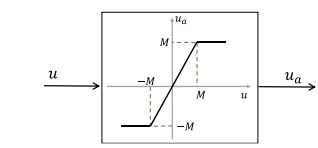
A block is used to model instruments with limited capabilities to provide inputs to the plant. A seen below:

The output of the block 𝑢𝑎 is related to the input 𝑢 with the following input-output relationship:

$$u_a = \begin{cases} -M & u < -M \\ u & u \in [-M, M] \\ M & u > M \\ \end{cases}$$

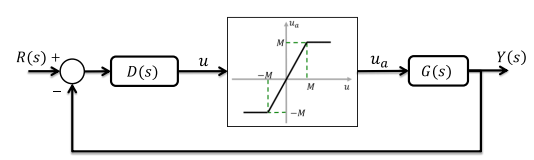
The control system can be seen below:

Given that $D(s)=15\dfrac{s+0.1}{s}$ and the transfer function of the plant is $G(s)=2(s+0.8)/(s+4)(s+36)$

How can I find the smallest value of 𝑀 to guarantee that the steady-state tracking error is zero when the reference input is a step function with amplitude 8 ?

I have calculated the transfer loop equation $L(s)=\dfrac{30(s+0.1)(s+0.8)}{(s+4)(s+36)}$ but I am completely stuck.

Answer 1

short answer, any non-zero saturation limited suffices. This is due to the integral action in the controller.

Steady-state error can be evaluated by computing the closed-loop transfer function and taking the limit of $s\rightarrow0$. Lets do that by removing the saturation:

$$T(s) = \frac{L(s)}{1+L(s)}$$ $$T(s) = \frac{30(s+0.1)(s+0.8)}{s(s+4)(s+6)+30(s+0.1)(s+0.8)}$$ $$\lim_{s\rightarrow0}\frac{30(s+0.1)(s+0.8)}{s(s+4)(s+6)+30(s+0.1)(s+0.8)} = 1$$

Recall that $T(s) = \frac{Y(s)}{R(s)}$, which means that if $T(0) = 1$, the DC (steady state) gain of the closed-loop transfer function is 1. This indicates it can track any constant reference perfectly. However, it should be noted that this is only in theory, and in practice this is not true. You are limited to the resolution of your sensors and you might not get enough power from the saturated input to overcome the static friction.