How does the stiffness of semicircular beams connected in series increases?

Question

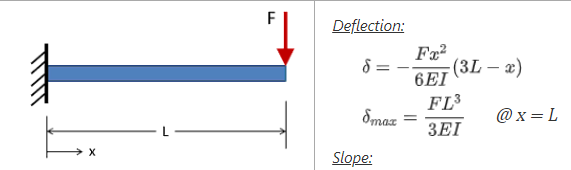
I am working to reduce the deflection in a cantilever/ dive board at work.$300 mm(L) \times 30mm(B) \times 2 mm(H)$, i.e. it's a rectangular cross section currently.

I can't change the material, or length.

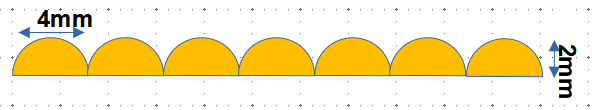
I started playing with cross sections to reduce the deflection. So I stacked 7 semi circular beams of 4mm Dia. next to each other(thus not changing the height from rectangular beam) assuming it would increase my "I" by 7 X I of single semi circular beam, thus making it stiffer than the current beam but IT DID NOT. Any idea why?

Calculations:

• I(Rectangular cross section): $bh^3/12= 20 mm^4$

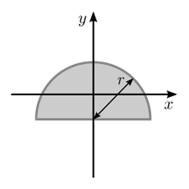
• I (single Semicircular Beam of Dia 4)= $\pi \cdot r^4/8= 6.28 mm^4$

Again, I assumed stacking up next to each other would make the total area moment of inertia(I) = $7 \cdot 6.28 = 43.96 mm^4.$

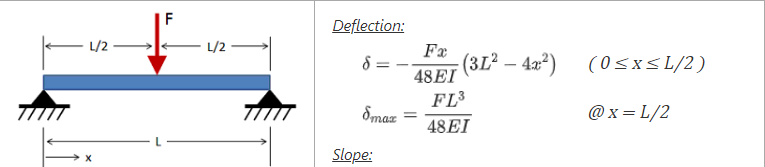
And Using Deflection = $FL^3/48EI$, I thought it would decrease my deflection by more than half.IT DIDN'T WORK. FEA results showed increase in deflection.

Any idea why? Sorry if it's a dumb question

Answer 1

If I understood correctly from your post you started with the following cross-section (Lets say A)

and you replaced it with the following (or the inverted) (let's call it B).

There is one reason why you are getting larger deflections,

the total second moment of area is smaller.

Crosssection A

The total second moment of area for Cross-section A along axis X is:

$$I_{xx,A} = \frac{bh^3}{12} = 20[mm^4]$$

Crosssection B

For cross-section B, the actual moment of area is -indeed- 7 times the moment of area for the semi-circle (SC). $$I_{xx,B} =7 \cdot I_{xx, SC}$$

However, the semi-circle $I_{xx, SC}$, is given by (see wikipedia) : $$ I_{xx, SC}= 0.1098r^4$$

Therefore the total $I_{xx,B}=12.3 mm^4$.

---

Comparison

According to the above you should expect approximately 60% more deflection from case B, because:

• $I_{xx,A}=20 mm^4$
• $I_{xx,B}=12.3 mm^4$

However that was to be expected because, if you put the two cross-section together you'd see that cross-section A encapsulates B.

-

Answer 2

You made two mistakes in your hand calculation:

1) Wrong equation was used to calculate the moment of inertia - you used the equation for $I_y$ instead of $I_x$, thus resulting in the hand calculation being larger than the calculation done by the FEM program ($I_y$ > $I_x$).

$I_x = \frac{(9\pi^2 -64)r^4}{72\pi} \approx 0.1098r^4$

$I_y = \frac{\pi r^4}{8} = 0.3927r^4$

2) Wrong equation was used for the deflection calculation.

The equation you have used corresponds to a simply supported beam with a concentrated load in the mid-span.

Thus, the FEM reported a much larger deflection than you had anticipated.

Please keep in mind, the correct/successful design of a diving board is quite involved, which shouldn't be contemplated by an amateur without being mentored, or supervised by a knowledgeable person.