Reaction force on a beam

Question

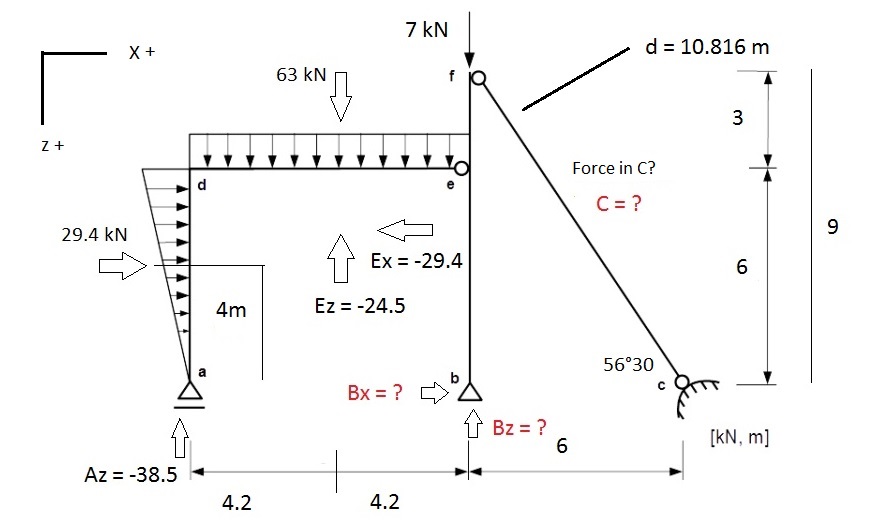
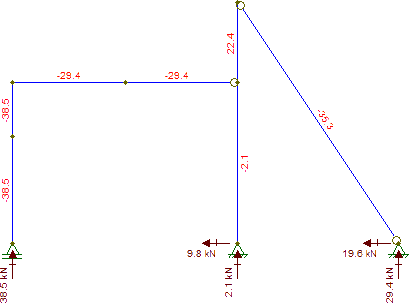
I have the following problem most of which I have calculated but have a difficulty with the beam under angle. The angle is 56,30 degress. I just cannot figure out how the forces there are acting - the equations I am making have three unknown variables in two equations, which cannot be calculated, so apparently there are forces I "cannot see" there as well and the equations are wrong.

The disributed loads have been replaced with concentrated loads: the point where they act is indicated.

Thank you very much for advice.

Answer 1

It is worth noting that this can be treated as two separate structures:

I assume that's how you solved the reactions of support A. Given that, you can ignore the entire left-hand structure and act like you only have the right-hand side.

For this, let's use the standard equations:

$$\begin{align} \sum F_x &= B_x + C_x + 29.4 = 0 \\ \sum F_z &= B_z + C_z - 24.5 - 7 = 0 \\ \sum M_B &= -29.4 \cdot 6 + 6C_z = 0 \\ \therefore C_z &= 29.4\text{ kN} \\ \therefore B_z &= 24.5 + 7 - C_z = 2.1\text{ kN} \end{align}$$

It might seem like you're now stuck without solving for $F_x$, but you can notice that the diagonal member is a truss, with only axial loads. That means that the vertical and horizontal forces applied to it must be proportional to its tangent. Therefore we have:

$$\begin{align} C_x &= -C_z \cdot \dfrac{6}{9} = -19.6\text{ kN} \\ \therefore B_x &= -29.4 - C_x = -9.8\text{ kN} \end{align}$$

To check our work: