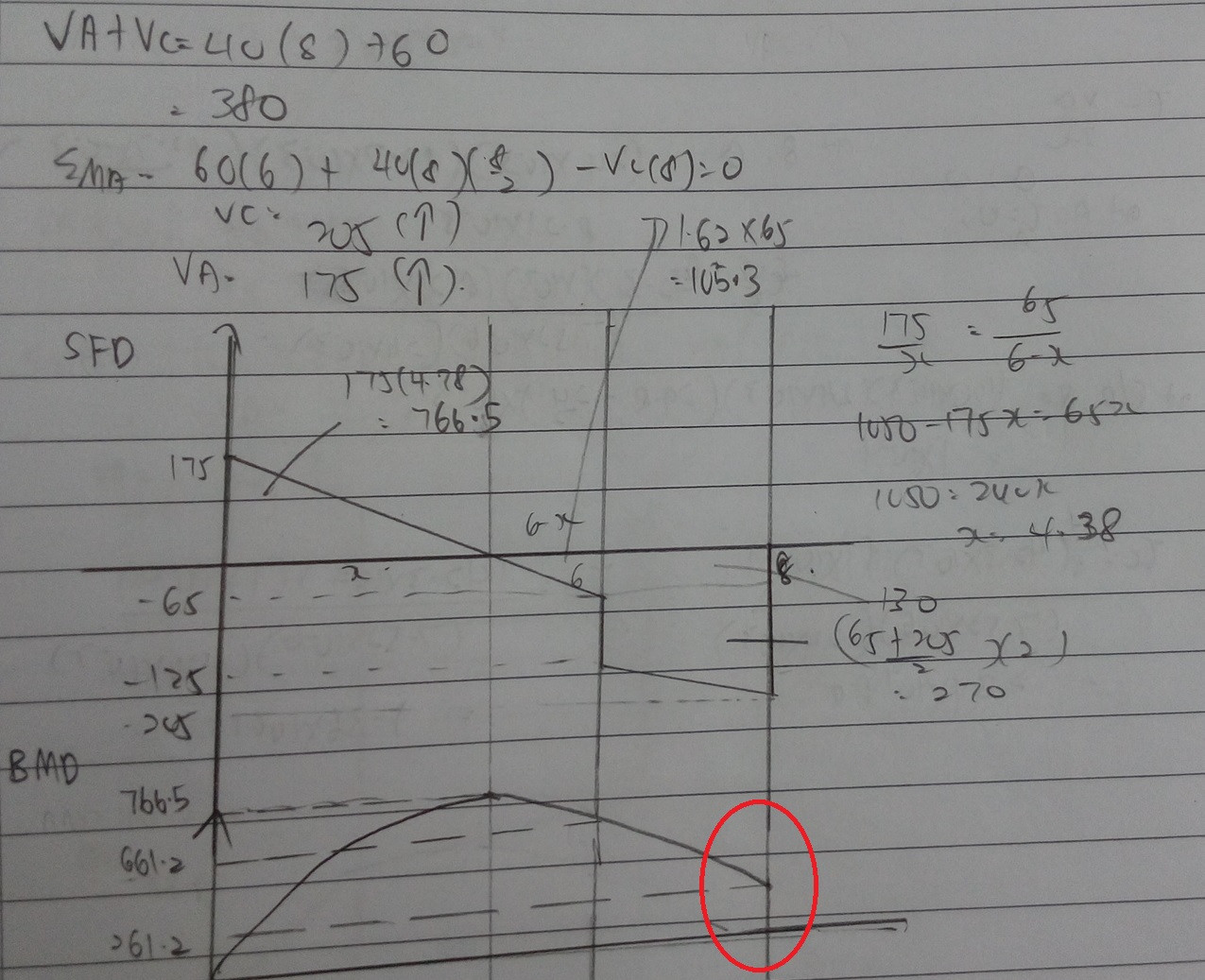
Your shear diagram is correct.
Bending moment is equal to the integral of shear. Therefore, the point of maximum bending moment is the point of zero shear. I can see you calculated that point as 4.38, which is correct (in fact, it's 4.375, but rounding is fine). That is found by
$$6\cdot\dfrac{175}{175+65} = 4.375\text{ m}$$
Now you know the point of maximum bending moment. You chose to calculate the value by integrating the shear diagram. That is equivalent to calculating the total area of the shear diagram up to that point, which is equal to
$$175\cdot\dfrac{4.375}{2} = 382.8\text{ kNm}$$
Your mistake was that you calculated the area of the rectangle $(175\cdot4.375$) instead of the triangle that describes the shear diagram.
Adopting the correct bending moment value, you can then correct the rest of it as well:
$$\begin{align}
M_B &= 382.8 - 65\cdot\dfrac{6-4.375}{2} = 330.0\text{ kNm} \\
M_C &= 330.0 - \dfrac{125+205}{2}\cdot2 = 0\text{ kNm}
\end{align}$$
And now, to check our work:
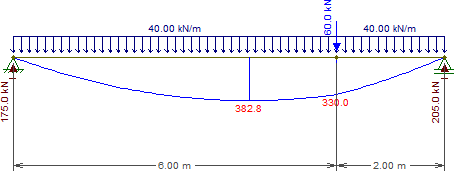
Diagram obtained with Ftool, a free 2D frame analysis program.