0
$\begingroup$

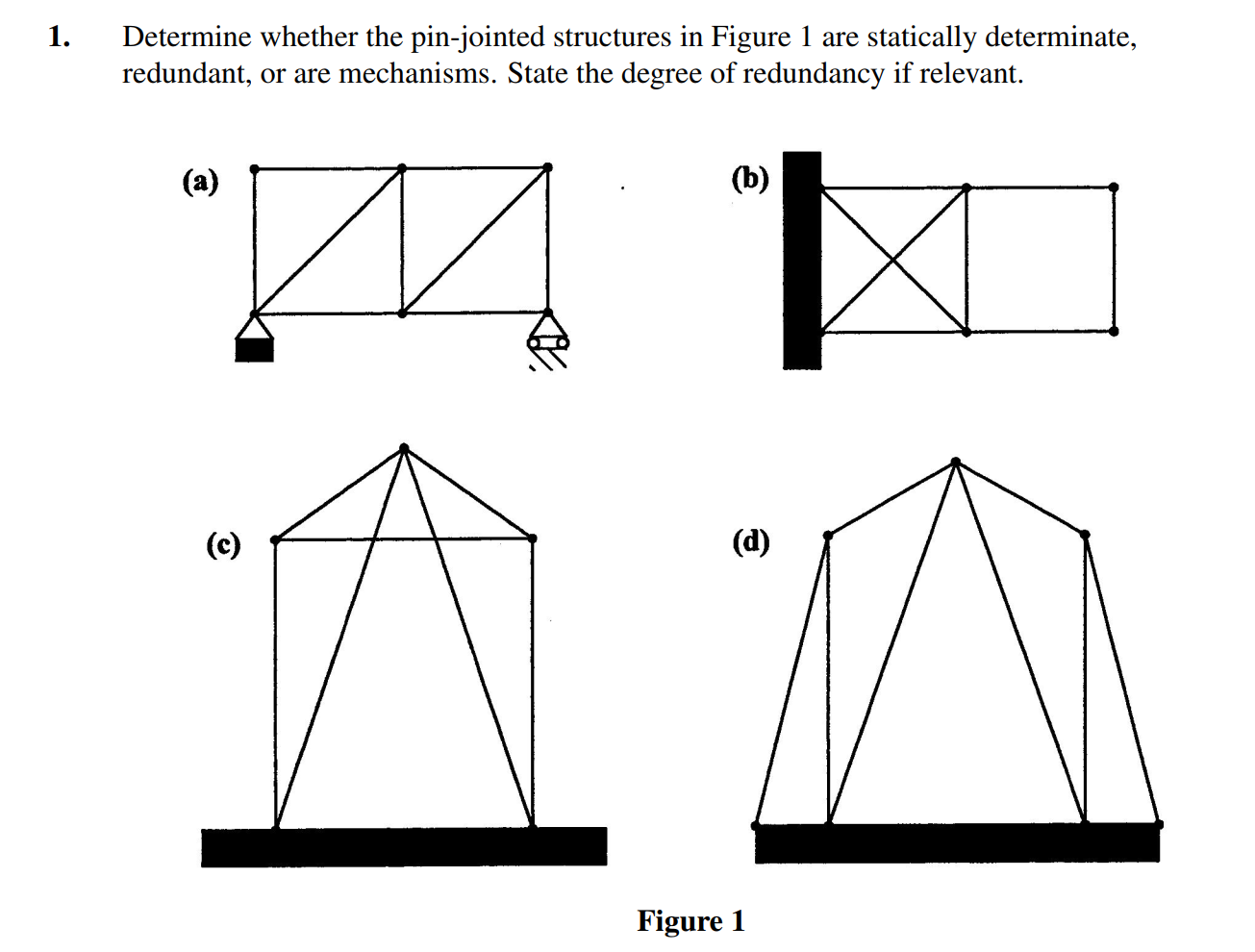
enter image description here

I was doing exam question and am confused as to how to determine if the structure is a mechanism or not.

Say that j = number of joints, r = total number of reactions, b = number of bars.

For both (a) and (b) I get 2j-r = b which is a necessary condition for static determinacy.

For (c) I get 2j-r = 6, b = 7 => 2j-r < b which I guess is a condition for static indeterminacy. But this structure by inspection looks unstable.

Same for (c), where I get 2j-r = 6, b = 8 => 2j-r < b which means it is supposed to be statically indeterminate structure but looks very unstable.

What I have been taught is that we have to try to "press" it, i.e. imagine exerting an external force at a certain joint and see if the structure collapses or not. But I get confused when I actually get to do it. Could you please help with this.

Also from the matrix method of solving the linear system I thought 2j-r < b is a necessary and sufficient condition for static indeterminacy.

Say that the linear system for the structure is expressed as E*t + f = 0, where E is the coefficient matrix, t is the tension vector and f is the external force vector.

The size of the matrix E will be 2j-r rows and b columns so if 2j-r < b then there are more variables than equations so I thought it should be a necessary and sufficient condition.

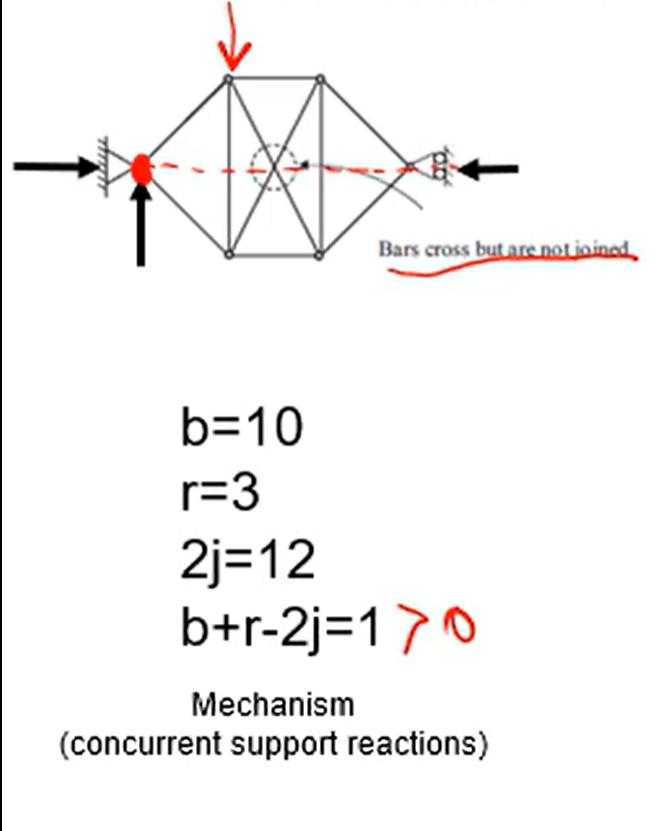
But my lecturer says that the below example shows the case in which 2j-r < b and the structure is still a mechanism, so I was wondering if for this one as well it's only a necessary condition, and if so why that is.

enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ The formula you use only works in certain cases, B is a mechanism since it has a solid structure coupled with a fourbar. C is also a special case.the bottom triangle us stable. The upper triangle one can rotate about its pivot with the first. The fourbar left over can move but not in the direction the triangle can... Not a good idea for a real structure though. $\endgroup$
    – joojaa
    Commented Jun 13, 2022 at 20:21
  • 1
    $\begingroup$ To add further you need to remove overdetermined subsystems in calculation or they will mask moving parts. $\endgroup$
    – joojaa
    Commented Jun 13, 2022 at 20:29

1 Answer 1

Reset to default
0
$\begingroup$

The answer for b) should have a redundancy of second degree - j = 6, r = 6, b = 8, i = (b+r) - 2j = (8+6)-2*6 = 2.

For c), redundancy of third degree - j = 5, r = 6, b = 7, i = (7+6) - 2*5 = 3.

For the truss in the last graph, the instability is caused by the roller, for which the translation in the y-direction is unrestrained, resulting in global instability.

Improve this answer
$\endgroup$
2
  • $\begingroup$ it depends on how you interpret the joint at the solid black line. Since this is a truss and not a frame, I took it as a moment connection is not possible at the support. Therefore for b) r = 4 for 2 pinned connections. 2*6-4= 8 = b= 8 which would be statically determinant. Though it is admittedly unstable at the right end were the members form a box. $\endgroup$
    – Forward Ed
    Commented Jun 13, 2022 at 19:12
  • $\begingroup$ Obviously, you are correct, as the OP does not accept my answer. $\endgroup$
    – r13
    Commented Jun 13, 2022 at 19:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.