Can anyone please explain this question to me? I am okay with answering a gear assembly with 2 gears at a time. But the third one here is confusing me.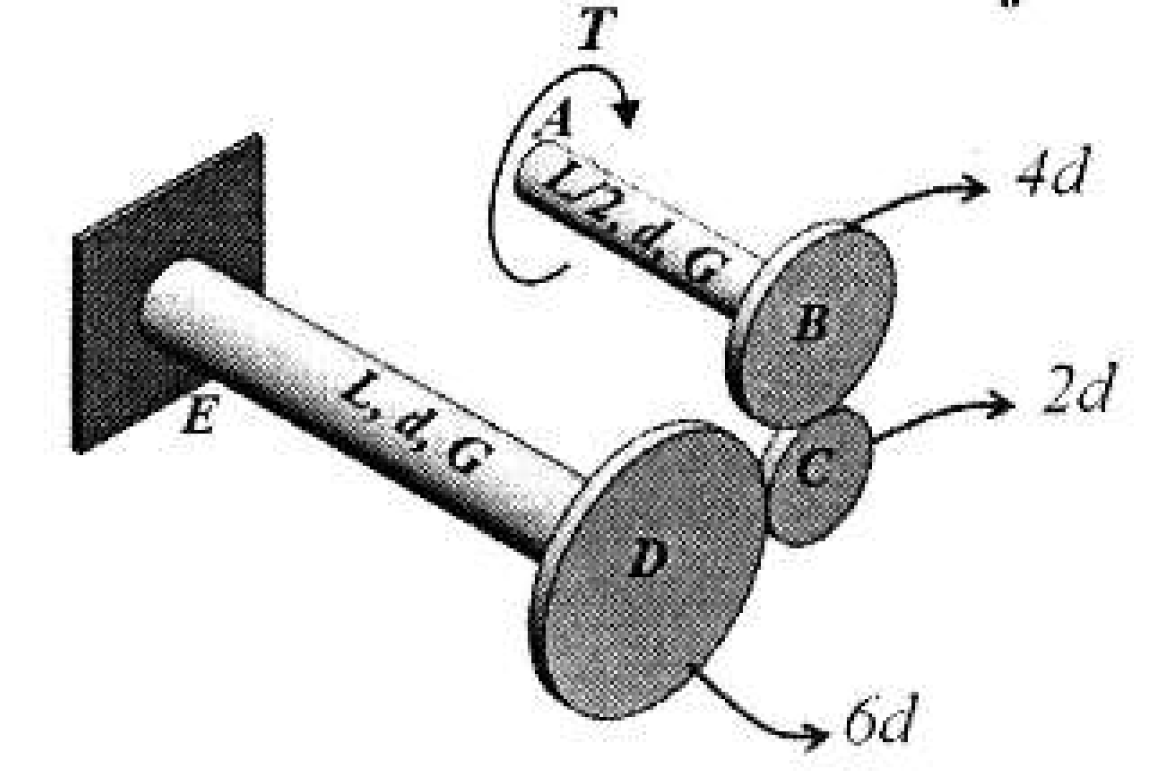
-
1$\begingroup$ gear C (the "idler") has no effect on the output except to reverse it's direction. $\endgroup$– Jonathan R SwiftCommented Mar 4, 2021 at 7:54
-
$\begingroup$ @JonathanRSwift Thanks. $\endgroup$– Ali KhaliliCommented Mar 7, 2021 at 8:36
1 Answer
There a few steps in this problem.
- The first one is to figure out what is the torque that each shaft is subjected.
- The second one is to determine the twisting angle.
In this you are making the assumption that the disks do not deform.
So the regarding the torque (if you know the basics about gear assemblies this is obvious):
- the short rod (L/2) is subjected to T torque
- the long rod (L) is subjected to $\frac{3}{2} T$ torque
The magnitude of the twist is given by:
$$\Delta \theta = \frac{M_{t,i} L_{i}}{G_i J_i}$$
where $J_i =\frac{\pi d^4}{32}$
therefore the twist is :
- for the short $$\Delta\theta_s = \frac{T L }{2 \;G \;J}$$
- for the long $$\Delta \theta_L = \frac{3 T L }{2\; G \;J}$$
However because of the gear ratio from long to short being 3/2, twisting one unit on the long (DE) will result in 3/2 unit rotation on the short.
Therefore the final twisting of point A is:
$$\Delta\theta_A=\Delta\theta_s +\frac{3}{2}\Delta \theta_L $$ $$\Delta\theta_A=\frac{T L }{2 G \;J} +\frac{3}{2}\frac{3 T L }{2 G J} $$ $$\Delta\theta_A=\frac{T L }{G J} \left (\frac{1}{2}+\left(\frac{3}{2}\right)^2\right)$$ $$\Delta\theta_A=\frac{11}{4}\frac{T L }{G J} $$
Finally substituting $J_i =\frac{\pi d^4}{32}$ (if everything went ok)
$$\Delta\theta_A=88\frac{T L }{G \pi d^4} $$
-
$\begingroup$ Thank you for your clarification. $\endgroup$ Commented Mar 4, 2021 at 6:49