Modelling an annular ring

Question

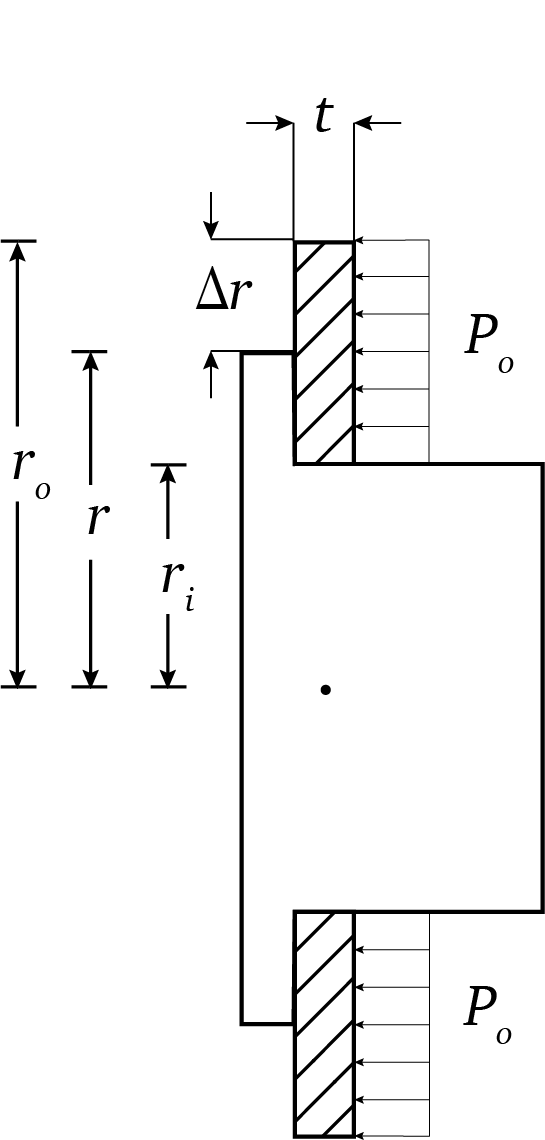
I am trying to model the shear failure of an annular ring with a uniformly distributed pressure, the ring is hashed out. I am not sure how the problem would be modelled as part of the ring is supported and under pressure and the other section is unsupported and under pressure. Any advice would be appreciated.

Answer 1

In this particular scenario, you can have two types of fixtures with two different

a) fixture to the right (See red)

In that case you only need to test for the shear failure of the ring. The shear stress will be:

$$\sigma_s = \frac{F}{A} $$

where:

• $F = \pi (r_o^2 - r^2) P_0$ : the force on the shear surface
• $A = 2\pi \cdot r \cdot t$: the shear surface

$$ \sigma_s = \frac{\pi (r_o^2 - r^2) P_0}{2\pi \cdot r \cdot t} $$

So finally:

$$ \sigma_s = \frac{ (r_o^2 - r^2) }{2 \cdot r \cdot t} P_0$$

And you just need to check whether the operating stress $\sigma_s$ is less than the allowable stress of the ring material.

b) fixture to the right

If

• the thickness of the flange $t_f$ of the "tap" (for lack of a better word), is less that the thickness of the ring (t),
• and the tap is supported at the right hand side (see red line).

you might need to check (under certain conditions) for shear failure at the green regions shown below .

In that case, the shear failure would be

$$\sigma_s = \frac{F_2}{A_2} $$

where:

• $F_2 = \pi (r_o^2 - r_i^2) P_0$ : the force on the shear surface
• $A_2 = 2\pi \cdot r_i \cdot t$: the shear surface

$$ \sigma_s = \frac{\pi (r_o^2 - r_i^2) P_0}{2\pi \cdot r_i \cdot t} $$

However, in this case failure due to bending needs to be investigated as well.

Answer 2

If the ring were free-standing it would support the pressure P by tangential tensile stress. $\sigma_{tensile}=P*r/t*\Delta r$

We need the elastic properties of the outer layer. Just for sake of argument let's say the

$E_{big \ ring}=1.5*E_{small\ ring}$ And say the thickness of the bushing is the same as the interior ring and the middle ring could be assumed rigid.

Now we have shear and moment (similar to cantilever moment and shear) at 2 levels: the transition from free ring to double thickness with the bushing lip and at the lower level transition from double layer to the rigid thick ring.

At the first transition, we have much less stress in the ring so it stretches less and moment and shear stress is created. how much less extension? the new thickness is equal to$ 1.5 t+t=2.5 t$ so the circumferential and radial elongation is 2.5 times less than the top part.

Ther are ways to determine the moment and shear at this intersection. but my guess is it is easier to use a FEM program to handle it.