Calculating Admissible Shear stress with a uniformly distributed load and a point load

Question

I have the following equation for calculating the Admissible shear stress for a uniformly distributed load.

$$\tau_{adm} = \frac{3}{2}\cdot \frac{F\cdot L}{2b\cdot h}$$

where

• $F$ = kN/m
• $L$ = Effective length of beam
• $b$ = width of timber
• $h$ = depth of timber

I am struggling to find out how this formula was derived. And secondly how this formula would need to be amended to include a point load.

Answer 1

Shear stress in beam section coming from lateral force is not uniform across the section. Derivation comes from Zhuravskii. The local lateral force basically increases bending moment when you move a little bit along the beam axis. So you can investigate bending stresses in consecutive sections.

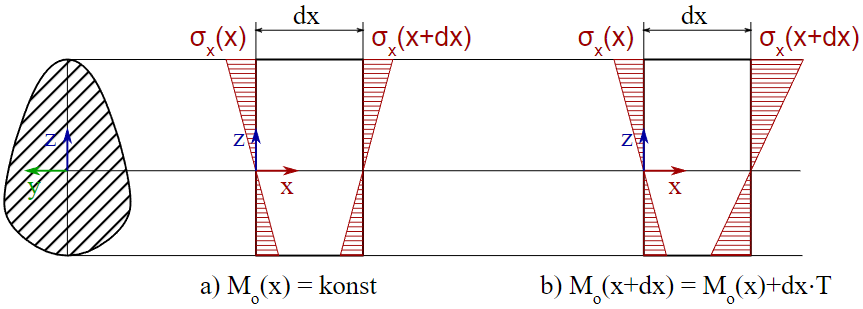
Not the trick is to cut the part between 2 sections horizontally, add the unknown shear stress $\tau$ and calculate it from the equilibrium.

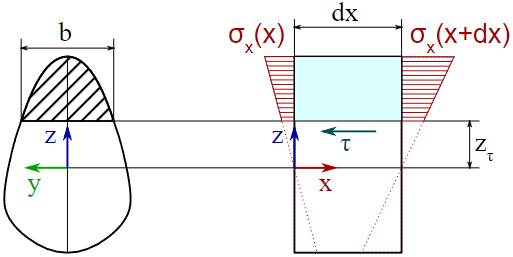
Thea equilibrium would be: $$- \int\limits_{\gamma_\tau} \sigma_x(x, z)dydz + \int\limits_{\gamma_\tau} \sigma_x(x+dx, z)dydz - \tau\cdot b\cdot dx = 0$$

Stresses directly from moments $M_o$ cancel out, and what remains comes just from lateral force $T$:

$$\tau \cdot b\cdot dx = \frac{T\cdot dx}{I_y}\cdot \int\limits_{\gamma_\tau} z dydz$$

This can be simplified to: $$\tau = \frac{T\cdot U_\tau}{b\cdot I_y}$$

where:

• $T$ is the local lateral force
• $U_\tau$ is the first moment of the area above the horizontal section
• $I_y$ is the second moment of area for the whole section
• $b$ is local section width at the investigated coordinate $z$

which is basically the Zhuravskii shear stress formula.

In your case: $$I_y = \frac{b\cdot h^3}{12}$$ The highest shear stress will be at the section centroid, so you need static moment for half of the section: $$U_\tau = A_\tau\cdot z_\tau = \left(\frac{h}{2}\cdot b\right)\cdot \frac{h}{4} = \frac{b\cdot h^2}{8}$$ The width will be of course $b$, so: $$\tau = \frac{T\cdot U_\tau}{b\cdot I_y} = \frac{T\cdot \frac{b\cdot h^2}{8}}{b\cdot \frac{b\cdot h^3}{12}} = \frac{3}{2}\cdot \frac{T}{b\cdot h} = \frac{3}{2}\cdot \frac{T}{A}$$

$T$ is probably $F\cdot \frac{L}{2}$ in your formula, so the question is how will adding the point load change the critical lateral force.

Edit: Admissible stress $\tau_{adm}$ should be less than maximum $\tau_{max}$, so it is possible that in your case, 2 is safety factor. This would make sense, because in uniformly loaded cantilever beam, the maximum lateral force would be $T_{max} = F\cdot L$;

$$\tau_{adm} = \frac{\tau_{max}}{2} = \frac{\frac{3}{2}\cdot \frac{T_{max}}{A}}{2}$$