4
$\begingroup$

If you have a prestressed concrete beam or slab and are jacking a cable from both ends, does the order of operations alter the result?

For a symmetric curve, the tension diagram along the cable's span after friction losses is always shown as symmetric.

enter image description here

Is this the case regardless of whether the ends were jacked simultaneously or one after the other?

If the ends are jacked simultaneously then the symmetric friction loss makes perfect sense. However, if the cable is jacked first from the left and then from the right, I'd expect the tension profile to be asymmetric.

When jacking from the left, the cable is pulled and on its left end the cable force is equal to $P_0$, the jacking force. On the right side, the cable force is $P_0 - \Delta P$, where $\Delta P = P_0\left(1-e^{-(\mu\alpha+kL)}\right)$ is the friction losses. The left side is then anchored (disregard the anchorage slip losses for a moment). The right side is then jacked. Effectively, this jacking adds a force of $\Delta P$ to the right end, so that it too is now at $P_0$. However, wouldn't the left end also be affected with an increment equal to $\Delta Pe^{-(\mu\alpha+kL)}$, such that the diagram is asymmetric?

What about if one considers the fact that when the left side is anchored, anchorage slip losses occur? So the diagram should go: friction losses from jacking the left side, anchorage slip losses from anchoring the left side, friction losses from jacking the right side and then anchorage slip losses from anchoring the right side.

EDIT After a few honest-to-God minutes freaking out thinking I was the biggest idiot on Earth I realized that what @sanchises commented doesn't rule out my question.

The cable in my question does remain in static equilibrium, as can be seen here:

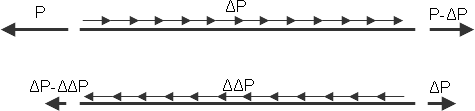
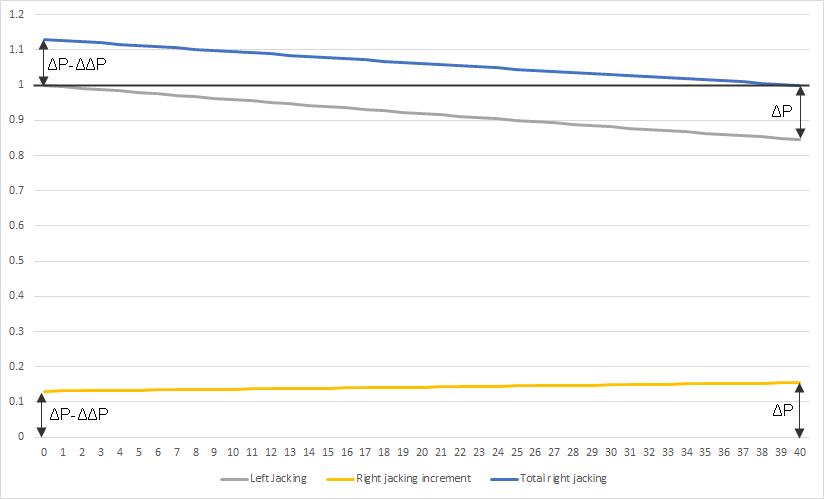
enter image description here

When jacking from the left, the cable suffers at its left extremity a force of $P$. Friction losses along the way ($\Delta P$) cause the right extremity to end up with $P - \Delta P$. The right extremity is then jacked, which effectively applies a force of $\Delta P$. Friction losses also apply here, now with a value of $\Delta\Delta P$, such that the left anchor is additionally jacked with $\Delta P - \Delta\Delta P$.

This results in the left anchor with a force of $P + \Delta P - \Delta\Delta P$ and the right anchor with only $P$. These are not, however, the only forces acting on the cable: the friction losses are what keeps it in static equilibrium. Globally, we have to the left $$P + \Delta P - \Delta\Delta P + \Delta\Delta P = P + \Delta P$$ and to the right $$\Delta P + P - \Delta P + \Delta P = P + \Delta P$$ both of which equal the total jacking force (first $P$ on the left and then $\Delta P$ on the right).

Improve this question
$\endgroup$
5
  • $\begingroup$ I have updated the question. The cable does remain in static equilibrium due to the frictional forces. $\endgroup$
    – Wasabi
    Commented Jun 11, 2015 at 17:55
  • $\begingroup$ That's right, I didn't mention that this is in concrete. I'll edit the question for future reference (I thought "post-tensioned cable" in the first sentence was enough). If you're saying I'm completely right, does that mean that indeed a prestressed (post-tensionsed) concrete cable does not display a symmetric tension diagram when not jacked simultaneously? I've never seen this mentioned anywhere before, thus my question. $\endgroup$
    – Wasabi
    Commented Jun 11, 2015 at 18:59
  • 1
    $\begingroup$ Yep! Though do take into account that some viscous effects may happen in practice (i.e., the cable may 'settle' a little); one might imagine that tapping the block in the middle temporarily reduces the static friction. $\endgroup$
    – Sanchises
    Commented Jun 11, 2015 at 19:03
  • $\begingroup$ If you compile these comments into an answer, I'll accept it. Man, I've never heard of this behavior before and always thought that, regardless of the pull order (synchronous or asynchronous), the end result would be symmetric. The more you know. $\endgroup$
    – Wasabi
    Commented Jun 11, 2015 at 19:08
  • 1
    $\begingroup$ I think you're better off answering your own question (this is actually accepted practice on StackExchange); you did the calculations better than I will in my answer, and all I did is say 'yes you are right, well done'. $\endgroup$
    – Sanchises
    Commented Jun 11, 2015 at 19:17

1 Answer 1

Reset to default
1
$\begingroup$

After the discussion with @sanchises in the comments, the answer is that, yes, the order of operations in jacking does affect the final tension diagram of the cable. A symmetric cable simultaneously jacked will have a symmetric tension diagram, while the same cable jacked from one side and then the other will not. The diagram actually ends up as a line almost parallel to the tension diagram after the jacking of the left side:

enter image description here

The cable in this example is jacked from the left side (grey line) and loses around 15% of its tension at the right anchor ($\Delta P$). It is then jacked from the right side by that same amount, adding to the tension profile according to the yellow line, which results in the blue line, with the left anchor being incremented by $0.15\cdot0.85=13\%$.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Does this answer the question in your title; Is it relevant? How does this loss compare to the initial pull of the cable, and how does it compare to the estimated losses used in codes? $\endgroup$
    – hazzey ♦
    Commented Jun 11, 2015 at 20:17
  • $\begingroup$ @hazzey, I don't understand. This demonstrates that it is quite relevant. The tension diagram is greatly modified depending on the order of jacking. If its synchronous, the diagram is symmetric. If not, the diagram is not only asymmetric, it's not even similar to the classic diagram (displayed in the OP). Also, one of the anchors ends with more than the original pull stress, which may put the stress over the limits defined in the codes, especially since the adopted pull stress usually is the limit, so any increase is relevant. The maximum increment is 25%, which I take to be very relevant. $\endgroup$
    – Wasabi
    Commented Jun 11, 2015 at 20:24
  • $\begingroup$ I can't tell if you used actual numbers to come up with your graphs or if you just did them for show. That is where my questioning comes from. I can't tell if your 15% is an estimate or a real number. $\endgroup$
    – hazzey ♦
    Commented Jun 11, 2015 at 21:52
  • $\begingroup$ @hazzey ah, I see! I did use real values and the exponential equation. It's a 40m span with $\mu = 0.28$ (which is high) and $k=0.01\mu$. The maximum value of +25% is if the friction losses are of 50% on the right anchor (over 200m using these coefficients), so that the left side gets $0.5\cdot0.5=+25\%$. $\endgroup$
    – Wasabi
    Commented Jun 11, 2015 at 22:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.