I'm trying to power and agitator (alternating circularly back and fourth) with a crank. I've seen a bunch of complicated mechanisms that do this, but I'm looking for the mechanically simplest method.
-
$\begingroup$ What mechanisms would you consider too complicated? How many revolutions should each stroke make, in what time span and how much torque would be required? $\endgroup$– fibonaticCommented Aug 24, 2016 at 0:36
-
$\begingroup$ Scale, frequency and cost constraints would help a lot. $\endgroup$– hauptmechCommented Aug 24, 2016 at 1:08
-
$\begingroup$ Are you talking about this type of motion: m.youtube.com/watch?v=QIZoMMPHBgo ? $\endgroup$– GisMofxCommented Aug 24, 2016 at 2:20
-
$\begingroup$ @GisMofx actually yes! that is the type of motion. I realize complicated is subjective, ideally less than 10 parts, and mechanical rather than electronic. I ideally one revolutions to two strokes, I have no idea of the torque and the time span is maybe one stroke per second $\endgroup$– user379468Commented Aug 24, 2016 at 2:28
2 Answers
What about a crank-rocker mechanism?
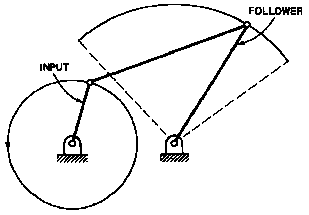
(source: udec.cl)
When I am looking for inspiration for mechanisms, I have a look in the book Mechanisms and mechanical devices, by Neil Sclater.
-
1$\begingroup$ And if you want a bigger rotation on each stroke you can just add gears. $\endgroup$ Commented Aug 24, 2016 at 12:13
-
$\begingroup$ Seems to me that basic bar mechanism design is nolonger being taught. $\endgroup$– joojaaCommented Aug 24, 2016 at 12:45
-
$\begingroup$ If you add a slider to the follower, the rocking angle becomes adjustable. $\endgroup$ Commented Dec 8, 2023 at 6:48
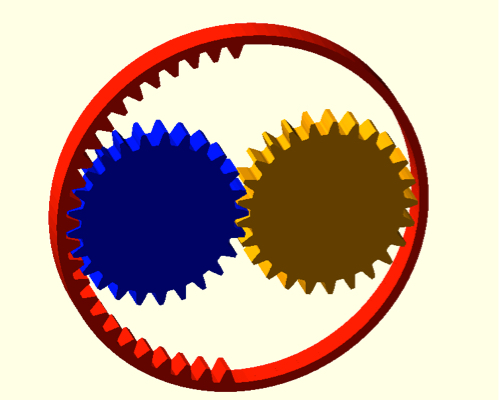
One solution is to use a system with a ring gear that only has teeth on half of its inner circumference, like conceptually shown in the following image:
There are three gears:
- Ring gear (red)
- Alternating gear (blue)
- Output gear (orange)
The alternating gear and output gears can be identical, and the sum of the numbers of teeth of the alternating and output gears should equal that to the ring gear if it had all its teeth instead of half. Otherwise the two inner gears will not properly fit inside the ring gear.
This mechanism works by rotating the ring gear, and the desired alternating motion will be obtained from the output gear. At any one time, the ring gear meshes will one of the inner gears. The inner gear that meshed will the ring gear will rotate in the same direction as the ring gear, causing the other inner gear to rotate in the opposite direction.
For example, let's say we rotate the ring gear CW. If the ring gear meshes with the output gear, the output gear will also turn CW. CW in, CW out. Now, if the ring gear meshes with the alternating gear, the alternating gear turns CW, forcing the output to be ACW. CW in, ACW out: the output shaft has alternated in direction.
The meshing of the ring gear switches between one inner gear to the other every half turn of the ring gear. It is worth noting that the output gear's speed does not change except when the meshing alternates, at which point the speed reverses.
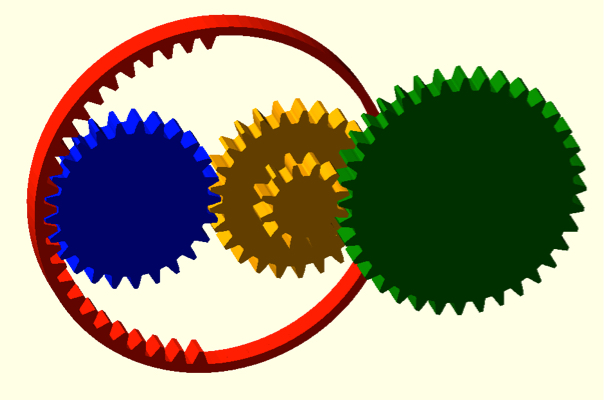
One issue with this concept as-is is that one revolution of the ring gear brings about one full turn of the output gear one way, followed by one full turn the other way: this rotation may be too much for your agitation mechanism. To resolve this, a gear reduction stage should be added to reduce the output rotation:
A gear has been fitted onto the side of the output gear (also orange to indicate its attachment), and this gear meshes with a larger gear (green). The green gear will turn slower and less far than the old orange output gear, and therefore the green gear becomes the new output. Therefore, the amount of rotation of the final output (green) that occurs before the direction alternates, in degrees, $\theta$, can be calculated using the following formula:
$$\theta=360° \cdot N_1/N_2$$
Where $N_1$ is the number of teeth on the gear attached to the side of the old orange output gear, and $N_2$ is the number of teeth on the new green output gear. In the image just above, $N_1=12$ and $N_2=36$, so the rotation angle before direction alternation is 120°. Note that if $N_1 \gt N_2$, you can get more than one output revolution before alternation (although this might not be suitable if the output is subject to high forces resisting motion).
Finally, it should be said that it is possible that, as the meshing transitions from one inner gear to the other, there may be a point where both inner gears are in mesh with the ring gear, jamming the gearset and making mesh alternation impossible. To resolve this, finer gear teeth should be used, as well as removing/filing down one or two of the end teeth on the ring gear to eliminate this dual meshing region.
ALTERNATIVE SETUP (Credit to joojaa's comment)
(@joojaa Please let me know if I've misinterpreted your comment)
An alternative configuration that does away with the ring gear would be like as shown:
Where we have the follow gears:
- Output gear (orange)
- 2x Large gears (dark red and dark blue)
- 2x Small gears (red and blue)
The small gears should have equal radii, as should the large gears. Either one of the red or blue gear sets should be driven by the crank. The large gears are in constant mesh, so this ensures that the red and blue gear sets rotate in opposite directions. The small gears have half the teeth removed and aligned such that only one of the small gears are in mesh with the output. Like before, as the mesh alternates, so does the direction of rotation of the output.
Note that the rotation of the output gear before alternation is given by:
$$\theta=180° \cdot N_s/N_o$$
Where $N_s$ and $N_o$ are the numbers of teeth on one of the small gears and the output gear respectively. Also, having selected the numbers of teeth for the small and output gears, the big gears must have the following number of teeth (so that the gears fit together):
$$N_b = N_s + N_o$$
-
$\begingroup$ There is an alernate configuration without the sunring. Instead you have 2 smaller equal radius gears with other half of gears deleted. That then mesh with the final gear. Also there is a method to do alternating rotation with a piston mechanism (due to it essentially being a fourbar like the cranker rocker in @jos answer) $\endgroup$– joojaaCommented Aug 28, 2016 at 16:01
-
$\begingroup$ I've included your gear configuration into the answer, if that's alright with you. $\endgroup$– InvoluteCommented Aug 28, 2016 at 16:57
-
1$\begingroup$ Yes, that is a good representation of what i mean. Offcourse you can use it im only glad you did. $\endgroup$– joojaaCommented Aug 28, 2016 at 17:11