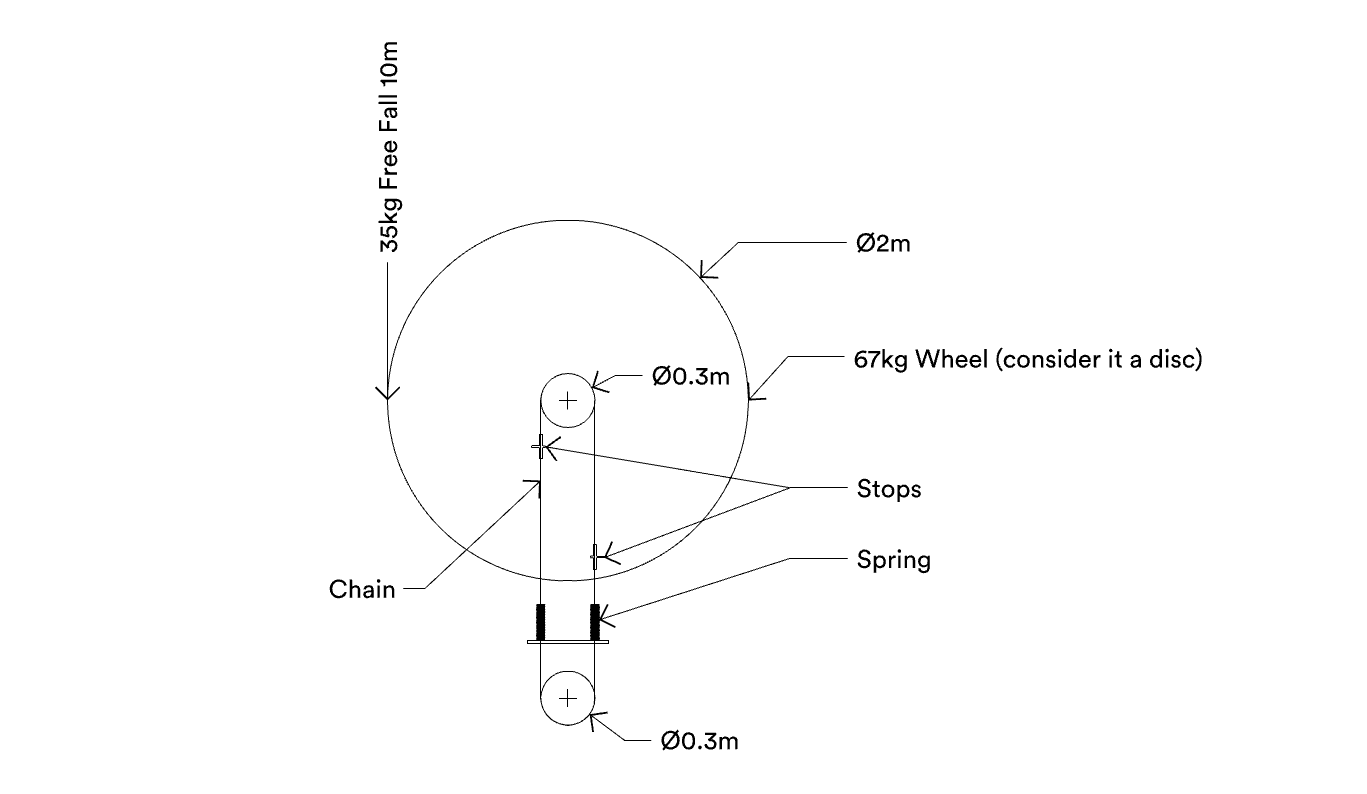
I have a wheel with a diameter of 2m and a weight of 67kg. The wheel has a radius of gyration of 0.82m. From this wheel a weight of 35kg is suspended. The weight will fall for 10m and accelerate the wheel. Connected to the shaft of the wheel is a sprocket of diameter 0.3m over which a chain with a stop runs. The stop on the chain hits a spring that will break the fall of the 35kg weight and the inertia of the wheel. The spring is 25cm long and compresses 10cm. What is the force acting on the spring?
What would be the right approach to solve this question?
My attempt:
- I calculated the the energy of the free fall of the weight
- I calculated energy of the rotating wheel
- I then summed up both linear and rotating energies and used the compression distance of the spring to get to the required force.
This resulting force seems awfully high. Any suggestions? Thanks!
Here is my attempt:
I calculated the the energy of the free fall of the weight through $E = \frac{1}{2} m v^2$ using the final velocity of the free fall (I did not know how to consider the initial inertia of the wheel though).
$g=10m/s^2$ [gravity]
$H=10 m$ [free fall height]
$t = \sqrt{\frac{2H}{g}}= 1.4s$ [time free fall]
$vmax = gt = 14.1m/s $ [velocity after t]
$m1 = 35kg$ [mass of falling object]
$E = \frac{1}{2} m v^2 = 3.5kJ$ [energy after t]I calculated energy of the rotating wheel $K = \frac{1}{2} I \omega^2$
$r1=1m$ [radius of wheel]
$w=\frac{v}{r} = 14.1/s$ [angular velocity]
$m2 = 67kg$ [mass of wheel]
$r2 = 0.82m$ [radius of gyration]
$I=m2*r2^2 = 45kgm^2$ [moment of inertia wheel]
$K=\frac{1}{2}Iw^2 = 4.5kJ$ [rotational energy wheel]I then summed up both linear and rotating energies and used the compression distance of the spring to get to the required force. $E(total) = 8.0kJ$
$d = 0.10m$ [spring length compression]
$F=\frac{E}{d} = 80kN$