0
$\begingroup$

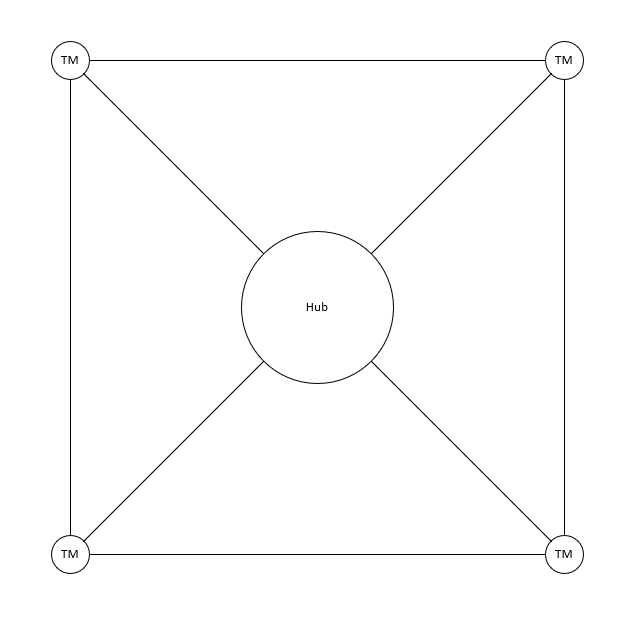
I'm studying a system in space that uses centrifugal force to keep four tip masses extended. The system is defined as follows : a central hub that has rotation freedom in all directions, four tip masses linked to the hub by cables, and cables linking all adjacent tip masses into a square. Here is a top view of the system :

enter image description here

I'm trying to find a good physical/mathematical model fit for this system. The cables may or may not be taut. Initially, the system is not rotating. For simplicity, we may keep the problem in 2D here. flexibility and mass of the cables can be neglected.

I'm trying to model this system for control, as I will try to design controllers (in 3D space) to execute maneuvers (especially, spin-up to a given rotation speed). Unfortunately, I'm at loss to model the cables... also, I'm afraid the model will be very nonlinear (when a cable reaches its max length), which will force me to go into nonlinear control, and I'm not sure I know the tools that will allow me to do it.

Of course, I could model the cables as fixed length as long as there is sufficient rotational speed to guarantee they will be kept taught, but I'm especially interested in controlling the early stages of rotation where the speed won't be sufficient to keep the cables taut.

What do you think would be the best approach ?

Improve this question
$\endgroup$
3
  • $\begingroup$ personally i'd have ditched the cables for telescoping arms or at least something stiffer. more mass but easier to balance. imbalance is a huge problem at high rpm- lots of loads on the hub that don't need to be there. $\endgroup$
    – Abel
    Commented Jan 17, 2022 at 0:29
  • $\begingroup$ Well unfortunately I’m stuck with that system @Abel $\endgroup$
    – Magix
    Commented Jan 17, 2022 at 12:19
  • $\begingroup$ if stuck with cables, always pull taught (against hub) before spinning up. add slack only when already spinning and always the same amount to opposing sides. $\endgroup$
    – Abel
    Commented Jan 18, 2022 at 2:47

1 Answer 1

Reset to default
1
$\begingroup$

If the flexibility and mass of the cables are small compared to the masses, the early stage could be just winding the cables around the hub.

Basically, we need to calculate the equation of a system of 4 whips lashing. The mass per unit length and bending moment stiffness in relation to the 4 end masses will plug into a differential equation with a large variety of trajectories. ( remember the cowboys)

Also, the link between the 4 masses needs to be a rod or a springy element like a bicycle tire spoke.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ My problem is about the link between the masses : it's cables, so it's not springy at all. Also can't model it as a rod because it could be slack so the physics could be way off $\endgroup$
    – Magix
    Commented Jan 16, 2022 at 22:08
  • $\begingroup$ ideally, when the system is past the transient early stage there is no need for the links. i think your best bet is to analyze the design in simulation software and maybe let it hang down at slow speeds, like an umbrella. i can imagine tho the entanglement of the links. $\endgroup$
    – kamran
    Commented Jan 16, 2022 at 23:18
  • $\begingroup$ it’s a space system, no gravity will be able to help @kamran $\endgroup$
    – Magix
    Commented Jan 17, 2022 at 12:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.