Calculating Anisotropic Thermal Conductivity for two Materials

Question

I've been working with SC magnet quench simulations. The conductor is made from NbTi in a Cu channel with a 5:1 ratio of Cu:NbTi. Opera3d has a quench program with an example that I began working from. In a quench scenario using a solenoid made from the conductor I described above heat propagates at different speeds throughout the bulk of the solenoid. For this case, heat conduction along the conductor (azimuthal) is fastest. Axial and radial propagation are much slower, with radial being the slowest.

Opera's documentation documentation has the following description regarding the anisotropic thermal conductivity:

Nonlinear anisotropic thermal conductivity properties are defined by three expressions based on the functions Cu_Kappa(T), Bulk_Kappa_r(T), and Bulk_Kappa_z(T).

For the azimuthal thermal conductivity of the bulk material,the conductivity of copper, Cu_Kappa(T), will be scaled by the copper factor. It is assumed that this is significantly higher than the conductivity of NbTi and dominates the thermal conduction in this direction. The bulk properties radially and axially are to be taken directly from the tables of measured values. (Note that the data used in this example are fictitious, but with similar characteristics to real materials).

So, the values used in the example are claimed to be taken from measured values.

My question is this: Can I create the radial and axial thermal conductivities from the thermal conductivities of NbTi and Cu along with their proportions in the conductor?

Here is what I've done so far in trying to find the answer:

1. Google searches. There are many articles and web pages which describe anisotropic $\kappa$. But all the information I've found assumes you already know the $\kappa$ values.
2. Hand calculations. I made the following model and tried to think of the problem in terms of thermal resistivity, which I then assumed I could treat like electrical resistances, i.e. use rules for parallel and series resistance to calculate the effective resistance.

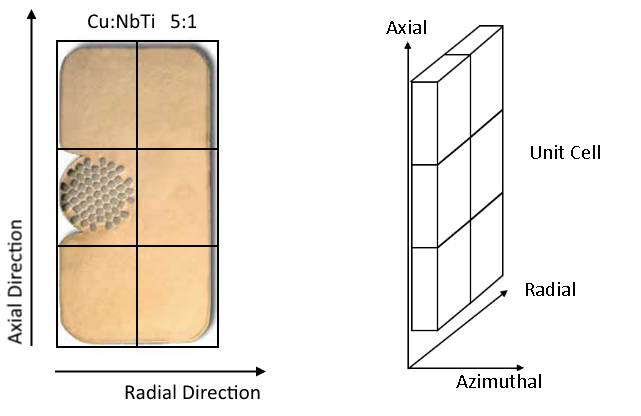
There is one more piece to this, however, and that is the insulation. Around the unit cell there is polyester insulation. It is 0.27 mm and 0.25 mm thick the edges that run in the axial and radial directions, respectively.

I am going to try accounting for this by summing the materials in their respective direction, each scaled by a packing factor.

Does this sound like a good approach? I've spent a bit of time on this already and have no idea if the answer to that approach will resemble the physical model.

Answer 1

You are not too far off with parallel and series resistors method.

The Rule of mixtures for composite design works in the same fashion - by treating each of the loads as either springs in parallel (when loaded in the direction of the fibers), or as springs in series (when loaded opposite to the direction of the fibers). (See this nice explanation if the Wikipedia explanation seems a bit difficult).

The result works for determining a wide variety of composite properties. From Wikipedia:

$$(\frac{f}{k_f} + \frac{1-f}{k_m})^{-1} < k_{composite} < fk_f + (1-f)k_m $$

can be used to determine bounds on your mixture. I'd really run the analysis with both values, and take the most conservative case.

If that doesn't work, I use MIL HDBK 17-3F for analysis. Page 223 shows the equation, which has a slightly larger lower bound by placing additional emphasis on fiber conduction. The insulation can then be added to the radials, again using the rule of mixtures (it's a pretty useful rule).

Unfortunately, I can't help you on Opera3D parameters.